The views expressed in this paper are those of the writer(s) and are not necessarily those of the ARJ Editor or Answers in Genesis.
Abstract
The highly energetic geological processes inevitably accompanying the Genesis Flood must have generated an enormous heat load without raising environmental temperatures beyond biological endurance limits. This is the second in a series of papers intended to identify and, where possible, to quantify the key sources of Flood heat. Our concern here is with the thermal history of the oceans viewed as a boundary condition on models of the Flood and post-Flood periods. Having reviewed palaeothermometry based on the oxygen-18 content of marine fossil shells (δ18O) in the first paper, we review here the development and application of alternative palaeothermometers. These include: (1) the magnesium/calcium (Mg/Ca) ratio in calcite fossil shells, notably those produced by foraminifera; (2) the strontium/calcium (Sr/Ca) ratio in scleractinian (aragonitic) corals; (3) biomolecular indicators including the alkenone unsaturation index, the iDGDT index (or TEX86) and the long diol index (LDI); (4) the clumped isotope (Δ47) index, which relates to the temperature-sensitive proportion of the joint heavy-isotope bond 13C-18O in carbonate-containing molecules in fossil materials. All these methods and the results they produce are subject to significant complications and uncertainties. However all constitute active, developing fields of research, especially the clumped isotope index, and many studies combine two or more of these methods. This provides additional information (e.g. salinity and continental ice volume) and facilitates assessment of complicating factors. In Mg/Ca palaeothermometry, the most mature of these methods, an uncertainty of ± 2.5ºC (sometimes less) can reasonably be claimed provided that complicating factors have been assessed and accounted for. The most important conclusion from this review, based on analysis of combined δ18O-Mg/Ca data and sea level records, is that deep ocean water has cooled by 12ºC since the early Eocene. This supports the conclusion in the first paper that deep ocean temperatures never exceeded 13ºC through the Cenozoic, an important constraint on Flood and post-Flood models. Further constraints on such models are provided by ocean temperatures reconstructed from studies focused on particular areas and particular points in the geological record. We have also identified several time-related puzzles and anomalies in the conventional literature which deserve investigation from a creation science perspective.
Keywords: Palaeotemperature; Mg/Ca; Sr/Ca; calcite; magnesium; strontium; foraminifera; corals; ostracods; alkenones; TEX86; diols; clumped isotopes
Introduction
The catastrophic global Flood at the time of Noah (described in Genesis 7 and 8) must have generated an enormous quantity of heat, thus raising the question of how environmental temperatures were kept within limits. This article is the second in a series aiming to identify, and where possible to quantify, the sources of Flood heat in order to provide boundary conditions and guidelines for creation scientists seeking to explain how the necessary cooling was accomplished.
The first article (Worraker 2018) considered boundary conditions relevant to modelling the earth’s thermal history including its internal temperature field, past and present ocean temperatures, surface heat flows and its inventory of heat-producing radionuclides. Of the various indicators of past ocean temperatures in current use by climate scientists, Worraker (2018) dealt only with the oxygen isotope ratio (18O/16O) in fossil shells and in ice, expressed as the 18O level or δ18O value (see Worraker 2018 for definitions). This is the longest-established and most widely used palaeotemperature indicator and has often been used for calibrating other, newer indicators. In the present article, we describe and assess the significance of several other temperature indicators including: (1) minor element ratio methods, notably Mg/Ca (magnesium/calcium) palaeothermometry, which can in principle be used to measure both the temperature and the oxygen isotope composition of seawater; (2) trace element methods, e.g. Sr/Ca and Li/Mg ratios, with particular application to corals; (3) biomolecular index methods, of which three are now commonly used in marine temperature reconstructions; (4) the carbonate clumped isotope index, which in principle can also give the temperature and, in conjunction with δ18O measurements, the seawater 18O level. These methods and their limitations are described in this order in the following subsections; Mg/Ca palaeothermometry, the most widely used of these, is given the most extensive and detailed analysis. The use of fossil assemblages as palaeoceanographic or palaeoclimate indicators is not addressed here, but deserves investigation from a creation science perspective at a later date.
Except where otherwise noted the implicit starting assumptions here are that (i) the conventional geological order of ocean floor deposits holds good within a Flood geology paradigm (Snelling et al. 1996), and (ii) the end of Flood deposits coincides approximately with the end of the Mesozoic (Austin et al. 1994). Whilst these are controversial points in Flood geology, most of our discussion does not critically depend on them. A short Nomenclature is also included.
Subsequent articles (Parts 3–6) in the series will consider particular Flood heat sources and Part 7 will summarize our findings and suggest possible directions for future investigations which might reveal a solution to Flood heat problems or clarify key related questions.
Mg/Ca Palaeothermometry
Introduction
Most planktonic and benthic foraminifera and other shell-forming marine invertebrates including ostracods (a class of small crustaceans, often known as “seed shrimps”), coccoliths and corals build their shells of calcium carbonate (CaCO3) in the form of calcite or aragonite using calcium ions [Ca2+] and carbonate ions [CO32-] from the seawater. In molar terms the magnesium concentration in seawater is typically ~5 times the calcium concentration (Mewes et al. 2014, de Nooijer et al. 2014; see also fig. 1), and during calcite shell formation magnesium ions [Mg2+], which are smaller than calcium ions, can substitute for them in the mineral lattice. In inorganic calcification this substitution is endothermic (Rosenthal and Linsley 2006, Lea 2014), such that the shell Mg/Ca ratio increases with temperature. Both experiments and thermodynamic analysis imply a sensitivity in the partition coefficient λMg [≈ (Mg/Ca)calcite/(Mg/Ca)solution] of Mg2+ ions between inorganic calcite and solution of approximately 3.0% per °C across the range 10–50°C (Barker et al. 2005; Lea, Mashiotta and Spero 1999; Oomori et al. 1987; Rosenthal, Boyle and Slowey 1997). This suggests, in line with hints from early twentieth-century chemical analyses of marine invertebrate skeletons (Clarke and Wheeler 1922), that the atomic Mg/Ca ratio in marine invertebrate shell calcite might serve as a temperature indicator. From the 1950s onwards a few studies began to confirm this idea (e.g. Blackmon and Todd 1959; Burton and Walter 1987; Chave 1954; Chilingar 1962; Katz 1973; Savin and Douglas 1973). However only from the mid-1990s onwards has the Mg/Ca ratio become widely used in palaeothermometry (e.g. Dwyer et al. 1995; Lea 2014; Lea, Mashiotta, and Spero 1999; Nürnberg, Bijma and Hemleben 1996; Rosenthal, Boyle, and Slowey 1997).
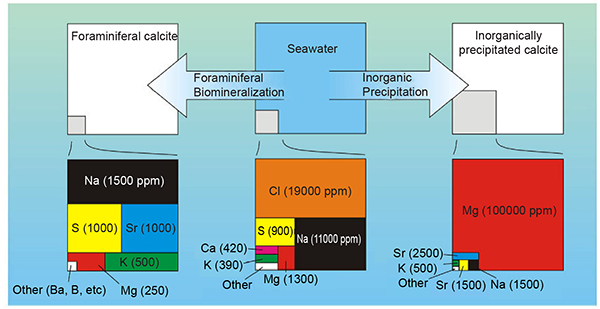
Fig. 1. Typical minor and trace element compositions of foraminiferal (left) and inorganically precipitated (right) calcite precipitated from seawater (middle). Concentrations (given as parts per million by weight) are very approximate as they differ between foraminiferal species and depend on several environmental factors. From fig. 1 in de Nooijer et al. (2014).
The shells most commonly studied in Mg/Ca thermometry are those produced by planktonic and benthic foraminifera (e.g. Barker et al. 2005; Erez 2003, Lea, Mashiotta, and Spero 1999, Katz et al. 2010; Rosenthal, Boyle and Slowey 1997); see figs. 2, 3, and 4. Others include, for example, ostracods (Dwyer et al. 1995; Elmore et al. 2012; Farmer, Cronin and Dwyer 2012), mussels (Klein, Lohmann and Thayer 1996; Vander Putten et al. 2000) and brachiopods (Butler et al. 2015). The Mg/Ca ratio in belemnite calcite, however, does not generally seem to be a useful temperature indicator (Li, McArthur and Atkinson 2012).
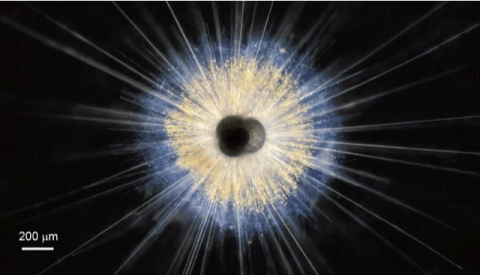
Fig. 2. This planktonic foram, a Globigerinoides ruber specimen, was collected as it floated about 3m below the surface off the coast of Puerto Rico. The central dark area is the shell surrounded by spines. The tiny yellow dots are symbiotic algae, which live in the protoplasm of the host organism. When the foram dies, the spines fall off and only the shell is preserved in the fossil record. Photo credit: Howard J. Spero/University of California, Davis. Source: https://ocean.si.edu/ocean-life/plankton/colorful-foram-globigerinoides-ruber.
Although far more magnesium is incorporated into inorganically precipitated calcite than into foraminiferal calcite (see fig. 1), the temperature sensitivity of the atomic Mg/Ca ratio measured in foraminiferal shells is generally much higher than the inorganic Mg/Ca temperature sensitivity (Lea 2014), and it differs significantly between species (Barker et al. 2005; Lea, Mashiotta, and Spero 1999; Rosenthal, Boyle, and Slowey 1997). Thus the incorporation of Mg into the shell calcite of these organisms, and of marine invertebrates generally, is biologically controlled (e.g. Erez 2003; Pérez-Huerta, Coronado, and Hegna 2018; Rosenthal, Boyle, and Slowey 1997; Sadekov, Eggins, and De Dekker 2005; Skinner and Elderfield 2005). The implications for Mg/Ca thermometry of vital (biological) effects, including environmentally induced habitat changes (e.g. seasonal or life cycle migration through the water column), are considered further below. Diagenetic changes also complicate interpretation of the Mg/Ca temperature signal in the marine sedimentary record. These effects are also discussed further below.
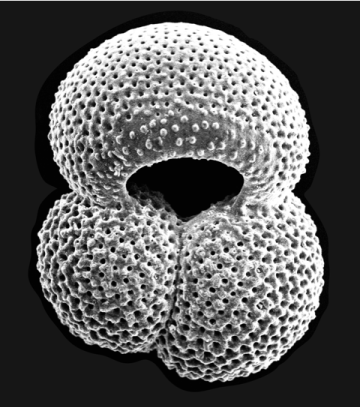
Fig. 3. A SEM (scanning electron microscope) image of a Globigerinoides ruber test from the Gulf of Mexico. Size not stated on the original, but largest chamber diameter probably ~300μm. Photo credit: Jennifer Flannery, 2017. Source: https://www.usgs.gov/media/images/sem-image-a-globigerinoides-ruber. Public domain.
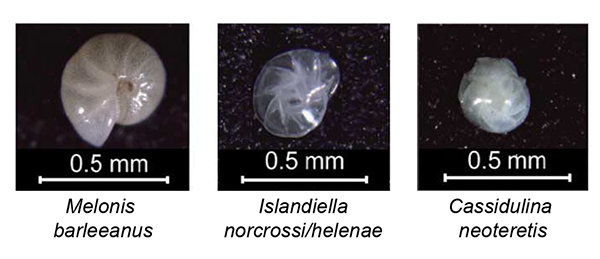
Fig. 4. Photographs of tests of three species of benthic foraminifera from the Arctic. Taken from fig. 4(a) in Kristjánsdóttir et al. (2007). M. barleeanus is pinkish brown in colour, I. norcrossi/helenae clear/translucent and C. neoteretis translucent to milky white.
An important feature of Mg/Ca thermometry is that measurement of the atomic Mg/Ca ratio in shell calcite along with the δ18O value in the same sample can potentially give both the formation temperature and the seawater 18O level (Billups and Schrag 2002; Elderfield and Ganssen 2000; Lear, Elderfield and Wilson 2000; Stott et al. 2004), and in practice δ18O values are usually measured alongside Mg/Ca ratios; this information may then allow estimates to be made of salinity and global ice volume (Barker et al. 2005; Lear, Elderfield and Wilson 2000; Rosenthal and Linsley 2006). From a uniformitarian temporal perspective the Mg/Ca ratio is regarded as a useful palaeoclimate indicator because the oceanic residence times of Ca and Mg, 106 and 107 years respectively (Barker et al. 2005; Rosenthal and Linsley 2006) are much longer than typical oceanic mixing times of ~103 years (Foster, Pogge von Strandmann, and Rae 2010). This means in the conventional chronology that the Mg/Ca ratio of seawater is practically constant over glacial/interglacial cycles. In a Flood scenario geochemical exchange and advection processes would occur on much shorter time scales, drastically reducing residence times. However no estimates of the reduced time scales are currently available.
Since ocean floors are geologically young (Müller et al. 2008), Mg/Ca palaeothermometry has mainly been employed on relatively recent fossil shells, mostly of Quaternary origin (e.g. Barker et al. 2005; Elmore et al. 2015; Nürnberg, Müller, and Schneider 2000; Sadekov et al. 2014). For example, of Mg/Ca data under the MARGO (‘Multiproxy Approach for the Reconstruction of the Glacial Ocean surface’, MARGO 2004) project, Barker et al. (2005) have undertaken SST (Sea Surface Temperature) reconstructions for the LGM (Last Glacial Maximum) using data from planktonic foraminifera. The LGM is dated at about 21 ka BP (before present) in the conventional chronology (Mix, Bard and Schneider 2001). Barker et al. (2005) conclude:
Most Mg/Ca reconstructions are concentrated in tropical regions and results consistently suggest LGM temperatures 2.0–3.5°C cooler than the Late Holocene for these regions. Results from northern latitudes are very scarce and some of them show inconsistencies with other proxies, particularly in those areas in which the temperature change associated with the last deglaciation is believed to be particularly large (> 4°C). Further data are required before any firm conclusions can be made regarding the origin of these disparities.
Mg/Ca data have also been used to trace temperature changes in the Indian Ocean through the last deglaciation (Levi et al. 2007). In another example, Mg/Ca data from the tests of the planktonic foraminifer Neogloboquadrina pachyderma have been used in conjunction with benthic foraminiferal δ18O data and faunal assemblage data to reconstruct North Atlantic hydrography and Greenland climate during the “last interglacial”, the relatively warm climate interval between the two most recent glacial maxima1 (Irvali et al. 2012, 2016).
However the use of Mg/Ca palaeothermometry is not confined to the Quaternary. By analysing benthic foraminiferal tests Lear, Elderfield and Wilson (2000) have produced a Cenozoic deep-sea Mg/Ca temperature and ice volume record covering the last 50 million years (Ma) in the conventional chronology, concluding that deep-ocean water has cooled by about 12°C since the early Eocene, and that although Antarctic ice first appeared in the earliest Oligocene, the deep water temperature was barely affected. A more sophisticated study by Cramer et al. (2011) extending back into the Cretaceous, using combined δ18O and Mg/Ca measurements on benthic foraminiferal tests and sea level data reached essentially the same conclusion. Southern Ocean cooling across the EOT (Eocene-Oligocene Transition, 35–32.5 Ma BP) has been traced using Mg/Ca data from southern hemisphere planktonic foraminifera (Bohaty, Zachos, and Delaney 2012).
Bice et al. (2006) have used combined Mg/Ca and δ18O data from planktonic foraminiferal tests to estimate upper ocean temperatures in the mid-Cretaceous, 102–82 Ma BP. Lea (2014) cites further results of Mg/Ca palaeothermometry through the Cenozoic.
Calibration
Empirical calibration of the Mg/Ca-temperature relationship is necessary because as already noted it is very different for organic precipitation (e.g. by foraminifera) compared with inorganic precipitation and it differs significantly between different organisms (Barker et al. 2005; Lea, Mashiotta, and Spero 1999; Rosenthal, Boyle, and Slowey 1997). In this section we consider several calibration exercises and their results. Complicating factors will be considered in more detail in the following section.
Calibration equations for planktonic foraminifera typically take the form
where Tc is the calcification temperature in °C and Mg/Ca is measured in units of mmol/mol. Examples from Lea, Mashiotta, and Spero (1999) are two species of cultured planktonic foraminifera, Globigerina bulloides, which does not host symbionts and for which the calibrations give A = 0.53 ± 0.17 and m = 0.102 ± 0.008 (temperature range 16–25°C), and symbiont-hosting Orbulina universa, for which A = 1.36 ± 0.24 and m = 0.085 ± 0.011 (17–27°C), corresponding to rates of increase in Mg/Ca with temperature of 10.2 ± 0.8% and 8.5 ± 1.1% per °C respectively.2 The standard error measures of these exponential data fits imply 1σ temperature uncertainties of ±1.13°C for each species.
Anand, Elderfield, and Conte (2003) produced Mg/Ca-temperature calibrations for several species of planktonic foraminifera based on paired Mg/Ca and δ18O measurements on samples taken in a 6-year sediment trap time series. Calcification temperatures were estimated from δ18O values, viz. measured δ18Oc (from shell calcite) and estimated δ18Ow for the seawater (based on salinity) using a standard palaeotemperature equation (Shackleton 1974) referenced to locally measured temperatures. Fig. 5 shows the data points and a combined calibration curve over the temperature range 14–28°C. In terms of the parameters of equation (1), this gives A = 0.38 ± 0.02 and m = 0.090 ± 0.003, which corresponds to a 1σ temperature uncertainty of ± 1.2°C. Barker et al. (2005, 825) state that:
The combined average deviation between calculated and isotopically derived temperatures for all species used in the generic calibration of Anand et al. (2003) increases from 0.9 to 1.5°C for specific and generic calibrations, respectively.
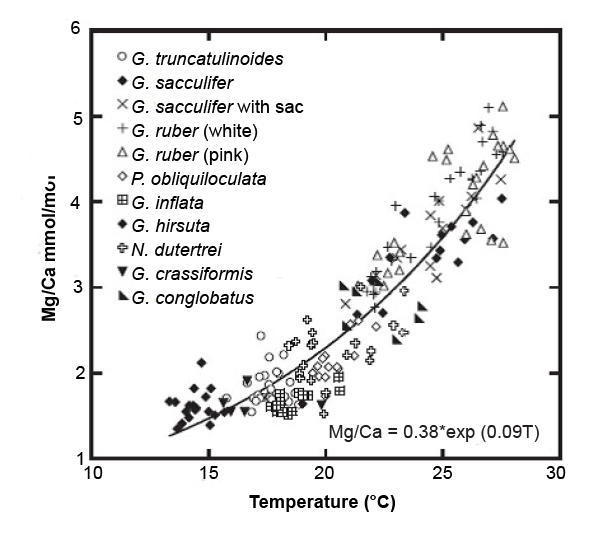
Fig. 5. The Mg/Ca versus temperature calibration obtained by Anand, Elderfield, and Conte (2003) for ten species of planktonic foraminifera from the Sargasso Sea. The samples were collected in a 6-year sediment trap time series. The habitat depth range of the organisms treated as a whole was estimated as 0–800m. Temperatures were estimated by measurement of δ18O in the test calcite. Taken from fig. 2 of Barker et al. (2005).
Thus uncertainties in reconstructed temperatures are smaller for species-specific calibrations than for combined calibrations, but to make practical use of the former in cored samples requires that the species represented are correctly identified; this is not necessarily straightforward for fossil material. The uncertainty in isotopic temperatures is not expressly quantified either by Anand, Elderfield and Conte (2003) or by Barker et al. (2005).
Subsequent temperature calibrations of Mg/Ca in planktonic foraminifera based on samples from a wider range of locations have produced similar calibration curves but with different constants. For example, Regenberg et al. (2009) distinguish between “warm water” shallow-dwelling species and thermocline species and two “cold water” deeper-dwelling species. Their results are summarised in table 1. Regenberg et al. (2009) cite an offset of ≈8°C between the “warm” and “cold” calibration curves,3 noting that this issue could cause problems in reconstructing temperatures from extinct species. The more recent investigation of δ18O and Mg/Ca values in eight living species of planktonic foraminifera by Jentzen et al. (2018) largely supports previous species-specific Mg/Ca-temperature calibrations whilst demonstrating known effects including depth migration and seasonal abundance variation.
| Species group | Depth range (m) | Temperature range (°C) | A | m | Tc uncertainty (°C) |
|---|---|---|---|---|---|
| Warm water 1 | 30–260 | 19–28 | 0.22 | 0.113 | ±0.24 (19ºC) ±0.29 (28ºC) |
| Warm water 2 | 30–260 | 19–28 | 0.29 | 0.101 | ±0.24 (19ºC) ±0.29 (28ºC) |
| Cold water | 390–630 | 8–15 | 0.84 | 0.083 | ±0.39 (8ºC) ±0.49 (15ºC) |
Von Langen et al. (2005) developed Mg/Ca-temperature calibrations for the non-spinose (spineless) planktonic foraminifera Neogloboquadrina pachyderma and N. dutertrei for use in temperate and polar regions. N. pachyderma are particularly valuable, being practically the only planktonic foraminifera living in polar waters; furthermore the coiling direction of their tests is temperature-sensitive (Bauch et al. 2003), although interpretation of this in the fossil record is not straightforward. The two types are now recognised as separate species: the left-coiling, cooler-water type is still N. pachyderma, while the right-coiling, warmer-water type is Neogloboquadrina incompta (Darling et al. 2006). Von Langen et al. (2005) used live culturing to establish the Mg/Ca-temperature relationship in neogloboquadrinid shells collected in plankton tows. Their calibration equation (cf. equation 1) gave constants A = 0.51(+0.23,−0.15), m = 0.10±0.02, temperature range 9–19.2°C. Comparing estimated temperatures with measured SSTs showed agreement within ±2°C (2σ confidence interval). However another study of Mg/Ca in N. pachyderma based on cores from the North Atlantic and Nordic Seas (Meland et al. 2006), where the present-day temperature range is 2–8°C, showed negligible sensitivity of Mg/Ca to temperature. Mg/Ca ratios in foraminiferal cells from the central Nordic Seas are ~0.4 mmol/mol higher than expected from established calibrations; sedimentation rates in this region are very low (<~5 mm/ka). Meland et al. (2006) cite several possible reasons for their results, including bioturbation, Holocene variability in old core tops, dissolution, the presence of volcanic ash and pore water chemistry.
The main calibration methods for planktonic foraminifera are culture, core top, and sediment traps. A simple outline of their characteristic advantages and disadvantages as described by Barker et al. (2005) is shown in table 2; see also Katz et al. (2010). Barker et al. (2005) note that despite the expected differences between calibrations produced by these different methods, in practice they give largely consistent results (Anand, Elderfield, and Conte 2003). Intra-specific variability also occurs as planktonic foraminifera migrate vertically throughout their life cycle, often forming calcite at increasing depth as they mature. This migration in depth (and hence in temperature) results in heterogeneity of Mg/Ca ratios within the tests of individual forams. Further intra-test variability occurs in many species due to the secretion of a secondary crust of calcite at the time of gametogenesis (germ-cell production). The Mg/Ca ratio of “whole-test” samples therefore generally represents the integration of various depth habitats and (potentially) of biological controls (Barker et al. 2005). Since many species of planktonic foraminifera live at depths exceeding 50 m, temperatures deduced from Mg/Ca ratios do not necessarily represent the SST of prime interest to palaeoclimatologists. This issue does not seem to be critical for surface and mixed layer species such as Globigerinoides ruber (pink and white) and Globigerinoides sacculifer4, but is more serious for deeper-dwelling species (Anand, Elderfield, and Conte 2003; Dekens et al. 2002). These habitat and life cycle complications are discussed in more detail below.
The use of tests produced by benthic foraminifera for Mg/Ca thermometry is inherently difficult because, except in very shallow water (e.g. as in coral reef environments—see for example Raja et al. 2005), bottom water is cold, which means that the uptake of magnesium by the foraminifera is relatively small. Furthermore during climate changes the temperature variation of the deep water mass is smaller than the SST variation, leading to only small changes in Mg/Ca in the resulting tests. Sadekov et al. (2014) estimate that analytical methods capable of distinguishing differences of 0.1–0.15 mmol/mol are necessary to successfully record glacial-interglacial deep water temperature changes of order 1°C. This small range in Mg/Ca is close to the typical analytical precision reported in the literature (e.g. 1σ ≈ 0.08–0.02 mmol/mol), implying poor signal-to-noise ratio. Complicating effects, e.g. the suppression of Mg/Ca at high carbonate ion concentrations (Bryan and Marchitto 2008), are discussed below.
Rosenthal, Boyle, and Slowey (1997) and Lear, Rosenthal, and Slowey (2002) develop Mg/Ca-temperature calibrations for benthic foraminifera, the latter using samples from a wide range of sites. For Cibicidoides pachyderma from the Little Bahama Bank (LBB), Rosenthal, Boyle, and Slowey (1997) obtain a calibration equation of the same form as (1) with A = 1.36 and m = 0.044 for the temperature range 4.5–18°C; they cite a 2σ forecasting uncertainty of ±0.6°C at 12°C, increasing to ±0.85°C at 18°C. The result obtained by Lear, Rosenthal, and Slowey (2002) for three common Cibicidoides species is similar but with different constants, viz. A = 0.867±0.049 and m = 0.109±0.007, which they estimate gives a 2σ uncertainty in bottom water temperature of ±1°C.
| Method | Advantage(s) | Disadvantage(s) |
|---|---|---|
| Culture | – Known, controlled temperature0 | – Laboratory conditions unrepresentative; – Calibration affected by changing biological controls 0 |
| Core top | – Calibrations based on material forming part of sediment record0 | – Temperature must be estimated; – Susceptible to post-depositional alteration, e.g. by calcite dissolution (which reduces Mg/Ca) 0 |
| Sediment trap | – Growth season known, hence range of temperatures used in calibration well constrained; – Trap material best represents material about to enter sediment record0 |
– Temperature must be estimated0 |
These Cibicidoides calibrations rely solely on LBB samples. Using samples from the Florida Straits, Marchitto et al. (2007) and Curry and Marchitto (2008) found significantly lower Mg/Ca sensitivity to temperature over the range 5.8–18.6°C. Their best data fits were linear rather than exponential, giving statistically indistinguishable sensitivities of 0.12 and 0.13 mmol/mol per °C respectively. In these cases the reduced sensitivity is most noticeable at temperatures above ~12°C; the authors attribute the difference as possibly due to dissolution effects. The standard error (1σ) estimates for temperature reconstructions from these Mg/Ca calibrations are ±2.4°C (Marchitto et al. 2007) and ±2.6°C (Curry and Marchitto 2008). Bryan and Marchitto (2008) cite the results of numerous Mg/Ca-temperature calibrations for several genera of benthic foraminifera taken both from the literature and from their own study, in total covering the temperature range from –2 to 19.0°C. The temperature standard error (1σ) estimates in the newly investigated species were ±1.6°C for Uvigerina peregrina, ±2.5°C for Planulina ariminensis and P. foveolata combined and ±4.5°C for Hoeglundina elegans.
Kristjánsdóttir et al. (2007) developed cold water (0–7°C) Mg/Ca-temperature calibrations for three Arctic species of benthic foraminifera in order to reconstruct the north Iceland shelf temperature over the last 4,000 years. Using δ18O-based calcification temperatures for reference they derived calibrations with 2σ uncertainty margins of ±0.63°C (Islandiella norcrossi/helenae), ±1.10°C (Melonis barleeanus) and ±0.62°C (Cassidulina neoteretis).
Barrientos et al. (2018) investigated the Mg/Ca-temperature relationship in six living Arctic species of benthic foraminifera, three of them epifaunal (living on the sea floor) and three infaunal (living within the sea floor sediment); the overall range of bottom water temperatures was very small, approximately –1.9 to +0.9°C; for individual species the range was even smaller. The three infaunal hyaline species (i.e. with clear or translucent shells) showed the clearest Mg/Ca-temperature relationship and were deemed potentially useful for Mg/Ca palaeothermometry in cold regions; these are Elphidium clavatum, Nonionella labradorica, and Cassidulina neoteretis. The calculated sensitivities of two epifaunal species, Cibicidoides wuellerstorfi and Oridorsalis tener, although roughly consistent with previous findings, were based on very limited datasets. However the high-Mg porcelaneous epifaunal species Quinqueloculina arctica showed a strong Mg/Ca dependence on carbonate ion concentration and were deemed by Barrientos et al. (2018) unsuitable for use in Mg/Ca thermometry.
The superfamily Miliolacea of deep-water, high-Mg benthic foraminifera exhibits a practically unique Mg/Ca sensitivity to temperature; these organisms contain on average about 10 times as much magnesium as the benthic species used most often in palaeothermometry. Sadekov et al. (2014) found that in Pyrgo sp. (comprising P. sarsi and P. murrhina) living at various depths in the Timor Sea close to the north Australian coast, the correlation of test Mg/Ca with water temperature and carbonate ion concentration (temperature range –1 to +8°C) followed a linear relationship of the form
where T is the bottom water temperature, Δ[CO32-] (≡ [CO32-]in situ–[CO32-]saturation) is the carbonate ion concentration referenced to the saturation state, A = 2.53±0.22, B = 0.129±0.023 and C = 4.63±0.53; B has units of mmol/mol per μmol/kg. The temperature sensitivity in equation (2) closely resembles the inorganic temperature sensitivity, implying that the magnesium content of Pyrgo sp. calcite is largely inorganically controlled. Since the magnesium uptake of Pyrgo sp. is so high (~18 mmol/mol at 4°C), Sadekov et al. (2014) note that the analytical precision of Mg/Ca measurements (2σ uncertainty margin 0.08 mmol/mol) is equivalent to an uncertainty in temperature estimates of only ±0.03°C; the approximate equivalent uncertainties for Cibicidoides sp. and Uvigerina sp. are ±0.5–1°C and ±1°C respectively. However the temperatures derived from the extremes of the Pyrgo sp. calibration curves (with the [CO32-] dependency removed) are –0.7±0.27°C when Mg/Ca = 3 and 8.1±0.92°C when Mg/Ca = 25 (1σ uncertainty margins).
In these studies of benthic foraminifera the Mg/Ca calibrations were based on samples taken from cores; sediment traps are not relevant for benthic organisms. However Toyofuku et al. (2000) investigated Mg/Ca in high-Mg benthic foraminifera both in cultures and in specimens taken from natural environments in order to evaluate the effect of environmental factors on Mg/Ca thermometry; they concluded that Mg/Ca values in the foraminiferal tests clearly reflect growth temperatures in the range 10–25°C.
The ostracod Mg/Ca-temperature calibration used by Dwyer et al. (1995) based on core top samples from the North Atlantic (temperature range 2–14°C) was linear, the bottom water temperature being given by T = 0.854*(Mg/Ca)–5.75; they estimate a 2σ uncertainty in reconstructed temperature of ±1°C. Other calibrations are given by Farmer, Cronin, and Dwyer (2012) and by Elmore et al. (2012).
Klein, Lohmann, and Thayer (1996) grew mussels (Mytilus trossulus, also known as M. edulis) in their natural habitat while measuring the water temperature and analysing its chemistry and isotopic (18O/16O) composition. They established a linear Mg/Ca-temperature correlation for the mussel shell material (temperature range 5–25°C) with statistics implying an apparent 1σ uncertainty of approximately ±1.5°C. Vander Putten et al. (2000) conducted a similar investigation of M. edulis mussels grown in cages in the sea close to the Netherlands coast (approximate temperature range 8–20°C). They found a seasonal, temperature-related variation in the shell Mg/Ca ratio. However this covariation was abruptly interrupted after the spring phytoplankton bloom, implying that control of magnesium incorporation into the mussel shells was now mainly biologically controlled; hence Vander Putten et al. (2000) could not recommend the Mg/Ca ratio in these mussels as a high-resolution temperature proxy.
Complications
Sample cleaning methods have sometimes been implicated in partial dissolution leading to skewed Mg/Ca ratios (Barker, Greaves, and Elderfield 2003; Marr et al. 2011), and since methods differ between laboratories, comparability of results is often prejudiced. The basic need here is for inter-laboratory standardization.
Beyond this, complicating factors in Mg/Ca thermometry are often difficult to separate because they relate to the effect of environmental conditions on the calcifying organisms during their lifetime and on their shells after death. Furthermore individual environmental factors are often interrelated, for example salinity and seawater magnesium concentration, or pH and carbonate ion concentration. However for practical purposes we may consider them under three main headings: (1) diagenetic effects (Edgar et al. 2015; Kontakiotis et al. 2016); (2) environmental effects, i.e. seawater chemistry (including Mg/Ca ratio, salinity, pH and carbonate ion concentration); (3) vital effects, which arise from the way the calcifying organisms respond to environmental changes and control their own internal chemical environment.
(1) Diagenetic (post-depositional) effects. These include recrystallization and calcite encrustation of shells, which can add high-Mg calcite, and post-depositional dissolution. Of these processes, partial dissolution is widely regarded as the most serious problem in Mg/Ca-palaeothermometry. It generally occurs when the bottom or pore water concentration of CO32- ions is below saturation, i.e. Δ[CO32-]<0. Since Mg-rich calcite is preferentially removed by dissolution, the Mg/Ca ratio of the remaining calcite is reduced (Barker et al. 2005; Brown and Elderfield 1996; Dekens et al. 2002; Rosenthal et al. 2000; Rosenthal and Lohmann 2002; Savin and Douglas 1973), thereby depressing reconstructed temperatures.
The saturation level of CO32- ions is primarily determined by temperature and (especially) pressure: calcite becomes more soluble with depth as the temperature falls and the pressure increases, the latter at a rate of approximately 100 bar per km. While near-surface waters are generally supersaturated with respect to calcium carbonate, at a depth known as the lysocline, the dissolution rate increases dramatically, and below this, at the carbonate compensation depth (CCD), the dissolution rate equals the calcite supply rate, and no more calcite is formed (Berger, Bonneau, and Parker 1982). Roughly speaking the CCD lies about 4.5 km down in the Pacific, 6 km down in the Atlantic, the difference arising because the Pacific has a lower pH and is cooler than the Atlantic, such that its lysocline and CCD are higher in the water column (Geosciences LibreTexts 2019). Within a particular ocean basin depth can thus serve as a proxy for Δ[CO32-] as a calcite dissolution parameter. However sometimes calcite can dissolve well above the lysocline, for example as the degradation of sedimentary organic material releases CO2 into sediment pore waters, thus acidifying the local environment (Brown and Elderfield 1996; Dekens et al. 2002; Regenberg et al. 2006; Tachikawa et al. 2008).
Several investigators have attempted to estimate the uncertainties introduced by partial dissolution of planktonic foraminiferal tests and if possible to correct for them (Dekens et al. 2002; Khider et al. 2015; Regenberg et al. 2006; Rosenthal et al. 2000; Rosenthal and Lohmann 2002; Tachikawa et al. 2008). Huang et al. (2008) found a seasonally dependent Δ[CO32-] dissolution effect. The effect of partial dissolution on Mg/Ca in benthic foraminiferal tests seems to have received little attention.
The high-Mg crust found on planktonic foraminiferal tests from the very high-salinity Red Sea (Hoogakker et al. 2009) and from the Mediterranean Sea (Boussetta et al. 2011; Kontakiotis et al. 2011, Sabbatini et al. 2011; van Raden et al. 2011) seems to be diagenetic in origin, arising from direct inorganic precipitation of Mg-rich calcite; in the Mediterranean the degree of overgrowth increases from northwest to southeast (Kontakiotis et al. 2016). Such crust is not attributed primarily to high salinity, nor necessarily to the high carbonate saturation state of interstitial water, but possibly to a combination. Sabbatini et al. (2011) find a salinity effect on Mg/Ca in material from the western Mediterranean, and note evidence of recrystallization in some tests (see also Tribble and MacKenzie 1998). Fig. 6 shows an example of an obvious diagenetic overgrowth from an open-ocean site.
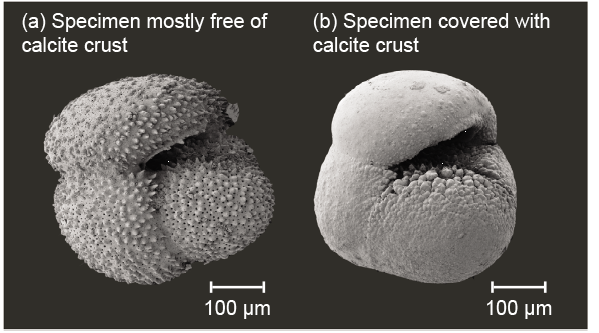
Fig. 6. SEM images of characteristic Globorotalia inflata tests from a South Atlantic core, classified as (a) nonencrusted and (b) encrusted specimens. Imaging performed at the Department of Geosciences, University of Bremen. Taken from fig. 2 in Groeneveld and Chiessi (2011).
(2) Environmental effects. The most important environmental variables seem to be magnesium concentration in the seawater, salinity, carbonate ion concentration and pH. Carbonate ion concentration and pH are linked via the balance of chemical species representing DIC (dissolved inorganic carbon), viz. aqueous CO2, bicarbonate (HCO3-) ions and carbonate (CO32-) ions. Increased levels of dissolved CO2 (which produces carbonic acid) tend to reduce both pH and carbonate ion concentration (Langdon et al. 2000, Russell et al. 2004).
Seawater magnesium concentration. Segev and Erez (2006) investigated the effect of ambient Mg/Ca (Mg/Casw) on both calcification and shell composition in two species of cultured benthic foraminifera. They found a positive nonlinear correlation between shell Mg/Ca and Mg/Casw (Mg/Casw range 0.53–10.1), equivalent to a magnesium distribution coefficient DMg which decreases with increasing Mg/Casw. The calcification rate in their experiments was sensitive to Mg/Casw rather than to the absolute Mg2+ or Ca2+ concentrations. Similar results were obtained by Raitzsch et al. (2010). For pre-Quaternary times (i.e. over time scales longer than the oceanic residence times of calcium and magnesium ions) Evans and Müller (2012) claim that previous calibrations of foraminiferal Mg/Ca against both temperature and Mg/Casw were based on the incorrect assumption that DMg was invariant with changes in Mg/Casw; they propose an alternative formulation.
Nehrke et al. (2013) investigated the transport of calcium ions in the benthic species Ammonia aomoriensis and developed a model to explain the full range of Mg/Ca found in benthic foraminifera. They deduced that the major transport mechanism for calcium ions was trans-membrane transport, with a small PT (passive transport) contribution; PT occurs through endocytosis (or vacuolization), an established phenomenon in foraminifera, in which a drop of seawater incorporated into a vacuole is then transported to the calcification site. Nehrke et al. (2013) conclude that this form of PT is key in determining the trace element composition of the test, claiming that their model predicts the effect of changing Mg/Casw. Mewes et al. (2014) undertook a culture study in two benthic foraminifera of calcification as a function of seawater Mg2+ concentration and found that shell Mg/Ca was positively correlated with Mg/Casw; again, DMg decreased with increasing Mg/Casw. Mewes et al. (2014) claim that their form of shell/seawater Mg/Ca relationship is fairly robust and generic.
In terms of changing oceanic Mg2+ concentrations over time, Fantle and DePaolo (2006) and Medina-Elizalde, Lea, and Fantle (2008) have suggested that seawater magnesium and calcium concentrations have changed significantly over the last few Ma, Mg/Ca oscillating on top of a rising Mg/Ca trend. Medina-Elizalde, Lea, and Fantle (2008) suggest that allowance for this effect would mean that early Pliocene SSTs in the tropical Pacific were significantly higher than derived from models assuming constant ocean Mg/Ca. Changes in ocean magnesium inventory can potentially be traced through its isotopic composition, manifested as the δ26Mg index,5 since this is unaffected by temperature or pH but is sensitive to continental weathering (representing a magnesium source) and to dolomite formation (a magnesium sink); see Katz et al. (2010); Pogge von Strandmann (2008).
Salinity. In culture studies of the planktonic foraminifera Globigerinoides sacculifer, Nürnberg, Bijma, and Hemleben (1996) found that temperature was the key factor controlling magnesium uptake when salinity was kept in a narrow range (33–36‰). However at constant temperature (26.5°C) and with widely varying salinity (22–45‰), test Mg/Ca was controlled by salinity, a 10% increase in S (salinity) producing a 110% increase in Mg/Ca. Lea, Mashiotta, and Spero (1999) found in culture studies of Orbulina universa that the Mg/Ca ratio in their tests increased by 4±3% per psu (1 psu ≈ 1‰—see Nomenclature) salinity increase. Sadekov et al. (2009) studied the Mg/Ca-temperature relationship in the final chambers produced by three species of planktonic foraminifera in core tops from southern equatorial regions. They used salinity (taken from previous publications—range 33–38‰) as a secondary input parameter in their calibration equations, though without discussing how it might influence magnesium uptake.
Kisakürek et al. (2008) investigated the Mg/Ca and Sr/Ca ratios of cultured Globigerinoides ruber (white) from the Gulf of Eilat as functions of temperature (18–30°C), salinity (32–44 psu) and pH (7.9–8.4); since these planktonic foraminifera generally live within 50 m of the sea surface, they are a favourite species for use in SST palaeothermometry. At ambient pH levels (8.1–8.3) they found no pH dependence, but obtained the following calibration equation:
Here T is temperature in °C and S salinity in psu, A = 0.08±0.02, B = 0.06±0.02, C = –2.8±1.0; quoted uncertainties are 2σ values. Kisakürek et al. (2008) suggest that sea surface salinity changes between glacial/interglacial periods can give rise to overestimates of Mg/Ca-based SST changes by roughly 0.7°C/psu. In a culture study of Globigerinoides sacculifer collected from surface water near the southwest coast of Puerto Rico, Dueñas-Bohórquez et al. (2009) found that a salinity increase of 4 psu was equivalent to a 1°C bias on Mg/Ca-based temperatures, noting that this needs to be taken into account when using Mg/Ca-based temperatures in conjunction with foraminiferal δ18O to deduce salinity.
In a study of cored samples of four species of planktonic foraminifera from the Mediterranean Sea, Ferguson et al. (2008) found salinity-related Mg/Ca variations in the range 15–59% per psu (i.e. much larger than in cultured foraminifera); they concluded that this was not due to diagenetic high-Mg overgrowths. Groeneveld et al. (2008) studied cored samples of G. sacculifer from Miocene/Pliocene sediment in the Caribbean, combining both Mg/Ca and δ18O measurements to deduce sea surface salinity changes during the shoaling of the Isthmus of Panama around 4.5 Ma BP (Bell et al. 2015). Groeneveld et al. (2008) did not find diagenetic overgrowths with especially high Mg/Ca, but concluded that there was a significant salinity variation recorded in their Mg/Ca data, and proposed a correction to Mg/Ca-determined SST values for the period 4.5–3.9 Ma using the Mg/Ca-S sensitivity found by Nürnberg, Bijma, and Hemleben (1996). However part of the underlying reasoning involves the assumption of orbitally forced climate changes; creation scientists generally reject this assumption (Hebert 2016a, b, c).
Sabbatini et al. (2011), working with cored G. ruber tests from the western Mediterranean Sea, found very little Mg/Ca-temperature correlation, but rather a strong sensitivity of Mg/Ca to salinity, a +1 psu change in S producing an apparent 1.7°C rise in T estimated from the Anand, Elderfield, and Conte (2003) calibration. This is similar to the sensitivity (+1.6°C Mg/Ca temperature bias for +1 psu change in S) found by Mathien-Blard and Bassinot (2009) in G. ruber tests from open-ocean sites, though in the latter case this is treated as a method of correcting Mg/Ca SST estimates using for reference isotopic (δ18O) temperature reconstructions based on the Shackleton (1974) calibration.
Hönisch et al. (2013) undertook a culture study of the effect of salinity variation on Mg/Ca in three planktonic foraminifera (Orbulina universa, G. sacculifer and G. ruber) and combined their results with previously published culture studies. They deduced respective Mg/Ca sensitivities to salinity of 4.4±2.3%, 4.7±1.2% and 3.3±1.7% per psu (2σ/95% confidence intervals), noting that these are much smaller than sensitivities derived from Atlantic coretop studies, cited as 27±4% for G. ruber. Hönisch et al. (2013) suggest that this discrepancy arises from the dissolution correction often applied to Mg/Ca data, which can lead to significant overestimation of temperatures, and claim that combining evidence of seasonality and latitude-specific habitat depth preferences with corresponding variations in environmental conditions enables reconciliation of culture calibrations and core top observations.
Khider et al. (2015) applied a Bayesian calibration model to G. ruber Mg/Ca data from 186 globally distributed core tops in terms of three parameters, viz. temperature, salinity and dissolution, the latter expressed as deep-water Δ[CO32-]. They report resulting sensitivities of 8.7±0.9%/°C, 3.9±1.2%/psu and 3.3±1.3%/μmol.kg-1 (for Δ[CO32-] < 21 μmol.kg-1) respectively (2σ uncertainty margins). These results are in good general agreement with previous studies. Khider et al. (2015) also applied Bayesian modelling to a published sedimentary record from the western tropical Pacific (Stott, Timmermann, and Thunell 2007), concluding that systematic changes in sea surface salinity and deep-water Δ[CO32-] were the main sources of bias in Mg/Ca palaeothermometry.
Gray et al. (2018) presented Mg/Ca-SST calibrations for 440 G. ruber (white) sediment trap/plankton tow samples from the Atlantic, Pacific, and Indian Oceans; 130 of these were newly collected. They investigated two calibration models, assuming (1) pH, and (2) [CO32-], to be the controlling carbonate system parameter. The resulting Mg/Ca sensitivities (2σ uncertainty margins) in model (1) are 6.0±0.8%/°C for temperature, 3.3±2.2%/psu for salinity and –8.3±7.7%/0.1 pH unit, and in model (2), 6.7±0.8%/°C for temperature, 5.0±3.0%/psu for salinity and –0.24±0.11%/μmol.kg-1 for [CO32-]. Gray et al. (2018) note that in both models the temperature sensitivity is smaller than in the commonly applied calibrations of Dekens et al. (2002) and Anand, Elderfield, and Conte (2003), commenting that estimates of salinity and carbonate chemistry (expressed either in terms of pH or [CO32-]) should ideally be combined with Mg/Ca measurements to give reliable SST reconstructions.
In a core-top study of four species of planktonic foraminifera from the Indonesian Throughflow region (the region between Borneo, Celebes and the eastward-extending Java island chain), Zhang et al. (2019) did not find a significant salinity effect on Mg/Ca. However they suggested that Mg/Ca-based temperature differences between two of the species, G. ruber (sensu stricto6) and G. sacculifer, could be used to reconstruct the depth of the mixed layer in this region.
Kontakiotis et al. (2016) review the field of foraminiferal Mg/Ca thermometry, focusing on the distinction between field-based and culture-based studies. Given some of the apparent discrepancies (see, for example, comments above on the work of Hönisch et al. 2013), they emphasize the need for combining them to better understand how foraminiferal Mg/Ca depends on temperature and the effect of complicating factors, notably salinity.
Carbonate ion concentration. As already noted, carbonate ion concentration can, if high, lead to post-depositional calcite encrustation of shells or, if low or negative, lead to calcite dissolution—in both cases compromising the use of Mg/Ca as a palaeothermometer. However it can also influence the Mg/Ca ratio in calcite produced by living organisms. Katz et al. (2010) note from various studies that Mg/Ca in benthic foraminifera at temperatures below 3°C is more sensitive to carbonate ion saturation than to temperature at low or undersaturated Δ[CO32-] levels, citing a sensitivity of 0.0086 mmol/mol per μmol/kg. Calibration of Mg/Ca in the deep-water benthic foraminiferal family Miliolacea by Sadekov et al. (2014) shows how it depends on both temperature and carbonate ion saturation [see equation (2)].
Bryan and Marchitto (2008), who found that the Mg/Ca-temperature relationship in four species of benthic foraminifera flattens out at higher temperatures, suggest (but without explanation) a possible Mg/Ca-suppression effect at high Δ[CO32-]. Lear, Mawbey, and Rosenthal (2010) used published sensitivities of Mg/Ca and Li/Ca ratios to both temperature and carbonate saturation state in benthic foraminiferal calcite to deduce bottom water temperatures and saturation states through the Middle Miocene climate transition (16.6–11.6 Ma BP in the conventional chronology). Dawber and Tripati (2012) investigated the correlation between four element/Ca ratios (B/Ca, Li/Ca, Mg/Ca and Sr/Ca) and bottom water Δ[CO32-] in the benthic foram Oridorsalis umbonatus and inferred a mechanistic cause for this correlation (i.e. it didn’t simply reflect the covariation of Δ[CO32-] with other hydrographic variables that influence element/Ca ratios). Weldeab, Arce, and Kasten (2016) found that the infaunal genus Globobulimina (more than one species) produced calcite Mg/Ca sensitive both to temperature and to pore water Δ[CO32-], the latter being correlated with bottom water Δ[CO32-]. They claimed that after correcting for Δ[CO32-]pore water, their calibrations were both sensitive and robust.
pH. As already noted, pH and carbonate ion concentration are closely linked, and the effect of seawater carbonate chemistry on calcification and on the uptake of magnesium by calcifying foraminifera can often be expressed through either parameter (Gray et al. 2018).
As for the apparent effect of pH on shell calcite Mg/Ca, Lea, Mashiotta, and Spero (1999) report sensitivities in culture experiments of –6±2% for G. bulloides and –6±3% for O. universa per 0.1 unit increase in pH. Russell et al. (2004) report from further culture experiments that below ambient pH (pH < 8.2), Mg/Ca decreased by 16±6% for G. bulloides and by 7±5% for O. universa per 0.1 unit increase in pH. Kisakürek et al. (2008), in experiments with cultured G. ruber, tested the sensitivity of shell calcite Mg/Ca to pH over the pH range 7.9–8.4. For the ambient pH range 8.1–8.3 the sensitivity was negligible, but reducing pH from 8.1 to 7.9 produced an increase of 80±10% (2σ) in Mg/Ca, and increasing pH from 8.3 to 8.4 produced a reduction in Mg/Ca of 35±11% (2σ). Evans et al. (2016), in another culture study of G. ruber especially concerned with the effect of seawater carbonate chemistry, argue that pH rather than CO32- is the key secondary control on shell calcite Mg/Ca. They present new Mg/Ca-pH calibrations showing how to correct for differential modern and ancient pH. As already noted, Gray et al. (2018) demonstrate how G. ruber Mg/Ca data can be calibrated by either of two models, both including temperature and salinity as independent variables, one including pH and the second CO32- as the third independent variable; they express a slight preference for pH to represent the effect of carbonate chemistry. In support of this, recent studies have suggested the use of boron isotope measurements (δ11B) as a seawater pH indicator (Henehan et al. 2016; Katz et al. 2010; Sosdian et al. 2018).
(3) Vital effects. These relate mainly to foraminifera, especially planktonic foraminifera. Included are interindividual variability; intra-test magnesium distribution; seasonal changes; variation in habitat depth with age (for planktonic organisms); shell size; ontogeny (variation in calcification related to maturity, especially during gametogenesis) and diurnal variation in calcification due to light level changes (e.g. Köhler-Rink and Kühl 2005), notably in the presence of photosynthetic algal symbionts; this last effect is considered below in the context of intratest magnesium banding.
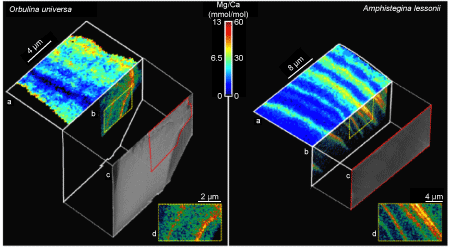
Fig. 7. The distribution of Mg in two species of foraminifera, Orbulina universa and Amphistegina lessonii, following fig. 1 in Branson et al. (2013). Electron microprobe maps of the shell samples show patterns of Mg/Ca banding (a) in agreement with Scanning Transmission X-ray Microscope (STXM) images of Mg-specific X-ray absorption (b, arbitrary units) in the thin-section samples (c). This confirms that the STXM Mg signal is real, not an artefact of sample density or thickness variations. Magnified areas of the STXM images denoted by dashed yellow boxes show the length scale of Mg heterogeneity to be in the order of 100–300nm. The colour scale bar denotes Mg/Ca in the electron microprobe maps [(a) and (d)].
Interindividual variability. Individual forams within a single population can produce differing test Mg/Ca ratios. This was demonstrated in a culture study of G. sacculifer, from which Dueñas-Bohórquez et al. (2011) deduced a contribution from interindividual variability (1σ) of 2.5±0.5°C to the total apparent temperature variance, very similar to the interindividual variability found in a core top study of G. ruber (Sadekov et al. 2008). Dueñas-Bohórquez et al. (2011) suggest that interindividual variability may be similar for many planktonic foraminiferal species. Given a sufficient sample size (Sadekov et al. 2008 suggest ~20 for G. ruber) from a single-species population, this variability will have no significant effect on the estimated mean Mg/Ca value.
Intra-test magnesium distribution. Microscopic examination of foraminiferal shells shows that the distribution of magnesium at the sub-micron scale is highly heterogeneous (Branson et al. 2013; Eggins, Sadekov and De Dekker 2004; Fehrenbacher et al. 2017; Geerken et al. 2018, 2019; Jonkers et al. 2016; Kunioka et al. 2006; Spero et al. 2015). It follows a banded structure (see fig. 7) in which high magnesium concentrations are associated with high concentrations of organic molecules, sodium, sulphur and other trace elements. However X-ray absorption spectroscopy shows that magnesium is uniformly substituted for calcium in an octahedral coordination within the calcite crystal lattice (Branson et al. 2013). These observations indicate the action of continuous calcification and magnesium uptake mechanisms in foraminifera, thereby implying an inorganic thermodynamic connection between seawater temperature and Mg/Ca in their shells. This fundamentally supports the use of their Mg/Ca ratio as a temperature indicator. Cusack et al. (2008) investigated the magnesium distribution in brachiopods, concluding that it was always incorporated in the calcite mineral lattice, not in the organic shell material; however they only found support for using Mg/Ca as a temperature indicator in rhynchonelliform brachiopod species, not in the craniid species Novocrania anomala. Branson et al. (2018) found that magnesium in the high-Mg marine ostracod Krithe is hosted in organic shell materials, not primarily in the calcite lattice; their observations suggest a more complex biologically controlled biomineralization mechanism. Unless the relevant processes and their controls are better understood, these observations cast doubt on Mg/Ca thermometry based on craniid brachiopod and Krithe ostracod shells.
High resolution microanalysis of Orbulina universa tests from the Indian Ocean (Eggins, Sadekov and De Dekker 2004) suggests that magnesium banding in foraminifera arises, not from temperature variations, but from the diurnal variation of [CO32-] concentration and hence of pH within the foraminiferal microenvironment due to the day-night photosynthesis-respiration cycle of algal symbionts: thin high-Mg bands alternate with broader, low-Mg bands. In this model the high-Mg calcite would be deposited at night and the low-Mg calcite in the daytime. Later experiments on the same species (Spero et al. 2015) confirm these observations and show that Mg-banding is an inherent feature of biomineralization in O. universa; they also support the fundamental soundness of Mg/Ca palaeothermometry. Banding in another planktonic foraminifer, Neogloboquadrina dutertrei, which has more complex test morphology, follows a similar diurnal pattern (Fehrenbacher et al. 2017) in which high-Mg calcite is also preferentially deposited at night.
This diurnally paced mechanism does not appear to be universally applicable. Erez (2003) argues that calcification and photosynthesis are not necessarily coupled in symbiont-hosting foraminifera, and that light-enhanced calcification via photosynthetic removal of CO2 does not generally apply to these organisms; he suggests that symbiotic algae may even compete with their hosts for inorganic carbon. Erez (2003) proposes a general model of biomineralization in foraminifera based on the precipitation of two types of calcite, high-Mg and low-Mg. However this model was criticized by Sadekov, Eggins, and De Dekker (2005), in particular as it applies to the symbionthosting benthic foraminifera Amphistegina lobifera and the planktonic species Orbulina universa, Globigerinoides ruber, and G. sacculifer because it predicts a pattern of Mg concentration within the bands in conflict with their observations.
Hathorne, James, and Lampitt (2009) investigated the detailed shell structure of two species of nonspinose planktonic foraminifera from the midnortheast Atlantic, Globorotalia inflata and G. scitula; both are essentially symbiont barren. Again they found banding of the Mg/Ca distribution through the test walls, and concluded that this is due to biomineralization. Although none of their suggested models could individually explain the observed patterns of variation, Hathorne, James, and Lampitt (2009) found, using whole-test calibrations, that temperatures based on mean Mg/Ca values from individual analyses generally matched measured growth temperatures; an exception was the Mg/Ca ratio in small G. scitula tests with only 3.5 chambers, which was higher than expected.
Geerken et al. (2018) found Mg-banding in the tests of two benthic foraminifera, closely correlated with Na-banding. Although a diurnally paced mechanism for this banding was deemed possible, Geerken et al. (2018) preferred some combination of other factors, viz. vacuolization, trans-membrane transport, a lattice strain effect and metastable precursor phases. In another culture study of banding in the same species, Geerken et al. (2019) established that magnesium, sodium, strontium and potassium are co-located in bands associated with organic linings in their tests. Furthermore changes in temperature and salinity induced coordinated changes in the banding pattern, which implies that independent changes in peak or trough height does not explain interindividual variability in element/Ca ratios.
Seasonal changes. Planktonic foraminifera show seasonal growth patterns, often becoming more abundant in warmer conditions, as well as producing seasonal temperature indications through their test δ18O and Mg/Ca values; these temperature changes are naturally smaller for thermocline-dwelling species than for mixed-layer species (e.g. Anand, Elderfield, and Conte 2003; Davis et al. 2016; Hönisch et al. 2013; Huang et al. 2008; Jentzen et al. 2018; Pak, Lea, and Kennett 2004; Regenberg et al. 2009; Weinkauf et al. 2016). In a core-top study of benthic foraminifera from the Arctic, Skirbekk et al. (2016) found seasonal reproduction and growth patterns in three species: Islandiella helenae/norcrossi reproduce and grow during summer, Buccella frigida in summer and autumn, and Nonionellina labradorica in autumn. In the appropriate seasons Skirbekk et al. (2016) found meaningful Mg/Ca-temperature correlations, which implies that resulting temperatures were seasonal rather than annual averages.
Several species of planktonic foraminifera are characterised by lunar abundance and shell flux variations, which have been attributed to a lunar-paced reproductive cycle (Davis et al. 2016; Jonkers et al. 2015). In sediment trap studies Weinkauf et al. (2016) investigated the effect of seasonal changes in temperature, productivity and growth conditions on calcification intensity (measured by size-normalised weight) in three species of planktonic foraminifera. Carbonate saturation was kept effectively constant, but they found significant inter-species differences in response to these factors. More generally, although seasonal effects are in one sense a complicating factor, the results of relevant sediment trap and core top studies can serve as a framework for interpreting subtle details in downcore Mg/Ca data provided that species are correctly identified and inter-species differences accounted for.
Habitat depth changes. Several studies have shown that planktonic foraminifera typically live at different depths and so record different temperatures whether based on δ18O or Mg/Ca; G. ruber generally gives the closest approach to SST (Anand, Elderfield, and Conte 2003; Barker et al. 2005; Dekens et al. 2002; Elderfield and Ganssen 2000; Regenberg et al. 2009; Sadekov et al. 2009). Thus an estimate of the depth range in which sample tests grew is important for interpretation of the temperature signal they carry.
We have noted that planktonic foraminifera often migrate vertically through the water column, calcifying at greater depths as they mature (Barker et al. 2005; Sadekov et al. 2009). For example, Marr et al. (2011) found that Mg/Ca in Globigerina bulloides from the southwest Pacific varied between chambers, the smallest value being found in the final (largest) chamber; this ontological change was interpreted as due to the forams sinking into cooler water during formation of the final (fourth) chamber. Marr et al. (2011) recommended using Mg/Ca from the penultimate or older chambers for SST reconstructions.
Shell size and ontogeny. Shell size was investigated by Elderfield, Vautravers, and Cooper (2002) for 17 species of planktonic foraminifera in six size fractions, the smallest 212–250 μm and the largest >500 μm. Apart from two globorotaliid species, Mg/Ca increased with shell size. This increase is generally in line with temperatures deduced from δ18O measurements, but species which live near the surface show larger Mg/Ca changes than is consistent with the temperature changes. For calibration Elderfield, Vautravers, and Cooper (2002) recommend the use of a single size fraction, in most cases the largest, but note that in absolute terms this will vary between species.
As for ontogeny, Nürnberg, Bijma and Hemleben (1996) found in cultured G. sacculifer specimens that had undergone gametogenesis that the Mg/Ca ratio increased from inner to outer layers of the final chamber primary calcite. They also found that so-called GAM calcite, a secondary layer deposited on the test just before gametogenesis, was considerably enriched in Mg/Ca relative to the primary calcite, the enrichment exceeding the reduction naturally due to calcification in a deeper, cooler environment. Dekens et al. (2002) noted evidence of GAM calcite in G. sacculifer but found none in G. ruber. Sadekov et al. (2009) measured Mg/Ca profiles through the walls of the final chambers in three species of planktonic foraminifera. They found that Mg/Ca values in the low-Mg layer in the final chamber in G. sacculifer and G. ruber and in the cortex (outer) layer in the final chamber in Pulleniatina obliquiloculata could be used as temperature indicators in addition to whole-test values. We have already discussed (see above) ontogenetic effects interwoven with depth change effects in G. bulloides in the work of Marr et al. (2011). Dueñas-Bohórquez et al. (2011) found in cultured G. sacculifer that the calcite Mg/Ca value decreased by an average of 0.43 mmol/mol between successive chambers (four chambers in total).
Summary and appraisal of Mg/Ca palaeothermometry. (1) Mg/Ca thermometry is based on the temperature-dependent substitution of magnesium for calcium in the calcite crystal lattice in the shells of calcite-forming marine organisms including foraminifera, ostracods, mussels and brachiopods; of these, foraminifera, especially planktonic foraminifera, are the most widely studied. Since this element substitution is endothermic, the shell Mg/Ca ratio is expected to increase with temperature. In practice the Mg/Ca ratio in biogenic calcite is lower than in inorganically precipitated calcite, but is more sensitive to temperature. This implies biological control over the calcification process.
(2) The particular attraction of Mg/Ca thermometry is that Mg/Ca ratios can be (and usually are) measured in the same fossil samples as δ18O values. Comparison of these two thermometers can be used to reconstruct seawater δ18O values, which may then permit reconstruction of salinity and continental ice volume (Rosenthal and Linsley 2006).
(3) Many Mg/Ca-temperature calibrations have been carried out since the mid-1990s, mostly for foraminifera, on the basis of culture, sediment trap and core top studies. Most of these have produced exponential Mg/Ca-temperature curves, though a few are better fit by a linear relationship. In terms of temperature, quoted uncertainty margins are typically within ±2.5°C. Species-specific calibrations give smaller uncertainty margins than generic calibrations.
(4) Most Mg/Ca temperature reconstructions have focused on near-surface temperatures in the Quaternary, but Mg/Ca palaeothermometry has been extended as far back as the Cretaceous, and Mg/Ca with δ18O from benthic foraminifera together with sea level data have been used to reconstruct deep-sea temperatures and cooling of ~12° since the early Eocene (Cramer et al. 2011; Lear, Elderfield, and Wilson 2000).
(5) Several factors complicate the application of Mg/Ca thermometry. Although difficult to separate, they may be considered under three headings, viz. diagenetic, environmental and vital effects. Diagenetic effects include shell dissolution, which tends to reduce Mg/Ca values, and calcite encrustation, which often adds high-Mg calcite. Environmental effects include the seawater magnesium concentration (especially over long time scales), salinity, carbonate ion (CO32-) concentration and pH; some calibrations include these factors explicitly. Vital effects include interindividual variability, the intra-test magnesium distribution (notably Mg-banding), seasonal changes, habitat depth changes, shell size and ontogenetic effects.
(6) Provided that complicating factors can be reliably identified and quantified with sufficient accuracy, Mg/Ca reconstructions of SST, thermocline or deep water temperatures may be achieved within a 2σ uncertainty within (sometimes well within) ±2.5°C. However all results obtained by this method should be treated with caution: it is still subject to development and refinement, and the pitfalls are not always obvious.
Trace Element Ratios in Corals (Sr/Ca And Li/Mg)
The aragonite skeletons of scleractinian corals are considered a sensitive and valuable source of palaeoclimate data, especially as they are potentially capable of providing subannual (seasonal) time resolution (Alibert and McCulloch 1997; Beck et al. 1992; Fowell et al. 2016; Gagan et al. 2000; Lea 2014; Swart, Elderfield, and Greaves 2002; Tierney et al. 2015). The various palaeotemperature indicators associated with these corals include δ18O, together with several based on the uptake of auxiliary cations, notably Sr2+ (Beck et al. 1992), Mg2+ (Mitsuguchi et al. 1996), Li+ (Marriott et al. 2004), and even U6+ (Felis and Pätzold 2004; Min et al. 1995). Although the coral Mg/Ca ratio has been used as a SST indicator (Mitsuguchi et al. 1996), it is not generally regarded as reliable when used independently of other element ratios (Montagna et al. 2014). The element ratio most commonly used as a coral temperature indicator is Sr/Ca (e.g. Alibert and McCulloch 1997; Beck et al. 1992; DeLong et al. 2014; Maupin, Quinn, and Halley 2008; Swart, Elderfield, and Greaves 2002; Wu et al. 2013; Zinke et al. 2019). Since Sr2+ ions are larger than Ca2+ ions, strontium substitution into the aragonite crystal lattice is exothermic and therefore the Sr/Ca ratio should decrease with increasing temperature (Rosenthal and Linsley 2006). As with Mg/Ca and δ18O in foraminifera, Sr/Ca and δ18O can be measured in the same coral samples to give the seawater δ18O value.
However a number of studies have cast doubt on the reliability and usefulness of the Sr/Ca thermometer. Complications and uncertainties arise from various sources, including: (1) algal symbiosis (Cohen et al. 2002; Lea 2014); (2) differing Sr/Ca-SST calibrations between different coral species, different individuals of the same species and even within the same colony (Alpert et al. 2016; Gaetani et al. 2011, Rosenthal and Linsley 2006; Saenger et al. 2008); (3) kinetic processes such as growth rate (Cohen et al. 2001; Lea 2014); (4) Rayleigh fractionation (which can affect all element/calcium ratios in aragonite; Grove et al. 2013); Gaetani et al. 2011; Kuffner et al. 2012; (5) differing interlaboratory standards (Hathorne et al. 2013a).
These complicating factors have prompted a search for alternative SST proxies, notably Li/Mg ratios (Fowell et al. 2016; Hathorne et al. 2013b; Marchitto et al. 2018; Montagna et al. 2014). The potential of this indicator lies in the opposing temperature controls on Li/Ca and Mg/Ca in the coral skeleton, which amplify the sensitivity of Li/Mg to SST. Also, Li/Ca and Mg/Ca respond similarly to Rayleigh fractionation, implying that precipitation progress does not significantly influence the Li/Mg ratio of the calcifying fluid; coral calcification involves a common seawater transport mechanism for cations, notably magnesium, strontium and boron (Gagnon, Adkins, and Erez 2012). Using cores of twentieth-century growth, Fowell et al. (2016) investigated the skeletal Sr/Ca and Li/Mg ratios of the coral Siderastrea siderea from the forereef and backreef zones of the Mesoamerican Barrier Reef System in the Caribbean. From field calibrations they found that Li/Mg and Sr/Ca ratios correlate well with SST, although both ratios are three times more sensitive to temperature change in the forereef than in the backreef. This implies the influence of another (unidentified) factor in addition to SST on element uptake during coral skeleton formation. Fowell et al. (2016) find that the use of a combined Sr/Ca and Li/Mg multiproxy calibration improves the precision of these SST reconstructions. However in a SST study based on element ratios in modern Porites corals, Zinke et al. (2019) found that Sr/Ca ratios gave a better match than Li/Mg ratios to measured SST changes.
Summary and appraisal of coral Sr/Ca palaeothermometry. The use of Sr/Ca in aragonitic corals as a SST indicator is attractive because of its potential for revealing seasonal changes. However, along with related element ratios such as Li/Mg, it is less well developed and understood, and subject to greater uncertainties, than Mg/Ca palaeothermometry. At present palaeo-SSTs based on this method should be treated with great caution, but improvements can be expected in the coming decades.
Biomolecular Temperature Indicators
Some marine sediment contains biogenic molecules, specifically cell membrane lipids (fats), with measurable characteristics which depend on their temperature of formation. On the assumption that these molecules have not been degraded or otherwise modified since they were formed and deposited, they can serve as temperature proxies. The temperature in question is usually the SST or the temperature of the uppermost part of the water column to a depth of 200 m or less; for climate scientists this is usually the most important ocean temperature.
Biomolecular temperature reconstructions are based on the principle that microorganisms adjust the rigidity of their cell membranes in response to environmental temperature (e.g. Ray, White, and Brock 1971). By altering the number of double bonds, rings, or branches in their phospho- or glycolipid membranes, microbes raise or lower the melting point of their cell structures and thereby adjust membrane fluidity. Useful lipids for palaeotemperature reconstructions must be: (1) well preserved and abundant in ancient sediments; (2) relatively easy to isolate and identify; and (3) reasonably specific to an organism such that temperature calibrations can be determined in both controlled experiments and in the natural environment (Tierney 2012, 2014). Requirement (3) presumes that the organisms responsible for producing the molecules of interest are not extinct. The use of these lipid molecules as temperature indicators is often linked in the mainstream literature with the fossil record of the organisms in view (Brassell 2014).
In order of discovery and usage we consider below the temperature-sensitive lipids of interest in a marine context, followed by a note of comparative studies which help to elucidate when and where they are applicable, and the uncertainties in the resulting temperature values.
(1) Alkenones, a type of ketone, first identified in sea floor sediments by Boon et al. (1978). Their use as temperature indicators was pioneered by Brassell et al. (1986). Alkenones are long single-chain molecules produced by certain haptophyte coccolithophores (single-celled marine green algae), notably Emiliania huxleyi and Gephyrocapsa oceanica, which grow in ocean surface waters. The relevant alkenones contain 37 carbon atoms in the central chain. The degree of unsaturation, the proportion of doubled carbon-carbon bonds, depends on the formation temperature: more unsaturated molecules are produced in colder water. Alkenone unsaturation thus serves as a SST indicator, expressed formally as the alkenone unsaturation index. This was initially denoted UK37 with an acknowledged temperature range of 11–28°C (Brassell et al. 1986). However a simpler version denoted UK'37 is now more commonly used; this has a useful temperature range of 0–29°C (Müller et al. 1998). Both indices are defined and their calibrations described in the Appendix. The upper limit of 29°C on the use of UK'37 appears to be fundamental because UK'37 reaches saturation (its maximum possible value of 1.0) at this temperature (Conte et al. 2006). Since the response of UK'37 to temperature is nonlinear above 24°C, declining towards its upper limit, interpretation of alkenone index data in tropical oceans is problematic. A statistical approach to this problem using spline fits and Bayesian regression has been proposed by Tierney and Tingley (2018).
Sachs et al. (2000), in seeking to provide recommended standards and procedures for the collection, preparation and use of alkenones as palaeoceanographic proxies, note a range of complicating factors. These include horizontal advection, vertical mixing and the effect of diagenesis on the unsaturation ratio. In order to ensure that apparent temporal offsets of climate proxies are correctly interpreted, Sachs et al. (2000) also suggest that:
. . . differential mixing rates of alkenone-containing particles and sand-sized foraminifera should be quantified by independent dating of the two phases.
However, in a biblical timescale temporal offsets are likely to be much smaller than envisaged by Sachs et al. (2000), or practically non-existent, which implies that the data may need a different interpretation. Sachs et al. (2000) also suggest that, at sites characterized by high deposition rates, alkenone investigations should be combined with other palaeoclimate proxy measurements. They further suggest that, in upwelling regions and in the vicinity of river plumes, salinity and nutrient proxies should be measured since alkenone synthesis is sensitive to changes in these parameters.
Herbert (2001) reviews alkenone calibrations by culture, water column and sediment core-top measurements. Culture studies show considerable variation in the UK'37-temperature relationship and suggest that growth rate as well as temperature can influence unsaturation. Assemblages of regional water column data sets give more consistent temperature calibrations than culture studies, but results based on basin-wide or global scales are not so useful. Globally based sediment core-top data produces a yet simpler picture in that sedimentary unsaturation indices follow a linear trend; the best correlation is that based on mean annual temperature in the upper 10 m of the water column. Exceptions are seen at high latitudes where highly seasonal production and salinity may affect sediment unsaturation ratios. Herbert concludes that much of the variance seen in culture and water column calibrations is absent in sediments, perhaps, he suggests, because of temporal averaging.
A more comprehensive review of alkenone palaeothermometry by Herbert (2003) suggests that UK'37 is generally a more robust SST proxy than those derived from foraminifera (i.e. δ18O and Mg/Ca) in that it survives extensive degradation in the water column and sediments, although Mg/Ca is preferable at temperatures above 25°C because of its higher temperature sensitivity. He notes that the alkenone index is generally a good proxy for mean annual SST, although this is not a straightforward relationship and can be distorted by regional variations in the factors which control the depth and seasonality of alkenone production. Herbert (2003) cites evidence of advection by deep currents distorting alkenone signals and their interpretation, but does not see this as a widespread problem. He notes that alkenone reconstructions of tropical SSTs at the LGM agree with Mg/Ca results for planktonic foraminifera in indicating a tropical cooling of 2–3°C (see also Visser, Thunell, and Stott 2003); thus alkenone palaeothermometry has contributed significantly to the question of SSTs at the LGM.
Dekens et al. (2008) compare SST records based on Mg/Ca and UK'37 from Pliocene and Pleistocene sediments in the eastern equatorial Pacific (EEP). They argue that these proxies can be meaningfully compared here because they relate to different organisms (Mg/Ca to planktonic foraminifera and alkenone unsaturation to coccolithophores, both well represented in the sediments here) and are subject to different biases (Mg/Ca needs environments with good calcite preservation while alkenone unsaturation measurements need high organic carbon preservation and temperatures < 29°C; both sets of requirements apply here). Dekens et al (2008) conclude that early Pliocene SSTs in the EEP were ~2–4°C higher than today, the results from the two proxies being in generally good agreement. However the favoured Mg/Ca calibration, based on core top measurements, typically gave temperatures about 1°C higher than the corresponding U K'37 figure. There was some uncertainty in the comparison arising mainly from uncertainty in the Mg/Ca calibration.
Marlowe et al. (1990) identified a series of both present-day and extinct coccolithophore species which they considered to be likely producers of alkenones and alkyl alkenoates found in ocean-floor deposits, reaching back to ~45 Ma BP in the conventional chronology. The oldest sediment in which alkenones have hitherto been identified is early Cretaceous porcellanite dated at 120.5 Ma (Brassell et al. 2004). Applying the standard alkenone temperature calibration to this material produces plausible values of SST, though Brassell et al. (2004) disclaim this as a meaningful temperature indication. At the time of writing the oldest material with published SST values derived from UK'37 measurements is early Eocene, conventionally dated at ~51 Ma (Brassell et al. 2004).
Prahl and Wakeham (1987, 369) throw an intriguing sidelight on the use of alkenones preserved in sediment as palaeotemperature proxies. They ask:
How far back in the geological record do long-chain, unsaturated ketones store palaeotemperature information? And, why are the long-chain, unsaturated ketones preserved in sediments at all? Most unsaturated lipids of phytoplanktonic origin do not survive passage through the pelagic food chain and hence, never even enter the sediment record. Long carbon chain length, unusual double bond positions and possibly molecular geometry provide at least partial explanations for the preservation of these intriguing biological markers.
These questions could prove worth investigating from a creationary perspective, since it is not clear that these biogenic molecules can be preserved intact in oceanic sediments for millions of years.
(2) GDGTs, iGDGTs or isoGDGTs (isoprenoidal glycerol dialkyl glycerol tetraethers, Schouten et al. 2002), for which the temperature indicator is known as TEX86, the TetraEther indeX of 86 carbons (Schouten et al. 2002; Tierney 2012, 2014). The membrane lipids used to calculate TEX86 are 86-carbon tetraethers produced by mesophilic marine and lacustrine Thaumarchaeota (previously known as Group I Crenarchaeota), such as Nitrosopumilus maritimus, a practically ubiquitous marine archaeon which oxidizes ammonia to nitrite. The tetraethers consist of paired C40 isoprenoid chains with varying numbers of cyclopentane rings and one cyclohexane ring connected by ether bonds to terminal glycerol groups. The detailed molecular structure of the most complex of these, crenarchaeol and its regioisomer, have been elucidated by Damsté et al. (2002) using NMR (nuclear magnetic resonance) imaging; see fig. 8. It appears that the cyclohexane ring here is crucial for the cold-tolerance of the Thaumarchaeota. Thus Damsté et al. (2002, 1649) state:
We have hypothesized that the formation of the cyclohexane ring is an adaption of the membrane lipids of hyperthermophilic archaea to relatively cold conditions in the open ocean.
In a biblical creation context, this proposed adaptation may have been included in the created genetic potential for diversification among the archaea.
Fig. 8. Structures of GDGTs relevant to construction of the TEX86 index. For glycerol dibiphytanyl glycerol tetraether GDGT-0, GDGT-4, and crenarchaeol, the numbering of carbon atoms is indicated. Items labelled II and III are GDGT-2 and GDGT-3 respectively. From Damsté et al. (2002), fig. 1.
The TEX86 value is calculated from the proportions of different numbers of these rings attached to each tetraether core structure. It is usually correlated with mean annual SST, but in some cases it reflects the temperature of subsurface water masses (Huguet et al. 2007). Tierney and Tingley (2015) imply that TEX86 may correlate equally well with the averaged temperature down to 200 m depth; the temperature range covered in their database is –3 to 30°C. Temperatures above 30°C were obtained by Robinson et al. (2017), but different variants of TEX86 and different calibrations produced temperatures differing by several degrees. The definition and temperature calibration of TEX86 are described in the Appendix, together with variant forms used in special circumstances (Kim et al. 2010; Liu et al. 2009a). An associated index termed the RI (Ring Index) was proposed by Zhang, Pagani, and Wang (2016) to determine whether iGDGT distributions from Mesozoic and Cenozoic sediments reflect SSTs alone, or whether they are influenced by other factors, e.g. production from terrestrial sources or within sediments, or indeed by non-thermal factors such as growth phase or nutrient level. RI is a weighted average of cyclopentane moieties which can be determined in the analysis used to determine TEX86. Zhang, Pagani, and Wang (2016) suggest that when RI and TEX86 indices deviate from the modern global TEX86-RI relationship, iGDGT distributions are not solely temperature-controlled, in which case TEX86-based temperature reconstructions are questionable. The definition of RI and the reference TEX86-RI relationship are given in the Appendix.
TEX86 has two acknowledged advantages over UK’37, viz. (1) it can be used at temperatures outside the range for alkenones, and (2) since GDGTs are produced by archaea, which have a fossil record extending back into the Precambrian, TEX86 may be used further down in the geological record (Tierney 2012, 2014). However point (2) is based on uniformitarian deep-time reasoning which assumes that the absence of a particular fossil implies its absence from the earth; this may not apply in a biblical paradigm. A further possible advantage, though based on limited research, is that GDGT distributions may be less affected by advection than alkenones (Kim et al. 2009; Park et al. 2014) such that the TEX86 temperature signal is generally more likely to relate to in-situ conditions. TEX86 has been used as a temperature indicator for middle Jurassic (Jenkyns et al. 2012) and even for early Jurassic sediments, corresponding to ~191 Ma in the uniformitarian chronology (Robinson et al. 2017).
(3) Long-chain alkyl (or alkane) diols. Rampen et al. (2012) studied a sediment core from the South Atlantic close to the Congo River outflow (West Africa), which in uniformitarian terms provided a 43 ka SST record. The temperature indicator here is LDI, the abundance of the C30 alkyl 1,15-diol relative to the combined C28 1,13-, C30 1,13- and C30 1,15-diol abundance; see Appendix for the diol naming convention, the definition of LDI and the annual mean SST calibration adopted by Rampen et al. (2012). The temperature range of the calibration is –3 to 27°C. Rampen et al. (2012) deduce that in a marine context the diols of interest were probably generated by photoautotrophic algae within the top 30 m of the water column; however they could not unequivocally identify the organisms responsible. In fresh water the appropriate diols are produced by eustigmatophyte algae, but the marine equivalent (Nannochloropsis) produces long chain diol distributions dominated by the C32 1,15-diol, which is relatively scarce in marine sediments. Nannochloropsis is therefore not considered to be a likely source of marine diols. Naafs, Hefter, and Stein (2012) applied the LDI proxy to a sediment core from the mid-latitude North Atlantic (near the Mid-Atlantic Ridge) spanning an early Pleistocene section conventionally dated at 2.49– 2.41 Ma (i.e. a complete glacial/interglacial interval). The results agreed well with UK’37 -based SST values and followed a similar trend to benthic δ18O-based temperatures. However, Naafs, Hefter, and Stein (2012) also could not identify the source organisms. A more recent study seeking to identify the biological sources of the relevant lipids by comparing lipid and genetic analyses of suspended particulate matter in the tropical North Atlantic has so far also produced inconclusive results (Balzano et al. 2018).
(4) Comparative studies involving biomolecular temperature indicators. Apart from culture-based or contemporary marine studies in which temperatures can be measured directly, most studies involving biomolecular temperature proxies provide comparisons with more traditional proxies, usually δ18O or Mg/Ca. Thus, for example, Brassell et al. (1986) introduce the alkenone unsaturation index via its correlation with δ18O values for the planktonic foraminifera Globigerinoides sacculifer and Globigerinoides ruber and the benthic foraminifer Cibicidoides wuellerstorfi. Dekens et al. (2008) compare results from Mg/Ca and UK’37 measurements from a site in the eastern equatorial Pacific down to the early Pliocene, about 5 Ma BP in conventional terms. They conclude that the temperatures recorded by these proxies generally agree provided that a core-top Mg/Ca calibration including a suitable dissolution correction is used; UK’37 data is taken to represent SST. Liu et al. (2009a) investigate a globally based set of SST records based on UK’37 and TEX86 data, together with corresponding δ18O data from benthic foraminifera through the Eocene-Oligocene transition. The UK’37 and TEX86 SST values are generally close together, but a few degrees higher than the corresponding δ18O values. For high-latitude and polar sites this is interpreted by Liu et al. (2009a) as indicating that while benthic δ18O records reflect deep-water production during winter months, UK’37 and TEX86 values capture mean annual SSTs which are probably biased toward late spring/early autumn temperatures.
Richey and Tierney (2016) measured GDGT and alkenone fluxes and UK’37 and TEX86 indices in the northern Gulf of Mexico over a 4-year (2010–2014) sediment trap time series and compared the data with core-top sediments at the same location. The sediment trap sampled the sediment flux from the overlying water column. The results show some differences between the two proxies, the alkenone signal reflecting the near-surface, mean annual temperature in the region while TEX86 indicates an integrated mean annual subsurface (0–200 m) temperature. Standard UK’37 calibrations are not fully satisfactory at higher SSTs because of the nonlinear UK’37 (T) relationship in this range (Conte et al. 2006).
Becker et al. (2015) developed and introduced a laboratory protocol for producing rapid and simultaneous UK’37, TEX86, and LDI analyses on samples taken from the same sediment core. Their test core was taken from the Sea of Marmara, and covered the period since the LGM (conventional date 21 ka BP). Major climatic changes took place during this time, including the formation of sapropel S1, dated according to Becker et al. (2015) at about 10 ka (see fig. 9). Unit 1 represents lacustrine conditions, while Unit 2 represents marine and Holocene conditions. While the diols and iGDGTs were especially abundant during sapropel S1, the authors concluded that there were sufficient concentrations of all the relevant lipids to construct meaningful SST plots throughout. Fig. 9b shows that these proxy-derived SST values are in broad agreement in Unit 1 and afterwards, with alkenones generally giving the lowest SST values and diols the highest. However Becker et al. (2015) regard the high alkenone-derived SSTs for the last glacial (up to about 15 ka BP) as unrealistic; they reason that the UK’37/SST transfer function is not applicable because the alkenone-producing organisms in the lacustrine environment are very different from Emiliania huxleyi, being characterized by a very different proxy/SST relationship.
Rodrigo-Gámiz et al. (2015) investigated the applicability of UK’37, TEX86, and LDI to SST reconstructions for the subpolar region around Iceland. They found (i) anomalous LDI-SST values in core top sediments and (ii) low mass fluxes of 1,13- and 1,15-diols relative to 1,14-diols, inferring that Proboscia diatoms, not eustigmatophyte algae, were the main source of long-chain alkyl diols in that area and concluding that the LDI index cannot be used there. Flux-weighted average temperature estimates had significant negative (approximately –2.3°C for UK’37) and positive (up to +5°C for TEX86) offsets against satellite-derived SSTs and temperature estimates derived from core top sediment. UK’37 estimates from surface sediments around Iceland correlated well with summer mean SSTs, while TEX86-derived estimates followed both annual and winter mean 0–200 m temperatures, suggesting a subsurface temperature signal.
Summary and appraisal of biomolecular temperature indicators. Temperature reconstructions based on biomolecular proxies cannot easily be compared since the residual lipid molecules are produced by different organisms and are subject to different biases (e.g. seasonality, the depth of the water column sampled, diagenetic alteration etc). Furthermore the alkenone index is limited to temperatures not exceeding 29°C, and the organisms which produce marine diols of interest remain unidentified. In some cases alkenone-based temperature signals may be compromised by advection of the alkenone-bearing material; this may be less of a problem for GDGTs. The use of biomolecular indicators has largely been confined to the upper part of the geological record, and none of them indicates ocean floor or globally representative temperatures. Although biomolecular temperature reconstructions should be treated with great caution, further developments can certainly be expected, and the geological range of the archaea suggests that GDGTs at least will be of great interest for research into pre-Cenozoic ocean temperatures.
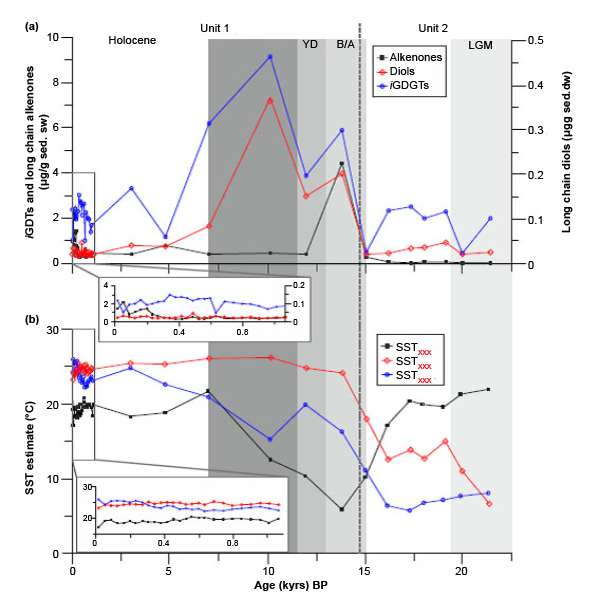
Fig. 9. (a) Total iGDGT, diol and alkenone concentrations (μg/g sediment dry weight) against conventional age; (b) SST estimates for cores GeoB15104-2 and -4 from the Sea of Marmara based on alkenones, diols and archaeal iGDGTs. Climatic events marked here are the Last Glacial Maximum (LGM), Bølling/Allerød, Younger Dryas and Sapropel S1. Unit 1 refers to the Holocene and marine and Unit 2 to the lacustrine phases in the history of the Sea of Marmara. Taken from fig. 5 of Becker et al. (2015).
Clumped Isotope Palaeothermometry
This more sophisticated version of oxygen isotope palaeothermometry has emerged within approximately the last 13 years. It seeks to quantify how 18O and 13C atoms are distributed in the lattice of the carbonate crystal by distinguishing “isotopologues”, molecules of similar chemical composition but different isotopic composition. When carbonates are reacted with orthophosphoric acid, they produce CO2 gas in isotopic equilibrium (subject to a constant offset linked to acid temperature) with the original carbonate. The technique involves measuring the proportion in this CO2 of the isotopologue with an atomic mass number of 47, viz. 13C18O16O, where the two “heavy” rare isotopes (13C and 18O) are substituted in the CO2 molecule. This represents the amount of “clumping” of the heavy isotopes in the carbonate crystal lattice (Carbonate Research 2015), and depends on its formation temperature. The result is expressed as Δ47‰, and its use is described by Ghosh et al. (2006) thus:
The abundance of the doubly substituted CO2 isotopologue, 13C18O16O, in CO2 produced by phosphoric acid digestion of synthetic, inorganic calcite and natural, biogenic aragonite is proportional to the concentration of 13C–18O bonds in reactant carbonate, and the concentration of these bonds is a function of the temperature of carbonate growth. This proportionality can be described between 1 and 50°C by the function: Δ47 = 0.0592 · 106 · T−2 − 0.02, where Δ47 is the enrichment, in per mil, of 13C18O16O in CO2 relative to the amount expected for a stochastic (random) distribution of isotopes among all CO2 isotopologues, and T is the temperature in Kelvin.
Ghosh et al. (2006) claim a 1σ temperature precision within ±2°C for Δ47 thermometry. Its calibration up to 50°C has been reassessed and refined by Zaarur, Affek, and Brandon (2013) and its temperature range has since been extended up to 250°C (Kluge et al. 2015). In the experiments of Kluge et al. (2015) the temperature was set at a series of fixed values with quoted precision ±2°C, while resulting Δ47 values were quoted with 1σ uncertainty of order ±0.01‰ (~2% of central values). Came, Brand, and Affek (2014) report a calibration based on modern brachiopods. Their results align well with those of Zaarur, Affek, and Brandon (2013), but they note significant discrepancies with other calibrations.
Δ47 thermometry can be used in both biogenic and non-biogenic carbonate systems and in marine and terrestrial settings; for example, it has been used to constrain the formation temperatures of eggs in modern birds and in dinosaurs (Eagle et al. 2015); Bernasconi et al. (2018) list other applications. A valuable feature in the marine context is that it does not depend on the δ18O value of the water in which the carbonate grew. Hence measurements of Δ47 in a sample, which necessarily involves measuring sample 18O, can in principle be used to reconstruct both the temperature and δ18O value of the water.
The derivation of Δ47 values from raw mass spectrometer data depends on the absolute isotope abundance ratios in reference materials, specifically VSMOW (Vienna Standard Mean Ocean Water) and VPDB (Vienna PeeDee Belemnite; see Ravelo and Hillaire-Marcel 2007 for definitions). To appreciate the extreme care in measurement and calibration necessary to obtain meaningful Δ47 data, note that: (1) the full Δ47 signal when the carbonate formation temperature changes from 0 to 1000°C is only of order 1‰ (Schauer et al. 2016); (2) the VSMOW scale for reporting oxygen isotope ratios of silicates and oxides is subject to ambiguity of up to 0.5‰, mainly because of differing procedures of oxygen extraction and differing definitions of the VSMOW scale between laboratories (Kusakabe and Matsuhisa 2008); (3) the natural abundance of the 13C18O16O isotopologue in analyte CO2 is a mere 44.4 ppm (Davies and John 2017).
Meaningful Δ47 measurements therefore require both reliable contamination indicators and stringent sample purification procedures. Davies and John (2017) advocate a cold trap temperature as low as –60°C to reduce contamination to acceptable levels. Reliable Δ47 temperature calibrations also require an accurate figure for 17O fractionation (Schauer et al. 2016); to accommodate this Kelson et al. (2017) recommend the 17O correction proposed by Brand, Assonov, and Coplen (2010). Daëron et al. (2016) employ several approaches to investigate the influence on measured Δ47 values of the (13C/12C) ratio in VPDB, the (18O/16O) and (17O/67O) ratios in VSMOW and λ, the slope of the “triple oxygen isotope line”, which defines a mass-dependent fractionation law (Brand, Assonov, and Coplen 2010). Having demonstrated that using different sets of these parameters can produce systematic offsets in Δ47 values as large as 0.04‰, Daëron et al. (2016) recommend using the parameter set proposed by Brand, Assonov, and Coplen (2010); this includes λ = 0.528, the observed value for natural waters (i.e. ocean and precipitation). In short, reliable Δ47 measurements demand extremely high laboratory standards (Bernasconi et al. 2018)
Stolper, Eiler, and Higgins (2018) investigated the effects on marine carbonate Δ47 values of balanced dissolution-reprecipitation reactions (i.e. recrystallization without change in the total mass of carbonate mineral) during diagenesis (burial and lithification) in both deep- and shallow-water settings. Their models are adaptations of 1D models developed by Richter and DePaolo (1987), Schrag, DePaolo, and Richter (1992) and others. Stolper, Eiler, and Higgins (2018) assume that diagenetic carbonate (i.e. which forms within the sediment column) forms in isotopic equilibrium with the pore fluids. This assumption is based on rates of carbonate precipitation in deep-sea sediments of 10-19 moles/m2/sec (Fantle and DePaolo 2007), which they note is 7 orders of magnitude lower than theoretically estimated rates (Watkins et al. 2013, 2014; Watkins and Hunt 2015). Given such a large discrepancy in carbonate precipitation rates in the mainstream literature, the source of these figures should be re-examined from the perspective of Flood and post-Flood timescales.
Stolper, Eiler, and Higgins (2018) conclude from their model that: (1) where the initial burial temperatures differ significantly (by tens of °C) from formation temperatures, both δ18O (carbonate) and Δ47 values are noticeably modified within the first km of burial; (2) comparison of model predictions with newly presented Δ47 data from ODP (Ocean Drilling Program; see IODP 2017) site 807 in the equatorial Pacific serves to verify the model while at the same time indicating, in line with previous studies, that Palaeogene equatorial western Pacific surface waters were warmer than they are today; (3) in shallow water settings, the main sedimentary systems preserved in Mesozoic and earlier rocks, the model predicts only minor changes (~0.01‰) in Δ47 values. This is as expected because formation and initial diagenesis occur at practically the same temperature.
Clumped isotope analysis of a suite of calcitic and phosphatic fossil shells from late Cambrian to mid-late Ordovician strata was used by Bergmann et al. (2018) in conjunction with petrographic and element analysis to reconstruct both SSTs and seawater δ18O levels in the early Palaeozoic. The modern brachiopods used for calibration included both rhynchonelliform (calcitic) brachiopods and both extant linguliform genera, Lingula and Glottidia, which are phosphatic, from a range of locations; the calibration suite covered a temperature range of 2–35°C. A key aspect was the use of paired calcitic and phosphatic fossil materials taken from the same stratigraphic sections. The point of this approach was that biogenic phosphates behave differently from biogenic calcite during diagenesis and thus serve as a valuable geochemical archive of oceanic conditions before GOBE, the “Great Ordovician Biodiversification Event” (Servais et al. 2009) in which apparent marine biodiversity increased drastically, notably in organisms producing thick-shelled calcitic skeletons.
Veizer and Prokoph (2015) cite reports of unrealistically high Palaeozoic ocean temperatures obtained by Δ47 thermometry (e.g. Henkes et al. 2014). Henkes et al. (2014) report apparent temperatures derived from Δ47 values in calcite from late Mississippian (Carboniferous) brachiopods from the Bird Spring formation, eastern Great Basin province, Nevada, USA reaching up to 166±13°C (the quoted uncertainty margin is 1σ/√n where n is the number of samples, in this case 3); see fig. 10. They infer that the fundamental problem is diagenetic reordering of C-O bonds in the calcite crystal lattice when residing for long periods at high temperature, a process that cannot be detected via changes in shell microstructure or trace element concentration. Following Passey and Henkes (2012), Henkes et al. (2014) undertook laboratory experiments involving heating fossil brachiopod calcite at different temperatures and times to observe directly rates of 13C-18O bond reordering. From the results they were able to deduce the Arrhenius parameters which characterise the reordering reaction, referring to the first-order model7 of Passey and Henkes (2012) and their own more sophisticated model, described as a “transient defect/equilibrium defect” model. They concluded that calcite samples kept below about 100°C for 106–108 years should not be affected by solid state C-O bond reordering. The equivalent figures for dolomite are an upper temperature limit of 150°C for tens of millions of years (Lloyd, Ryb, and Eiler 2018). From a biblical perspective these timescales are unrealistic, which suggests that the assumed thermal histories and underlying physics should be re-examined with much shorter time scales in view.
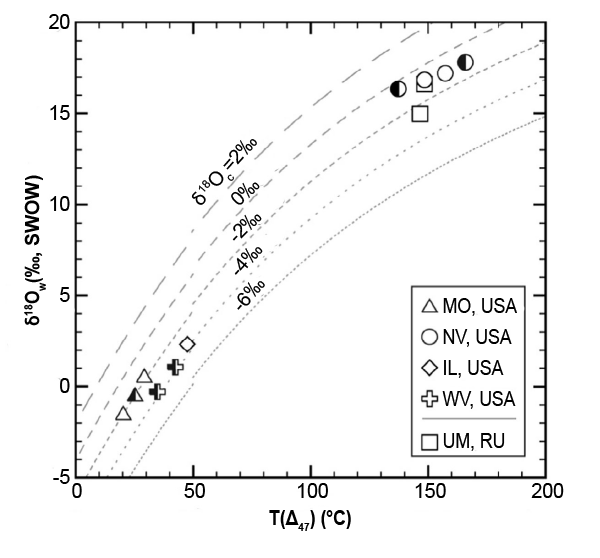
Fig. 10. Carboniferous brachiopod carbonate clumped isotope temperatures [T(Δ47)] versus apparent water oxygen isotope compositions of the precipitating waters (δ18Ow). From Henkes et al. (2014), fig. 2. Water oxygen isotope values were determined from measured carbonate δ18O and T(Δ47) using the calcite-water oxygen isotope temperature calibration of Kim and O’Neil (1997) up to 50°C; above this temperature the calibration of O’Neil, Clayton, and Mayeda (1969) was used. Open symbols represent non-luminescent shells while half-filled symbols represent semi-luminescent shells. The grey dashed and dotted lines represent solutions to the calcite-water oxygen isotope thermometry equations for constant carbonate δ18Oc values as noted on the figure. The sample source locations are noted on the figure using normal U.S. state identifiers (e.g. MO USA=Montana, USA, and so on); UM, RU=Ural Mountains, Russia.
Breitenbach et al. (2018) have undertaken coupled Mg/Ca and Δ47 studies of core top samples of planktonic foraminifera from several oceans, claiming from their results that this combination can provide reliable and consistent temperature estimates.
Summary and appraisal of clumped isotope palaeothermometry. Although the clumped isotope method looks very promising and is currently the subject of vigorous development, results obtained to date should be treated with caution. However the method can be applied so widely using fossil and mineral samples from the whole geological record that it will inevitably be developed further. Creation scientists are well advised to keep abreast of future developments.
Interpretation of the Data in Terms of Flood Geology
Most of the commentary on the various palaeothermometers discussed in this article would apply either on conventional or biblical time scales. Although the life spans of relevant fossil-producing organisms are not generally well known, it is unlikely that many species live for years; brachiopods are an obvious exception. Weeks or months seem more typical for foraminifera and ostracods; see, for example, Nigam, Saraswat, and Mazumder (2003) regarding the life spans of planktonic foraminifera. This is short compared with expected timescales of environmental change in a post-Flood scenario. Violent rapid changes in ocean conditions during the Flood year itself are most likely to be manifest in sudden changes in faunal assemblages, which are not considered here.
In general terms changes in ocean conditions were probably much gentler after the Flood than during the Flood. Thus reconstructed temperatures from post-Flood sediment cores should, subject to proper calibration and allowance for complicating factors, fairly represent sequences of temperature changes. During the Flood whole ecosystems were probably transported, rapidly and often violently, from their pre-Flood environments and may have become mixed and buried with others. Consequently fossil shells or tests within Flood sediments would probably give relatively messy, perhaps confusing, palaeotemperature signals. Thus where there are both Flood and post-Flood sediments on the ocean floor, temperature-related data from cores penetrating the Flood/post-Flood boundary might show a change in character from inconsistent and confusing below the boundary to more consistent above it. This in turn could serve to enable mapping of the Flood/post-Flood boundary on the ocean floor. However none of the literature considered in this paper has presented results obviously following such a pattern; the idea remains conjectural.
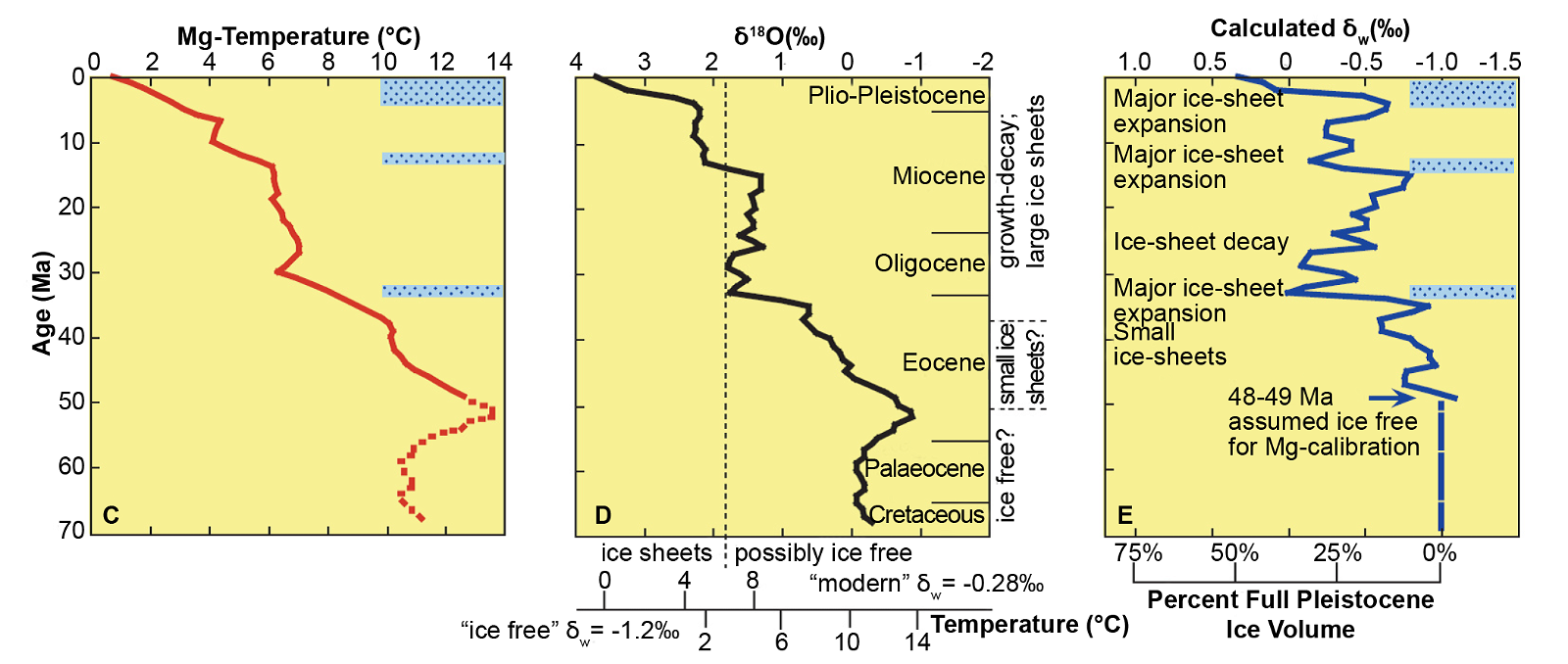
Fig. 11. Curve (C) represents a smoothed, averaged temperature record based on a Mg/Ca calibration applied to the suite of benthic foraminiferal samples studied by Lear, Elderfield, and Wilson (2000). The broken line indicates temperatures calculated from the δ18O record assuming an ice-free world. Blue areas indicate periods of substantial ice sheet growth determined from the δ18O record combined with the Mg/Ca record; (D) represents the Cenozoic composite benthic foraminiferal δ18O record based on Atlantic cores and normalized to Cibicidoides spp. (from Miller, Fairbanks, and Mountain 1987). The vertical dashed line indicates probable existence of ice sheets (δw is seawater δ18O); (E) shows estimated variation in seawater δ18O, a measure of global ice volume. The “full Pleistocene ice volume” scale is from Dwyer et al. (1995). From Lear, Elderfield, and Wilson (2000), fig. 1.
The temporal coverage of the proxy data of interest is not sufficiently dense to construct plots of (say) Mg/Ca-derived temperatures through the Cenozoic to rival the δ18O plot presented by Veizer and Prokoph (2015). A plot of Mg/Ca and δ18O-based deep-sea temperatures since the early Eocene is presented by Lear, Elderfield, and Wilson (2000), who used data from six species of benthic foraminifera from four well-separated sites to deduce an overall cooling of 12°C since the early Eocene; see fig. 11.
A more sophisticated study, again based on benthic foraminiferal Mg/Ca and δ18O together with sea-level records (Cramer et al. 2011), produced similar results. Although this study extended further back into the Cretaceous, there was no Mg/Ca data from earlier than the Palaeocene (~62 Ma BP in conventional terms); see figs. 12 and 13. A puzzling feature of the work reported by Cramer et al. (2011) is that cooling episodes do not necessarily correlate with ice volume growth, expressed thus in their Abstract:
Our reconstructions indicate differences between deep ocean cooling and continental ice growth in the late Cenozoic: cooling occurred gradually in the middle-late Eocene and late Miocene-Pliocene while ice growth occurred rapidly in the earliest Oligocene, middle Miocene and Plio-Pleistocene.
However, Cramer et al. (2011) acknowledge their ignorance of the mechanisms underlying these episodes of rapid ice sheet growth. This apparent timing mismatch should perhaps be investigated from a Flood geology perspective. In terms of temperature the results obtained by Lear, Elderfield, and Wilson (2000) and by Cramer et al. (2011), although subject to significant uncertainties, tie in closely with the corresponding δ18O data and support the conclusion of Worraker (2018) that deep ocean temperatures never rose above 13°C during the Cenozoic. Post-Flood Ice Age models which assume significantly higher bulk ocean temperatures and in which Cenozoic deposits represent the post-Flood period conflict with these findings.
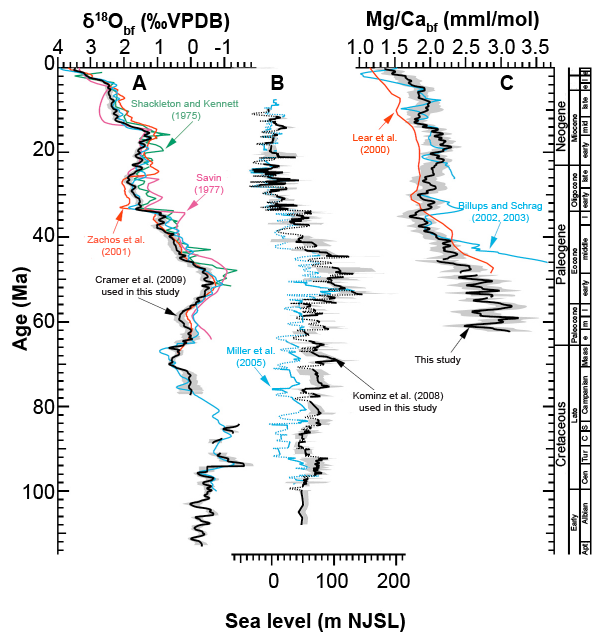
Fig. 12. Primary reconstructed proxy records used by Cramer et al. (2011), viz. (A) δ18Obf, (B) NJSL, and (C) Mg/Cabf. From Cramer et al. (2011), fig. 1. Records used in the study are shown in black; prior and alternate versions of these records are shown in colour for comparison. Shaded areas are uncertainty envelopes; in the case of curves (A) and (C) these delineate the 90% confidence interval. Cramer et al. (2011) note that there is better correspondence of δ18Obf trends, as reconstructed in separate studies spanning four decades, than with Mg/Cabf in studies spanning only one decade, which reflects the greater difficulty in reconciling Mg/Cabf compared with δ18Obf in different species and from different locations.
Key: δ18Obf (‰)—benthic foraminiferal δ18O relative to the VPDB (Vienna PeeDee Belemnite) standard; Mg/Cabf (mmol/mol)—benthic foraminiferal Mg/Ca ratio; NJSL (m)—sea level as measured in cores from the New Jersey coastal plain. See Grossman (2012) for definitions of δ18O standards.
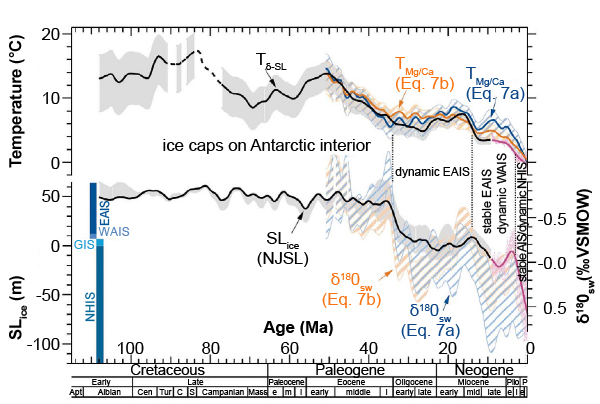
Fig. 13. Summary of temperature (upper curve) and ice volume (lower curve) reconstructions since the early Cretaceous, smoothed to only show variations on conventional time scales longer than 5Ma. From Cramer et al. (2011), fig. 9.
Key: NJSL (m)—sea level as measured in cores from the New Jersey coastal plain; SLice (m)—change in sea level due to growth and decay of continental ice sheets; TMg/Ca (ºC)—ocean temperature calculated from Mg/Cabf, the benthic foraminiferal Mg/Ca ratio (mmol/mol); δ18Obf (‰)—benthic foraminiferal δ18O relative to the VPDB (Vienna PeeDee Belemnite) standard; δ18Osw (‰)—seawater δ18O relative to VSMOW (Vienna Standard Mean Ocean Water); Tδ-SL (ºC)—ocean temperature calculated from δ18Obf using SLice to constrain δ18Osw. The heavy black curves are based on NJSL and δ18Obf data, while the lighter blue and orange curves are based on Mg/Cabf and δ18Obf, the two colours representing different Mg/Ca-temperature calibrations. Shaded coloured areas illustrate the uncertainty margins. EAIS, WAIS, GIS and NHIS are the East Antarctic, West Antarctic, Greenland and Northern Hemisphere ice sheets respectively. The vertical blue bars on the left indicate the approximate cumulative range of sea level variations due to ice sheets. See Grossman (2012) for definitions of δ18O standards.
Other results from the use of the temperature indicators discussed here relate to specific water depths, specific regions and specific points in geological history and do not add a great deal to the overall picture of ocean temperatures through time. However, there is considerable Mg/Ca coverage available of the Quaternary, including for example SSTs in equatorial, tropical and polar regions (Barker et al. 2005; Irvali et al. 2012, 2016; Levi et al. 2007; Nürnberg, Müller, and Schneider 2000), thermocline temperatures in the South Atlantic (Groeneveld and Chiessi 2011), Antarctic Intermediate Water temperatures (Elmore et al. 2015) and bottom water temperatures in the eastern equatorial Pacific (Sadekov et al. 2014). For temperature-time plots from a selection of these sources see figs. 14–19; in all cases the original references should be consulted for more detailed background information. Since the Quaternary is the geological interval of special interest for modelling a post-Flood Ice Age, these Mg/Ca-based results serve as useful ocean temperature data points against which to check model predictions. Other coverage from Mg/Ca results cited here includes Caribbean SSTs through the Miocene-Pliocene transition (Groeneveld et al. 2008; see fig. 20), thermocline and intermediate deep water temperatures in the Southern Ocean through the Eocene-Oligocene cooling (Bohaty, Zachos and Delaney 2012; see fig. 21) and mid-Cretaceous tropical Atlantic upper ocean temperatures (Bice et al. 2006; see figs. 22 and 23). Results from Bice et al. (2006) may be problematic, e.g. because of conflicting trends in δ18O and Mg/Ca data and the very high reported SSTs, but the others should again be treated as providing useful data points when modelling Flood or post-Flood environmental conditions. The literature cited here, of course, is by no means exhaustive.
We have noted certain questions and inconsistencies in the conventional literature which raise issues worth investigating from a creation science perspective. For example, we have cited a comment by Prahl and Wakeham (1987) questioning the longevity and integrity of alkenones, which have been used as palaeotemperature indicators as far back as the early Eocene, 51 Ma BP in the conventional chronology. Investigation of the degradation of these and other biogenic molecules (GDGTs and long-chain diols) in ocean sediments over time seems a worthwhile creation science exercise. The huge mismatch of 7 orders of magnitude between (supposedly measured) rates of carbonate precipitation in deep-sea sediments (Fantle and DePaolo 2007) and theoretical estimates (Watkins et al. 2013, 2014; Watkins and Hunt 2015) clearly deserves re-examination from a biblical viewpoint. The question of solid-state C-O bond reordering cited by Henkes et al. (2014) as the cause of unrealistically high Palaeozoic temperatures obtained by Δ47 palaeothermometry, supposedly occurring over multi-million year timescales, should also be re-examined.
Conclusions
Fig. 14. Down-core Mg/Ca records for two sites in the South Equatorial Atlantic, spanning the last 270ka in the conventional chronology, from figs. 2 and 3 respectively in Nürnberg, Müller, and Schneider (2000). Left-hand panels show the proxy data, while right-hand panels show the reconstructed temperature plots. (A) shows data from Globigerinoides sacculifer tests and compares Mg/Ca- and δ18O-derived temperatures, while (B) shows data from Globigerinoides ruber and compares Mg/Ca- and alkenone-derived (UK’37) temperatures. The thick black lines in these plots indicate smoothed data.
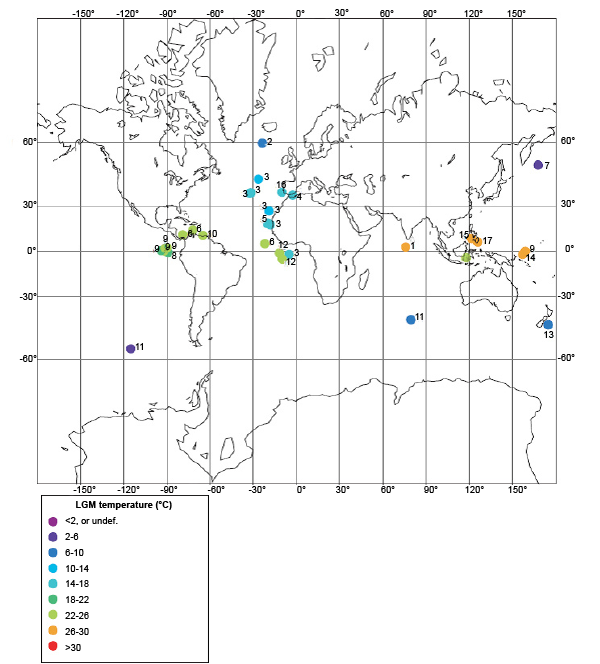
Fig. 15. Estimated SSTs at the Last Glacial Maximum (21kaBP in the conventional chronology) based on planktonic foraminiferal Mg/Ca data. Taken from fig. 5 in Barker et al. (2005). The site numbering identifies the data sources and is as noted in the original figure caption; location 3 refers to the work reported by Barker et al. (2005). The source of all the data used in constructing this map is the MARGO (‘Multiproxy Approach for the Reconstruction of the Glacial Ocean surface’) database (http://www.pangaea.de/Projects/MARGO/).
Fig. 16. δ18O and Mg/Ca record based on Globigerinoides ruber (white) tests from core MD98-2165 (southeast of Java, Indonesia: 9º39´S, 118º20´E, 2100m water depth), from fig. 3 in Levi et al. (2007). Time axis (labelled in units of 1ka) goes back to 26kaBP in the conventional chronology; YD is the Younger Dryas event, and\H1 the Heinrich 1 event (see Nomenclature). Empty triangles at the top indicate levels in the core with assigned dates.
1. All the ocean palaeotemperature indicators reviewed here, specifically the Mg/Ca ratio in calcite fossil shells, the Sr/Ca and Li/Mg ratios in scleractinian corals, the biomolecular indicators (the alkenone unsaturation index, the iGDGT index or TEX86, and the LDI or long-diol index) and the clumped carbonate index, suffer from significant complications and uncertainties. However all are subject to active current research and development, especially the very widely applicable clumped carbonate index, and improvements in accuracy and reliability can be expected in the coming decades, regardless of timescale assumptions. In many studies two or more of these indicators are combined, which can provide additional information (e.g. salinity and continental ice volume from simultaneous δ18O and Mg/Ca measurements) and facilitate assessment of complicating factors. In Mg/Ca palaeothermometry, the most mature and widely used of these methods, claims of an uncertainty of order ±2.5°C are reasonable provided that complicating factors have been assessed and accounted for.
2. Our most important conclusion, based on extensive combined benthic foraminiferal δ18O-Mg/Ca data and sea level data, is that deep ocean water has cooled by about 12°C since the early Eocene. This reinforces the conclusion in Worraker (2018) that deep ocean temperatures never exceeded 13°C through the Cenozoic, an important constraint on Flood and post-Flood environmental models.
3. The temperatures derived from proxy studies restricted to particular intervals in the geological record, particular areas and particular water depths (surface, thermocline, bottom water etc.) can, subject to scrutiny from a creation science perspective, be used as reference data points against which the predictions of Flood and post-Flood environmental models may be checked; examples are the extensive δ18O-Mg/Ca coverage of the Quaternary, and the cooling of thermocline and intermediate deep water in the Southern Ocean across the Eocene-Oligocene transition (Bohaty, Zachos, and Delaney 2012). The exact use of such data points will depend on how a particular model is chosen to relate to the geological record.
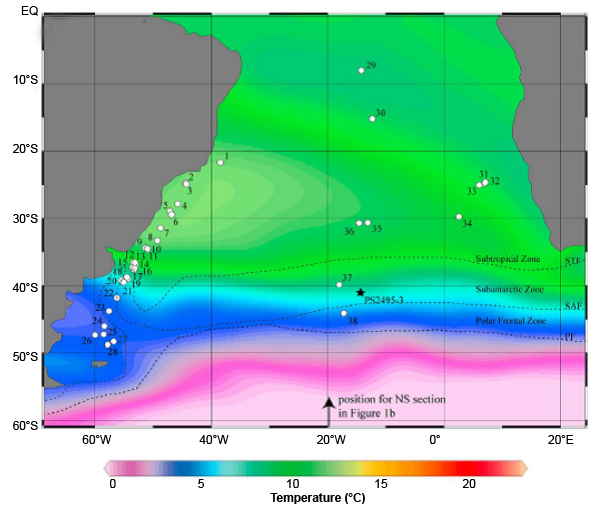
Fig. 17. South Atlantic map, colour-coded to show present-day mean annual temperatures at 350m depth. Taken from fig. 1 in Groeneveld and Chiessi (2011). White circles show sampling sites. The location of core PS2495-3 (see fig. 18) is marked by a black star. Dashed lines show the boundaries between the Subtropical Zone (STZ), Subantarctic Zone (SAZ) and Polar Frontal Zone (PFZ) (see Peterson and Stramma 1991).
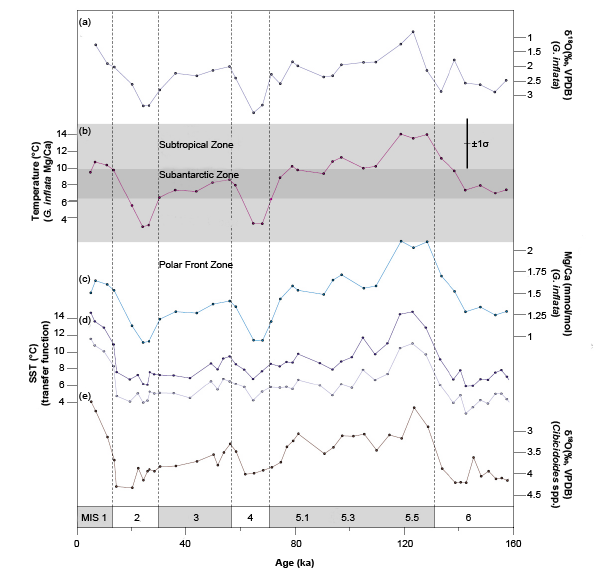
Fig. 18. Downcore records from gravity core PS2495-3 (41.27ºS, 14.49ºW, 3134m water depth) covering the last 160ka in the conventional chronology. Taken from fig. 4 in Groeneveld and Chiessi (2011). The various plots are: (a) G. inflata δ18O; (b) G. inflata Mg/Ca palaeotemperatures; (c) G. inflata Mg/Ca; (d) summer and winter SSTs based on foraminiferal transfer functions (for a description of the underlying methodology, see Kucera et al. 2005); (e) Cibicidoides spp. δ18O. Horizontal grey areas give a possible indication of when core PS2495-3 was located in the Subtropical Zone, Subantarctic Zone or Polar Frontal Zone (Peterson and Stramma 1991). Numbers on the lower portion of the plot depict Marine Isotope Stages (MIS), and vertical dashed lines mark the boundaries between adjacent stages. The vertical black bar associated with plot (b) depicts the ±1σ uncertainty in the Mg/Ca-temperature calibration used by Groeneveld and Chiessi (2011). The frequentlycited system of Marine Isotope Stages is described by Railsback et al. (2015).
4. We have found some puzzling questions and inconsistencies in the conventional literature which merit investigation from a creation science perspective. Most of these are related to time scales, viz. the longevity and integrity of biomolecules in ocean floor sediments over multi-million year time scales, the rates of carbonate precipitation in deep-sea sediment, and solid state carbon-oxygen bond reordering over multi-million year time scales. The puzzling apparent non-relationship between temperature and ice volume through the Cenozoic noted by Cramer et al. (2011) also merits a creation science investigation; this may prove of particular interest for models of the post-Flood period.
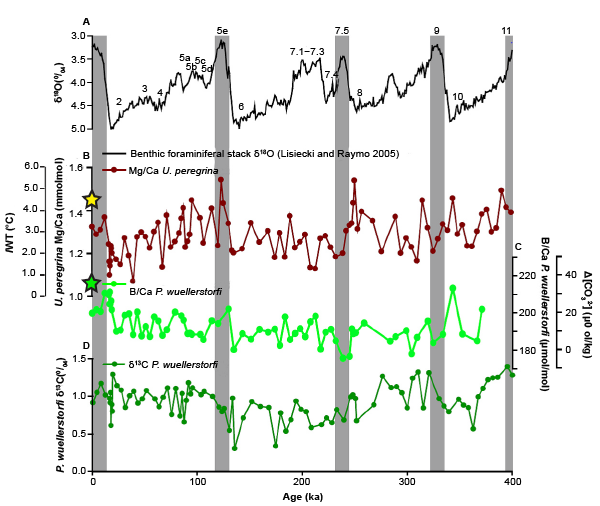
Fig. 19. Compilation of Antarctic Intermediate Water proxy records for core DSDP593 (40º30´S, 167º41´E, 1068m water depth), taken from fig.4 in Elmore et al. (2015). Plots are: (A) Benthic foraminiferal δ18O from the LR04 stack (Lisiecki and Raymo 2005); (B) IWT (Intermediate Water Temperature) plot based on Uvigerina peregrina Mg/Ca data (Elderfield et al. 2010); (C) Δ[CO32-] (units μmol/kg), based on Planulina wuellerstorfi B/Ca ratios; (D) P. wuellerstorfi δ13C. Note that U. peregrina is an infaunal species and P. wuellerstorfi an epifaunal species (see Nomenclature for definitions).
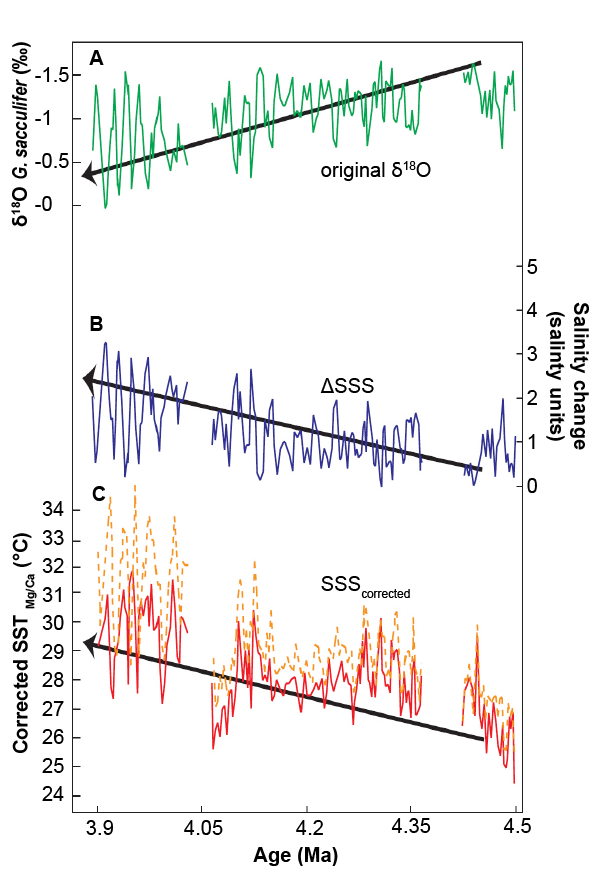
Fig. 20. Globigerinoides sacculifer Mg/Ca record for ODP Site 1000 (16º33´N, 79º52´W, 916 m water depth; see Steph et al. 2006) adjusted to account for the influence of salinity through the time interval 4.5–3.9 Ma BP in the conventional chronology. Taken from fig. 8 in Groeneveld et al. (2008). Arrows indicate trends resulting from the restriction of upper ocean water masses between the Pacific and the Caribbean since 4.5 Ma. Plots: (A) Original G. sacculifer δ18O record for Site 1000 (Steph et al. 2006); (B) Salinity change calculated using the rule Δ1‰ δ18O = Δ2 salinity units (Broecker 1989); (C) SSTMg/Ca record adjusted for salinity using the equivalence Δ1 salinity unit = Δ7% Mg/Ca (Nürnberg, Bijma, and Hemleben 1996); dotted line shows the original, uncorrected SSTMg/Ca record.
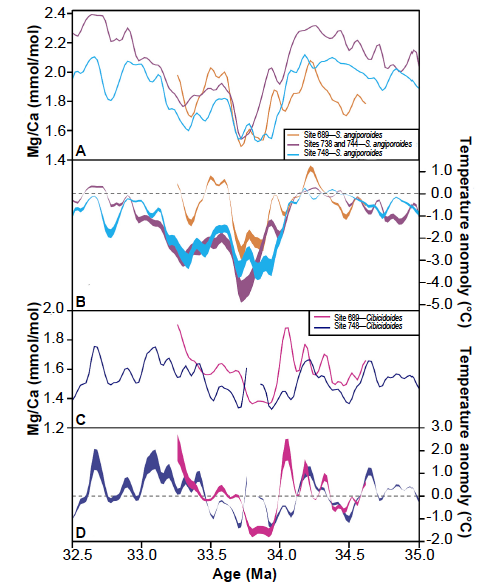
Fig. 21. Compilation of filtered Mg/Ca records for Southern Ocean sites through the EOT (Eocene-Oligocene Transition), 35–32.5 Ma BP in the conventional chronology. Taken from fig. 4 in Bohaty, Zachos, and Delaney (2012). Plots: (A) Mg/Ca records for planktonic foraminifer Subbotina angiporoides from ODP Sites 689 (orange line), 748 (light blue line), and 738 and 744 (purple line); (B) Temperature anomaly, defined as the relative temperature change with respect to the baseline average for 34.5–34.2 Ma and determined from S. angiporoides Mg/Ca data using a temperature sensitivity (exponential constant, cf. equation 1) between 0.08 and 0.1; (C) Mg/Ca records for benthic foraminifer Cibicidoides praemundulus from Sites 689 (pink line) and 748 (dark blue line); (D) Temperature anomaly, the relative temperature change with respect to the baseline average for 34.5–34.2 Ma and determined from Cibicidoides Mg/Ca data using a temperature sensitivity between 0.109 and 0.12. Site coordinates and water depths are: 689 (64.5ºS, 3.1ºE, 2080 m), 748 (58.4ºS, 79.0ºE, 1291 m), 738 (62.7ºS, 82.8ºE, 2253 m) and 744 (61.6ºS, 80.6ºE, 2307 m).
Fig. 22. Site map of ODP Leg 207, located on the Demerara Rise, Suriname, Western Equatorial Atlantic. The Cretaceous black shales investigated by Bice et al. (2006) were sampled from ODP Sites 1257 (water depth 2951 m), 1258 (3192 m) and 1260 (2548 m). Downloaded from http://www-odp.tamu.edu/publications/207_IR/map.htm. Parent page is entitled “Volume 207 Initial Reports (Proceedings of the Ocean Drilling Program)” at http://www-odp.tamu. edu/publications/207_IR/207ir.htm.
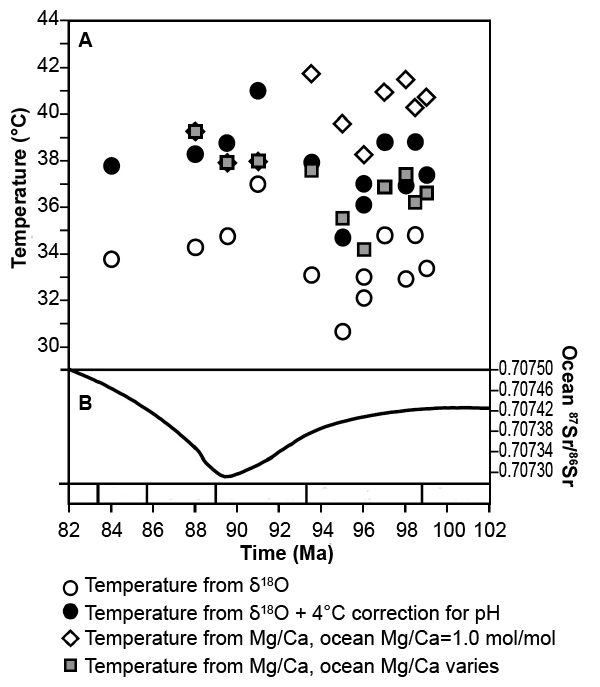
Fig. 23. (A) Cretaceous upper ocean temperature estimates from planktonic foraminiferal δ18O and Mg/Ca in the Demerara Rise black shales studied by Bice et al. (2006), who consider estimates indicated by solid circles and shaded squares to be more plausible than their open equivalents; (B) ocean 87Sr/86Sr ratios, included as an indicator of hydrothermal seawater exchange and continental weathering rate, the minimum possibly indicating a minimum in seawater Mg/Ca. The horizontal axis indicates conventional age. Taken from fig. 1 in Bice et al. (2006), which should be consulted for further details.
Acknowledgments
Paul Garner (Biblical Creation Trust) provided access to many of the references. I wish also to thank the anonymous reviewers of this paper for their suggestions, which I believe have enhanced its value.
Alibert, Chantal, and Malcolm T. McCulloch. 1997. “Strontium/Calcium Ratios In Modern Porites Corals From The Great Barrier Reef As a proxy For Sea Surface Temperature: Calibration Of the Thermometer and Monitoring of ENSO.” Paleoceanography and Paleoclimatology 12, no. 3 (June): 345–363. doi.org/10.1029/97PA00318.
Alpert, Alice E., Anne L. Cohen, Delia W. Oppo, Thomas M. DeCarlo, Jamison M. Gove, and Charles W. Young. 2016. “Comparison Of Equatorial Pacific Sea Surface Temperature Variability And Trends With Sr/Ca Records From Multiple Corals.” Paleoceanography and Paleoclimatology 31, no. 2 (February): 252–265. doi:10.1002/2015PA002897.
Anand, Pallavi, Henry Elderfield, and Maureen H. Conte 2003. “Calibration Of Mg/Ca Thermometry In Planktonic Foraminifera From a Sediment Trap Time Series.” Paleoceanography and Paleoclimatology 18, no. 2 (June): 28-1–28-15. doi.org/10.1029/2002PA000846.
Austin, Steven A., John R. Baumgardner, D. Russell Humphreys, Andrew A. Snelling, Larry Vardiman, and Kurt P. Wise. 1994. “Catastrophic Plate Tectonics: A Global Flood Model Of earth History.” In Proceedings of the Third International Conference on Creationism, edited by R. E. Walsh, 609–621. Pittsburgh, Pennsylvania: Creation Science Fellowship.
Balzano, Sergio, Julie Lattaud, Laura Villanueva, Sebastiaan W. Rampen, Corina P. D. Brussaard, Judith van Bleijswijk, Nicole Bale, Jaap S. Sinninghe Damsté, and Stefan Schouten. 2018. “A Quest For the Biological Sources Of Long Chain Alkyl Diols In the Western Tropical North Atlantic Ocean.” Biogeosciences 15: 5951–5968. doi: 10.5194/bg-15-5951-2018.
Barker, S., M. Greaves, and H. Elderfield. 2003. “A Study Of Cleaning Procedures Used For Foraminiferal Mg/Ca Paleothermometry.” Geochemistry, Geophysics, Geosystems 4, no. 9 (September). doi: 10.1029/2003GC000559.
Barker, Stephen, Isabel Cacho, Heather Benway, and Kazuyo Tachikawa. 2005. “Planktonic Foraminiferal Mg/Ca As a Proxy For Past Oceanic Temperatures: A Methodological Overview And Data Compilation For The Last Glacial Maximum.” Quaternary Science Reviews 24, nos. 7–9 (April): 821–834.
Barrientos, Natalia, Caroline H. Lear, Martin Jakobsson, Christian Stranne, Matt O’Regan, Thomas M. Cronin, Alexandr Y. Gukov, and Helen K. Coxall. 2018. “Arctic Ocean Benthic Foraminifera Mg/Ca Ratios And Global Mg/Ca-Temperature Calibrations: New Constraints At Low Temperatures.” Geochimica et Cosmochimica Acta 236 (September): 240–259.
Bauch, D., K. Darling, J. Simstich, H. A. Bauch, H. Erlenkeuser, and D. Kroon. 2003. “Palaeoceanographic Implications Of Genetic Variation In Living North Atlantic Neogloboquadrina pachyderma.” Nature 424 (17 July): 299–302.
Beck, J. Warren, R. Lawrence Edwards, Emi Ito, Frderick W. Taylor, Jacques Recy, Francis Rougerie, Pascale Joannot, and Christian Henin. 1992. “Sea-Surface Temperature From Coral Skeletal Strontium/Calcium Ratios.” Science 257, no. 5070 (July 31): 644–647.
Becker, Kevin W., Julius S. Lipp, Gerard J. M. Versteegh, Lars Wörmer, and Kai-Uwe Hinrichs. 2015. “Rapid And Simultaneous Analysis Of Three Molecular Sea Surface Temperature Proxies And Application To Sediments From the Sea Of Marmara.” Organic Geochemistry 85 (August): 42–53.
Bell, David B., Simon J. A. Jung, Dick Kroon, David A. Hodell, Lucas J. Lourens, and Maureen E. Raymo. 2015. “Atlantic Deep-Water Response To the Early Pliocene Shoaling Of the Central American Seaway.” Scientific Reports 5: 12252. doi: 10.1038/srep12252.
Berger, W. H., M.-C. Bonneau, and F. L. Parker. 1982. “Foraminifera On the Deep-Sea Floor: Lysocline And Dissolution Rate.” Oceanologica Acta 5, no. 2: 249–258.
Bergmann, Kristin D., Seth Finnegan, Roger Creel, John M. Eiler, Nigel C. Hughes, Leonid E. Popov, and Woodward W. Fischer. 2018. “A Paired Apatite And Calcite Clumped Isotope Thermometry Approach To Estimating Cambro-Ordovician Seawater Temperatures And Isotopic Composition.” Geochimica et Cosmochimica Acta 224 (1 March): 18–41.
Bernasconi, Stefano M., Inigo A. Müller, Kristin D. Bergmann, Sebastian F. M. Breitenbach, Alvaro Fernandez, David A. Hodell, Madalina Jaggi, Anna Nele Meckler, Isabel Millan, and Martin Ziegler. 2018. “Reducing Uncertainties In Carbonate Clumped Isotope Analysis Through Consistent Carbonate-Based Standardization.” Geochemistry, Geophysics, Geosystems 19, no. 9 (September): 2895–2914. doi: 10.1029/2017GC007385.
Bice, Karen L., Daniel Birgel, Philip A. Meyers, Kristina A. Dahl, Kai-Uwe Hinrichs, and Richard D. Norris. 2006. “A Multiple Proxy And Model Study Of Cretaceous Upper Ocean Temperatures And Atmospheric CO2 Concentrations.” Paleoceanography and Paleoclimatology 21, no. 2 PA2002. doi: 10.1029/2005PA001203.
Billups, K., and D. P. Schrag. 2002. “Paleotemperatures And Ice Volume Of the Past 27 Myr Revisited With Paired Mg/Ca and 18O/16O Measurements On Benthic Foraminifera.” Paleoceanography and Paleoclimatology 17, no. 1 (February): 3-1–3-11. doi.org/10.1029/2000PA000567.
Blackmon, Paul D., and Ruth Todd. 1959. “Mineralogy Of Some Foraminifera As Related To Their Classification And Ecology.” Journal of Paleontology 33, no. 1 (January): 1–15.
Bohaty, Steven M., James C. Zachos, and Margaret L. Delaney. 2012. “Foraminiferal Mg/Ca Evidence For Southern Ocean Cooling Across the Eocene-Oligocene Transition.” Earth and Planetary Science Letters 317–318 (1 February): 251– 261.
Bonfardeci, Alessandro, Antonio Caruso, Annachiara Bartolini, Franck Bassinot, and Marie-Madeleine Blanc-Valleron. 2018. “Distribution And Ecology Of the Globigerinoides Ruber—Globigerinoides Elongatus Morphotypes In The Azores Region During the late Pleistocene-Holocene.” Palaeogeography, Palaeoclimatology, Palaeoecology 491 (1 February): 92–111. doi: 10.1016/j.palaeo.2017.11.052.
Boon, Jaap J., F. W. v.d. Meer, P. J. W. Schuyl, J. W. de Leeuw, P. A. Schenck and A.L. Burlingame. 1978. “Organic Geochemical Analyses Of Core Samples From Site 362, Walvis Ridge, DSDP Leg 40.” Initial Reports. DSDP Legs 38, 39, 40, and 41, Supplement, 627–637.
Boussetta, S., F. Bassinot, A. Sabbatini, N. Caillon, J. Nouet, N. Kallel, H. Rebaubier, G. Klinkhammer, and L. Labeyrie. 2011. “Diagenetic Mg-Rich Calcite In Mediterranean Sediments: Quantification And Impact On Mg/Ca Thermometry.” Marine Geology 280 (nos. 1–4): 195–204.
Brand, Willi A., Sergey S. Assonov, and Tyler B. Coplen. 2010. “Correction For The 17O Interference In δ(13C) Measurements When Analyzing CO2 With Stable Isotope Mass Spectrometry (IUPAC Technical Report).” Pure and Applied Chemistry 82, no. 8 (26 May): 1719–1733.
Branson, Oscar, Simon A. T. Redfern, Aurora C. Elmore, Elizabeth Read, Sergio Valencia, and Henry Elderfield. 2018. “The Distribution And Coordination Of Trace Elements In Krithe Ostracods And Their Implications For Paleothermometry.” Geochimica et Cosmochimica Acta 236 (1 September): 230–239.
Branson, Oscar, Simon A. T. Redfern, Tolek Tyliszczak, Aleksey Sadekov, Gerald Langer, Katsunori Kimoto, and Henry Elderfield. 2013. “The Coordination Of Mg In Foraminiferal Calcite.” Earth and Planetary Science Letters 383 (1 December): 134–141.
Brassell, S. C., G. Eglinton, I. T. Marlowe, U. Pflaumann, and M. Sarnthein. 1986. “Molecular Stratigraphy: A New Tool For Climatic Assessment.” Nature 320 (13 March): 129–133.
Brassell, Simon C., Mirela Dumitrescu, and the ODP Leg 198 Shipboard Scientific Party. 2004. “Recognition Of Alkenones In A Lower Aptian Porcellanite From The West-Central Pacific.” Organic Geochemistry 35, no. 2 (February): 181–188.
Brassell, Simon C. 2014. “Climatic Influences On The Paleogene Evolution Of Alkenones.” Paleoceanography and Paleoclimatology 29, no. 3: 255–272. doi: 10.1002/2013PA002576.
Breitenbach, Sebastian F. M., Maryline J. Mlenek-Vautravers, Anna-Lena Grauel, Li Lo, Stefano M. Bernasconi, Inigo A. Müller, James Rolfe, Fernando Gázquez, Mervyn Greaves, and David A. Hodell. 2018. “Coupled Mg/Ca And Clumped Isotope Analyses Of Foraminifera Provide Consistent Water Temperatures.” Geochimica et Cosmochimica Acta 236 (1 September): 283–296.
Broecker, W. S. 1989. “The Salinity Contrast Between the Atlantic And Pacific Oceans During Glacial Time.” Paleoceanography and Paleoclimatology 4, no. 2: 207–212. doi: 10.1029/PA004i002p00207.
Brown, Sarah J., and Henry Elderfield. 1996. “Variations In Mg/Ca And Sr/Ca Ratios Of Planktonic Foraminifera Caused By Postdepositional Dissolution: Evidence Of Shallow Mg-Dependent Dissolution.” Paleoceanography and Paleoclimatology 11, no. 5 (October): 543–551. doi.org/10.1029/96PA01491.
Bryan, Sean P., and Thomas M. Marchitto. 2008. “Mg/Ca-Temperature Proxy In Benthic Foraminifera: New Calibrations From The Florida Straits And a Hypothesis Regarding Mg/Li.” Paleoceanography and Paleoclimatology 23, no. 2, PA2220. doi: 10.1029/2007PA001553.
Burton, Elizabeth A., and Lynn M. Walter. 1987. “Relative Precipitation Rates Of Aragonite And Mg Calcite From Seawater: Temperature Or Carbonate Ion Control?” Geology 15, no. 2: 111–114.
Butler, Scott, Trevor R. Bailey, Caroline H. Lear, Gordon B. Curry, Lesley Cherns, and Iain McDonald. 2015. “The Mg/Ca-Temperature Relationship In Brachiopod Shells: Calibrating A Potential Palaeoseasonality Proxy.” Chemical Geology 397 (18 March): 106–117.
Came, Rosemarie E., Uwe Brand, and Hagit P. Affek. 2014. “Clumped Isotope Signatures In Modern Brachiopod Carbonate.” Chemical Geology 377 (4 June): 20–30.
Carbonate Research@Imperial College. 2015. What are clumped isotopes? http://www.carbonateresearch.com/clumped_isotope.
Carlson, Anders E., Peter U. Clark, Brian A. Haley, Gary P. Klinkhammer, Kathleen Simmons, Edward J. Brook, and Katrin J. Meissner. 2007. Geochemical Proxies Of North American Freshwater Routing During The Younger Dryas Cold Event.” Proceedings of the National Academy of Sciences of the United States of America 104, no. 16: 6556– 6561.
Chave, Keith E. 1954. “Aspects Of the Biogeochemistry Of Magnesium. 1. Calcareous Marine Organisms.” Journal of Geology 62, no. 3 (May): 266–283.
Chilingar, George V. 1962. “Dependence On Temperature Of Ca/Mg Ratio Of Skeletal Structures Of Organisms And Direct Chemical Precipitates Out Of Seawater.” Bulletin of the Southern California Academy of Sciences 61, no. 1: 45–60.
Clarke, Frank Wigglesworth, and Walter Calhoun Wheeler. 1922. “The Inorganic Constituents Of Marine Invertebrates.” USGS Professional Paper 124, 55, 61. https://pubs.usgs.gov/pp/0124/report.pdf.
Cohen, Anne L., Graham D. Layne, Stanley R. Hart, and Phillip S. Lobel. 2001. “Kinetic Control Of Skeletal Sr/Ca In A Symbiotic Coral: Implications For The Paleotemperature Proxy.” Paleoceanography and Paleoclimatology 16, no. 1 (February): 20–26. doi.org/10.1029/1999PA000478.
Cohen, Anne L., Kathryn E. Owens, Graham D. Layne, and Nobumichi Shimizu. 2002. “The Effect Of Algal Symbionts On The Accuracy Of Sr/Ca Paleotemperatures From Coral.” Science 296, no. 5566 (12 April): 331–333.
Conte, Maureen H., Marie-Alexandrine Sicre, Carsten Rühlemann, John C. Weber, Sonja Schulte, Detlef Schulz-Bull, and Thomas Blanz. 2006. “Global Temperature Calibration Of The Alkenone Unsaturation Index (UK’37) In Surface Waters And Comparison With Surface Sediments.” Geochemistry, Geophysics, Geosystems 7, no. 2 (February): 1–22.
Cramer, B. S., K. G. Miller, P. J. Barrett, and J. D. Wright. 2011. “Late Cretaceous-Neogene Trends In Deep Ocean Temperature And Continental Ice Volume: Reconciling Records Of Benthic Foraminiferal Geochemistry (δ18O And Mg/Ca) With Sea Level History.” Journal of Geophysical Research 116, no. C12. doi: 10.1029/2011JC007255.
Curry, W. B., and T. M. Marchitto. 2008. “A Secondary Ionization Mass Spectrometry Calibration Of Cibicidoides pachyderma Mg/Ca With Temperature.” Geochemistry, Geophysics, Geosystems 9, no. 4 (April): 1–13.
Cusack, Maggie, Alberto Pérez-Huerta, Markus Janousch, and Adrian A. Finch. 2008. “Magnesium In the Lattice Of Calcite-Shelled Brachiopods.” Chemical Geology 257, nos. 1–2 (30 November): 59–64.
Daëron, M., D. Blamart, M. Peral, and H. P. Affek. 2016. “Absolute Isotopic Abundance Ratios And the Accuracy Of Δ47 Measurements.” Chemical Geology 442 (28 November): 83–96.
Damsté, Jaap S., Stefan Schouten, Ellen C. Hopmans, Adri C. T. van Duin, and Jan A. J. Geenavasen. 2002. “Crenarchaeol: The Characteristic Core Glycerol Dibiphytanyl Glycerol Tetraether Membrane Lipid Of Cosmopolitan Pelagic Crenarchaeota.” Journal of Lipid Research 43: 1641–1651.
Darling, Kate F., Michal Kucera, Dick Kroon, and Christopher M. Wade. 2006. “A Resolution For the Coiling Direction Paradox In Neogloboquadrina pachyderma.” Paleoceanography and Paleoclimatology 21, no. 2 (June) PA2011. doi: 10.1029/2005PA001189.
Davies, Amelia J., and Cédric M. John. 2017. “Reducing Contamination Parameters For Clumped Isotope Analysis: The Effect Of Lowering Porapak™ Q Trap Temperature To Below –50°C.” Rapid Communications in Mass Spectrometry 31, no. 16 (August 30): 1313–1323.
Davis, Catherine V., Tessa M. Hill, Ann D. Russell, Brian Gaylord, and Jaime Jahncke. 2016. “Seasonality In Planktic Foraminifera Of the Central California Coastal Upwelling Region.” Biogeosciences 13, no. 18 (16 September): 5139–5150. doi: 10.5194/bg-13-5139-2016.
Dawber, C. F., and A. Tripati. 2012. “Relationships Between Bottom Water Carbonate Saturation And Element/Ca Ratios In Coretop Samples Of The Benthic Foraminifera Oridorsalis umbonatus.” Biogeosciences 9, no. 8 (9 August): 3029–3045. doi: 10.5194/bg-9-3029-2012.
Dekens, Petra S., David W. Lea, Dorothy K. Pak, and Howard J. Spero. 2002. “Core Top Calibration Of Mg/Ca In Tropical Foraminifera: Refining Paleotemperature Estimation.” Geochemistry, Geophysics, Geosystems 3, no. 4 (April): 1–29.
Dekens, Petra S., Ana Christina Ravelo, Matthew D. McCarthy, and Christopher A. Edwards. 2008. “A 5 Million Year Comparison Of Mg/Ca And Alkenone Paleothermometers.” Geochemistry, Geophysics, Geosystems 9, no. 10 (October), Q10001: 1–18. doi: 10.1029/2007GC001931.
De Laeter, John R., John Karl Böhlke, P. De Bièvre, H. Hidaka, H. S. Peiser, K. J. R. Rosman, and P. D. P. Taylor. 2003. “Atomic Weights Of The Elements. Review 2000 (IUPAC Technical Report).” Pure and Applied Chemistry 75, no. 6: 683–800.
DeLong, Kristine L., Jennifer A. Flannery, Richard Z. Poore, Terrence M. Quinn, Christopher R. Maupin, Ke Lin, and Chuan-Chou Shen. 2014. “A Reconstruction Of Sea Surface Temperature Variability In the Southeastern Gulf Of Mexico From 1734 to 2008 C.E. Using Cross-Dated Sr/Ca Records From The Coral Siderastrea siderea.” Paleoceanography and Paleoclimatology 29, no. 5 (May): 403–422. doi: 10.1002/2013PA002524.
De Nooijer, L. J., H. J. Spero, J. Erez, J. Bijma, and G. J. Reichart. 2014. “Biomineralization In Perforate Foraminifera.” Earth-Science Reviews 135 (August): 48–58.
Dueñas-Bohórquez, Adriana, Régine Elisabeth da Rocha, Azumi Kuroyanagi, Jelle Bijma, and Gert-Jan Reichart. 2009. “Effect Of Salinity And Seawater Calcite Saturation State On Mg And Sr Incorporation In Cultured Planktonic Foraminifera.” Marine Micropaleontology 73, nos. 3–4 (December): 178–189.
Dueñas-Bohórquez, Adriana, Régine Elisabeth da Rocha, Azumi Kuroyanagi, Lennart J. de Nooijer, Jelle Bijma and Gert-Jan Reichart. 2011. “Interindividual Variability And Ontogenetic Effects On Mg And Sr Incorporation In the Planktonic Foraminifer Globigerinoides sacculifer.” Geochimica et Cosmochimica Acta 75, no. 2 (15 January): 520–532.
Dwyer, Gary S., Thomas M. Cronin, Paul A. Baker, Maureen E. Raymo, Jeffrey S. Buzas, and Thierry Corrège. 1995. “North Atlantic Deepwater Temperature Change During Late Pliocene And Late Quaternary Climatic Cycles.” Science 270, no. 5240 (24 November): 1347–1351.
Eagle, Robert A., Marcus Enriquez, Gerald Grellet-Tinner, Alberto Pérez-Huerta, David Hu, Thomas Tütken, Shaena Montanari et al. 2015. “Isotopic Ordering In Eggshells Reflects Body Temperatures And Suggests Differing Thermophysiology In Two Cretaceous Dinosaurs.” Nature Communications 6: 8296.
Edgar, Kirsty M., Eleni Anagnostou, Paul N. Pearson, and Gavin L. Foster. 2015. “Assessing the Impact Of Diagenesis On δ11B, δ13C, δ18O, Sr/Ca And B/Ca Values In Fossil Planktic Foraminiferal Calcite.” Geochimica et Cosmochimica Acta 166 (1 October): 189–209.
Eggins, Stephen M., Aleksey Sadekov, and Patrick De Deckker. 2004. “Modulation And Daily Banding Of Mg/Ca in Orbulina Universa Tests By Symbiont Photosynthesis And Respiration: A Complication For Seawater Thermometry?” Earth and Planetary Science Letters 225, nos. 3–4: 411–419.
Eglinton, Timothy I., and Geoffrey Eglinton. 2008. “Molecular Proxies For Paleoclimatology.” Earth and Planetary Science Letters 275, nos. 1–2 (30 October): 1–16.
Elderfield, H., and G. Ganssen. 2000. “Past Temperature And δ18O Of Surface Ocean Waters Inferred From Foraminiferal Mg/Ca Ratios.” Nature 405 (25 May): 442–445.
Elderfield, H., M. Vautravers, and M. Cooper. 2002. “The Relationship Between Shell Size And Mg/Ca, Sr/Ca, δ18O And δ13C Of Species Of Planktonic Foraminifera.” Geochemistry, Geophysics, Geosystems 3, no. 8. doi: 10.1029/2001GC000194.
Elderfield, H., M. Greaves, S. Barker, I. R. Hall, A. Tripati, P. Ferretti, S. Crowhurst, L. Booth, and C. Daunt. 2010. “A Record Of Bottom Water Temperature And Seawater δ18O For The Southern Ocean Over The Past 440 Kyr Based On Mg/Ca Of Benthic Foraminiferal Uvigerina spp.” Quaternary Science Reviews 29: 160–169.
Elmore, A. C., S. Sosdian, Y. Rosenthal, and J. D. Wright. 2012. “A Global Evaluation Of Temperature And Carbonate Ion Control On Mg/Ca Ratios Of Ostracoda Genus Krithe.” Geochemistry, Geophysics, Geosystems 13, no. 9: Q09003. doi: 10.1029/2012GC004073.
Elmore, Aurora C., Erin L. McClymont, Henry Elderfield, Sev Kender, Michael R. Cook, Melanie J. Leng, Mervyn Greaves, and Sambuddha Misra. 2015. “Antarctic Intermediate Water Properties Since 400 Ka Recorded In Infaunal (Uvigerina peregrina) And Epifaunal (Planulina wuellerstorfi) Benthic Foraminifera.” Earth and Planetary Science Letters 428 (15 October): 193–203.
Erez, Jonathan. 2003. “The Source Of Ions For Biomineralization In Foraminifera And Their Implications For Paleoceanographic Proxies.” Reviews in Mineralogy and Geochemistry 54, no. 1 (3 January): 115–149.
Evans, David., and Wolfgang Müller. 2012. “Deep Time Foraminifera Mg/Ca Paleothermometry: Nonlinear Correction For Secular Change In Seawater Mg/Ca.” Paleoceanography and Paleoclimatology 27, no. 4 (December): PA4205. doi: 10.1029/2012PA002315.
Evans, David, Bridget S. Wade, Michael Henehan, Jonathan Erez, and Wolfgang Müller. 2016. “Revisiting Carbonate Chemistry Controls On Planktic Foraminifera Mg/Ca: Implications For Sea Surface Temperature And Hydrology Shifts Over the Paleocene-Eocene Thermal Maximum and Eocene-Oligocene Transition.” Climate of the Past 12: 819–835.
Fantle, Matthew S., and Donald J. DePaolo. 2006. “Sr Isotopes And Pore Fluid Chemistry In Carbonate Sediment Of the Ontong Java Plateau: Calcite Recrystallization Rates And Evidence For A Rapid Rise In Seawater Mg Over The Last 10 Million Years.” Geochimica et Cosmochimica Acta 70, no. 15 (1 August): 3883–3904.
Fantle, Matthew S. and Donald J. DePaolo. 2007. “Ca Isotopes In Carbonate Sediment And Pore Fluid From ODP Site 807A: The Ca2+(aq)-Calcite Equilibrium Fractionation Factor And Calcite Recrystallization Rates In Pleistocene Sediments.” Geochimica et Cosmochimica Acta 71, no. 10 (15 May): 2524–2546.
Farmer, Jesse R., Thomas M. Cronin, and Gary S. Dwyer. 2012. “Ostracode Mg/Ca Paleothermometry In The North Atlantic And Arctic Oceans: Evaluation Of A Carbonate Ion Effect.” Paleothermometry and Paleoclimatology 27, no. 2 (June): PA2212. doi: 10.1029/2012PA002305.
Fehrenbacher, Jennifer S., Ann D. Russell, Catherine V. Davis, Alexander C. Gagnon, Howard J. Spero, John B. Cliff, Zihua Zhu, and Pamela Martin. 2017. “Link Between Light-Triggered Mg-Banding And Chamber Formation In The Planktic Foraminifera Neogloboquadrina dutertrei.” Nature Communications 8: 15441 (15 May). doi: 10.1038/ncomms15441.
Felis, Thomas, and Jürgen Pätzold. 2004. “Climate Reconstructions From Annually Banded Corals.” In Global Environmental Change in the Ocean and on Land, edited by M. Shiyomi et al., 205–227.
Ferguson, J. E., G. M. Henderson, M. Kucera, and R. E. M. Rickaby. 2008. “Systematic Change Of Foraminiferal Mg/Ca Ratios Across A Strong Salinity Gradient.” Earth and Planetary Science Letters 265, nos. 1–2 (15 January): 153–166.
Foster, G. L., P. A. E. Pogge von Strandmann, and J. W. B. Rae. 2010. “Boron And Magnesium Isotopic Composition Of Seawater.” Geochemistry, Geophysics, Geosystems 11, no. 8 (August): Q08015. doi: 10.1029/2010GC003201.
Fowell, Sara E., Kate Sandford, Joseph A. Stewart, Karl D. Castillo, Justin B. Ries, and Gavin L. Foster. 2016. “Intrareef Variations In Li/Mg And Sr/Ca Sea Surface Temperature Proxies In The Caribbean Reef-Building Coral Siderastrea siderea.” Paleoceanography and Paleoclimatology 31, no. 10 (October): 1315–1329. doi: 10.1002/2016PA002968.
Gaetani, Glenn A., Anne L. Cohen, Zhengrong Wang, and John Crusius. 2011. “Rayleigh-Based, Multi-Element Coral Thermometry: A Biomineralization Approach To Developing Climate Proxies.” Geochimica et Cosmochimica Acta 75, no. 7: 1920–1932.
Gagan, M. K., L. K. Ayliffe, J. W. Beck, J. E. Cole, E. R. M. Druffel, R. B. Dunbar, and D. P. Schrag. 2000. “New Views Of Tropical Paleoclimates From Corals.” Quaternary Science Reviews 19, nos. 1–5 (1 January): 45–64.
Gagnon, Alexander C., Jess F. Adkins, and Jonathan Erez. 2012. “Seawater Transport During Coral Biomineralization.” Earth and Planetary Science Letters 329–330 (1 May): 150–161.
Geerken, Esmee, Lennart Jan de Nooijer, Inge van Dijk, and Gert-Jan Reichart. 2018. “Impact Of Salinity On Element Incorporation In Two Benthic Foraminiferal Species With Contrasting Magnesium Contents.” Biogeosciences 15, no. 7 (16 April): 2205–2218. doi: 10.5194/bg-15-2205-2018.
Geerken, E., L. J. de Nooijer, A. Roepert, L. Polerecky, H. E. King, and G.-J. Reichart. 2019. “Element Banding And Organic Linings Within Chamber Walls Of Two Benthic Foraminifera.” Scientific Reports 9 (5 March): 3598. doi: 10.1038/s41598-019-40298-y.
Geosciences LibreTexts. 2019. “3.3: The Lysocline And The CCD.” https://geo.libretexts.org/Bookshelves/Book:_Oceanography_(Hill)/03:_Sediments_-_the_Memory_of_the_Ocean/3.3:_The_Lysocline_and_the_CCD.
Ghosh, Prosenjit, Jess Adkins, Hagit Affek, Brian Balta, Weifu Guo, Edwin A. Schauble, Dan Schrag, and John M. Eiler. 2006. “13C–18O Bonds In Carbonate Minerals: A New Kind Of Paleothermometer.” Geochimica et Cosmochimica Acta 70, no. 6 (15 March): 1439–1456.
Gray, William R., Syee Weldeab, David W. Lea, Yair Rosenthal, Nicolas Gruber, Barbara Donner, and Gerhard Fischer. 2018. “The Effects Of Temperature, Salinity, And The Carbonate System On Mg/Ca In Globigerinoides ruber (White): A Global Sediment Trap Calibration.” Earth and Planetary Science Letters 482 (15 January): 607–620.
Groeneveld, Jeroen, Dirk Nürnberg, Ralf Tiedemann, Gert-Jan Reichart, Silke Steph, Lars Reuning, Daniela Crudeli, and Paul Mason. 2008. “Foraminiferal Mg/Ca Increase In the Caribbean During the Pliocene: Western Atlantic Warm Pool Formation, Salinity Influence, Or Diagenetic Overprint?” Geochemistry, Geophysics, Geosystems 9, no. 1 (January): Q01P23. doi: 10.1029/2006GC001564.
Groeneveld, Jeroen, and Cristiano M. Chiessi. 2011. “Mg/Ca Of Globorotalia inflata As A Recorder Of Permanent Thermocline Temperatures In The South Atlantic.” Paleoceanography and Paleoclimatology 26, no. 2 (June): PA2203. doi: 10.1029/2010PA001940.
Grossman, Ethan L. 2012. “Applying Oxygen Isotope Paleothermometry In Deep Time.” In Reconstructing Earth’s Deep-Time Climate, edited by Linda C. Ivany and Brian T Huber, 39–67. The Paleontological Society Papers, Vol. 18.
Grove, Craig A., Sebastian Kasper, Jens Zinke, Miriam Pfeiffer, Dieter Garbe-Schönberg, and Geert-Jan A. Brummer. 2013. “Confounding Effects Of Coral Growth And High SST Variability On Skeletal Sr/Ca: Implications For Coral Paleothermometry.” Geochemistry, Geophysics, Geosystems 14, no. 4 (April): 1277–1293. doi: 10.1002/ggge.20095.
Hathorne, Ed C., Rachael H. James, and Richard S. Lampitt. 2009. “Environmental Versus Biomineralization Controls On The Intratest Variation In The Trace Element Composition Of The Planktonic Foraminifera G. inflata And G. scitula.” Paleoceanography and Paleoclimatology 24, no. 4 (December): PA4204. doi: 10.1029/2009PA001742.
Hathorne, Ed C., Alex Gagnon, Thomas Felis, Jess Adkins, Ryuji Asami, Wim Boer, Nicolas Caillon, et al. 2013a. “Interlaboratory Study For Coral Sr/Ca And Other Element/Ca Ratio Measurements.” Geochemistry, Geophysics, Geosystems 14, no. 9 (September): 3730–3750. doi: 10.1002/ggge.20230.
Hathorne, Ed C., Thomas Felis, Atsushi Suzuki, Hodaka Kawahata, and Guy Cabioch. 2013b. “Lithium In the Aragonite Skeletons Of Massive Porites Corals: A New Tool To Reconstruct Tropical Sea Surface Temperatures.” Paleoceanography and Paleoclimatology 28, no. 1 (March): 143–152. doi: 10.1029/2012PA002311.
Hebert, Jake. 2016a. “Should the “Pacemaker Of the Ice Ages” Paper Be Retracted? Revisiting An Iconic Argument For Milankovitch Climate Forcing, Part 1.” Answers Research Journal 9 (March 9): 25–56. https://answersingenesis.org/environmental-science/ice-age/should-pacemaker-ice-agespaper-be-retracted/.
Hebert, Jake. 2016b. “Revisiting An Iconic Argument For Milankovitch Climate Forcing: Should the “Pacemaker Of the Ice Ages” Paper Be Retracted? Part 2.” Answers Research Journal 9 (May 25): 131–147. https://answersingenesis.org/environmental-science/ice-age/should-pacemaker-ice-agespaper-be-retracted-2/.
Hebert, Jake. 2016c. “Revisiting An Iconic Argument For Milankovitch Climate Forcing: Should The “Pacemaker Of the Ice Ages” Paper Be Retracted? Part 3.” Answers Research Journal 9 (September 14): 229–255. https://answersingenesis.org/environmental-science/ice-age/should-pacemaker-ice-ages-paper-be-retracted-3/.
Henehan, Michael J., Gavin L. Foster, Helen C. Bostock, Rosanna Greenop, Britteny J. Marshall, and Paul A. Wilson. 2016. “A New Boron-Isotope-pH Calibration For Orbulina universa, with Implications For Understanding And Accounting For ‘Vital Effects’.” Earth and Planetary Science Letters 454 (15 November): 282–292.
Henkes, Gregory A., Benjamin H. Passey, Ethan L. Grossman, Brock J. Shenton, Alberto Pérez-Huerta, and Thomas E. Yancey. 2014. “Temperature Limits For Preservation Of Primary Calcite Clumped Isotope Paleotemperatures.” Geochimica et Cosmochimica Acta 139 (15 August): 362–382.
Herbert, T. D. 2001. “Review Of Alkenone Calibrations (Culture, Water Column, And Sediments).” Geochemistry, Geophysics, Geosystems 2, no. 2 (February5). Paper number 2000GC000055. https://doi.org/10.1029/2000GC000055.
Herbert, T. D. 2003. “Alkenone Paleotemperature Determinations.” In Treatise on Geochemistry, edited by Heinrich D. Holland and Karl K. Turekian, Vol. 8.15: 391–432
Hodell, David A., James E. T. Channell, Jason H. Curtis, Oscar E. Romero, and Ursula Röhl. 2008. “Onset Of “Hudson Strait” Heinrich Events In the Eastern North Atlantic At the End Of the Middle Pleistocene Transition (~640 ka)?” Paleoceanography and Paleoclimatology 23, no. 4 (December): PA4218. doi: 10.1029/2008PA001591.
Hönisch, Bärbel, Katherine A. Allen, David W. Lea, Howard J. Spero, Stephen M. Eggins, Jennifer Arbuszewski, Peter deMenocal, Yair Rosenthal, Ann D. Russell, and Henry Elderfield. 2013. “The Influence Of Salinity On Mg/Ca In Planktic Foraminifers—Evidence From Cultures, Core-Top Sediments And Complementary δ18O.” Geochimica et Cosmochimica Acta 121 (15 November): 196–213.
Hoogakker, Babette A. A., Gary P. Klinkhammer, Harry Elderfield, Eelco J. Rohling, and Chris Hayward. 2009. “Mg/Ca Paleothermometry In High Salinity Environments.” Earth and Planetary Science Letters 284, nos. 3–4 (15 July): 583–589.
Huang, Kuo-Fang, Cheng-Feng You, Hui-Ling Lin, and Ying-Tzeng Shieh. 2008. “In Situ Calibration Of Mg/Ca Ratio In Planktonic Foraminiferal Shell Using Time Series Sediment Trap: A Case Study Of Intense Dissolution Artifact In the South China Sea.” Geochemistry, Geophysics, Geosystems 9, no. 4: Q04016. doi: 10.1029/2007GC001660.
Huguet, Carme, Arndt Schimmelmann, Robert Thunell, Lucas J. Lourens, Jaap S. Sinninghe Damsté, and Stefan Schouten. 2007. “A Study Of the TEX86 Paleothermometer In the Water Column And Sediments Of the Santa Barbara Basin, California.” Paleoceanography and Paleoclimatology 22, no. 3: PA3203. doi:10.1029/2006PA001310.
IODP (International Ocean Discovery Program). 2017. Exploring the Earth under the Sea. http://www.iodp.org/.
Irvali, N., U.S. Ninnemann, E.V. Galaasen, Y. Rosenthal, D. Kroon, D.W. Oppo, H.F. Kleiven, K.F. Darling and C. Kissel. 2012. “Rapid Switches In Subpolar North Atlantic Hydrography And Climate During The Last Interglacial (MIS 5e).” Paleoceanography and Paleoclimatology 27, no. 2: PA 2207. doi: 10.1029/2011PA002244.
Irvali, Nil, Ulysses S. Ninnemann, Helga (Kikki) F. Kleiven, Eirik V. Galaasen, Audrey Morley, and Yair Rosenthal. 2016. “Evidence For Regional Cooling, Frontal Advances, And East Greenland Ice Sheet Changes During The Demise Of The Last Interglacial.” Quaternary Science Reviews 150 (15 October): 184–199.
Jenkyns, H. C., L. Schouten-Huibers, S. Schouten, and J. S. Sinninghe Damsté. 2012. “Warm Middle Jurassic–Early Cretaceous High-Latitude Sea-Surface Temperatures From The Southern Ocean.” Climate of the Past 8, no. 1 (2 February): 215–226.
Jentzen, Anna, Dirk Nürnberg, Ed C. Hathorne, and Joachim Schönfeld. 2018. “Mg/Ca And δ18O In Living Planktic Foraminifers From The Caribbean, Gulf of Mexico And Florida Straits.” Biogeosciences 15, no. 23: 7077–7095. doi: 10.5194/bg-15-7077-2018
Jonkers, L., C. E. Reynolds, J. Richey, and I. R. Hall. 2015. “Lunar Periodicity In The Shell Flux Of Planktonic Foraminifera In the Gulf of Mexico.” Biogeosciences 12, no. 10: 3061–3070. doi: 10.5194/bg-12-3061-2015.
Jonkers, Lukas, Ben Buse, Geert-Jan A. Brummer, and Ian Robert Hall. 2016. “Chamber Formation Leads To Mg/Ca-Banding In The Planktonic Foraminifer Neogloboquadrina pachyderma.” Earth and Planetary Science Letters 451: 177–184.
Katz, Amitai. 1973. “The Interaction Of Magnesium With Calcite During Crystal Growth At 25–90°C And One Atmosphere.” Geochimica et Cosmochimica Acta 37, no. 6 (June): 1563–1578.
Katz, Miriam E., Benjamin S. Cramer, Allison Franzese, Bärbel Hönisch, Kenneth G. Miller, Yair Rosenthal, and James D. Wright. 2010. “Traditional And Emerging Geochemical Proxies In Foraminifera.” Journal of Foraminiferal Research 40, no. 2 (April 1): 165–192.
Kelson, Julia R., Katharine W. Huntington, Andrew J. Schauer, Casey Saenger, and Alex R. Lechler. 2017. “Toward A Universal Carbonate Clumped Isotope Calibration: Diverse Synthesis And Preparatory Methods Suggest A Single Temperature Relationship.” Geochimica et Cosmochimica Acta 197 (15 January): 104–131.
Khider, D., G. Huerta, C. Jackson, L.D. Stott and J. Emile-Geay. 2015. “A Bayesian, Multivariate Calibration For Globigerinoides ruber Mg/Ca.” Geochemistry, Geophysics, Geosystems 16, no. 9 (September 2015): 2916–2932. doi: 10.1002/2015GC005844.
Kim, Jung-Hyun, Xavier Crosta, Elisabeth Michel, Stefan Schouten, Josette Duprat, and Jaap S. Sinninghe Damsté. 2009. “Impact Of Lateral Transport On Organic Proxies In The Southern Ocean.” Quaternary Research 71, no. 2 (March): 246–250.
Kim, Jung-Hyun, Jaap van der Meer, Stefan Schouten, Peer Helmke, Veronica Willmott, Francesca Sangiorgi, Nalân Koç, Ellen C. Hopmans, and Jaap S. Sinninghe Damsté. 2010. “New Indices And Calibrations Derived From The Distribution Of Crenarchaeal Isoprenoid Tetraether Lipids: Implications For Past Sea Surface Temperature Reconstructions.” Geochimica et Cosmochimica Acta 74, no. 16: 4639–4654.
Kim, Sang-Tae, and James R. O’Neil. 1997. “Equilibrium And Nonequilibrium Oxygen Isotope Effects In Synthetic Carbonates.” Geochimica et Cosmochimica Acta 61, no. 16 (August): 3461–3475.
Kisakürek, B., A. Eisenhauer, F. Böhm, D. Garbe-Schönberg, and J. Erez. 2008. “Controls On Shell Mg/Ca And Sr/Ca In Cultured Planktonic Foraminiferan, Globigerinoides ruber (White).” Earth and Planetary Science Letters 273 (nos. 3–4): 260–269.
Klein, Robert T., Kyger C. Lohmann, and Charles W. Thayer. 1996. “Bivalve Skeletons Record Sea-Surface Temperature And δ18O Via Mg/Ca And 18O/16O Ratios.” Geology 24, no. 5 (May 1): 415–418.
Kluge, Tobias, Cédric M. John, Anne-Lise Jourdan, Simon Davis, and John Crawshaw. 2015. “Laboratory Calibration Of the Calcium Carbonate Clumped Isotope Thermometer In The 25–250°C Temperature Range.” Geochimica et Cosmochimica Acta 157 (15 May): 213–227.
Köhler-Rink, Stephanie, and Michael Kühl. 2005. “The Chemical Microenvironment Of The Symbiotic Planktonic Foraminifera Orbulina universa.” Marine Biology Research 1, no. 1 (17 October): 68–78.
Kontakiotis, George, P. Graham Mortyn, Assimina Antonarakou, Miguel A. Martínez-Botí, and Maria V. Triantaphyllou. 2011. “Field-Based Validation Of A Diagenetic On G. ruber Mg/Ca Paleothermometry: Core Top Results From The Aegean Sea (Eastern Mediterranean).” Geochemistry, Geophysics, Geosystems 12, no. 9 (September): Q09004. doi: 10.1029/2011GC003692.
Kontakiotis, George, P. Graam Mortyn, Assimina Antonarakou, and Hara Drinia. 2016. “Assessing The Reliability Of Foraminiferal Mg/Ca Thermometry By Comparing Field-Samples And Culture Experiments: A Review.” Geological Quarterly 60, no. 3 (March): 547–560.
Kristjánsdóttir, G. B., D. W. Lea, A. E. Jennings, D. K. Pak, and C. Belanger. 2007. “New Spatial Mg/Ca-Temperature Calibrations For Three Arctic, Benthic Foraminifera And Reconstruction Of North Iceland Shelf Temperature For The Past 4000 Years.” Geochemistry, Geophysics, Geosystems 8, no. 3 (March): Q03P21. doi: 10.1029/2006GC001425.
Kucera, Michal, Mara Weinelt, Thorsten Kiefer, Uwe Pflaumann, Angela Hayes, Martin Weinelt, Min-Te Chen, Alan C. Mix, Timothy T. Barrows, Elsa Cortijo, Josette Duprat, Steve Juggins, and Claire Waelbroeck. 2005. “Reconstruction Of Sea-Surface Temperatures From Assemblages Of Planktonic Foraminifera: Multi-Technique Approach Based On Geographically Constrained Calibration Data Sets And Its Application To Glacial Atlantic And Pacific Oceans.” Quaternary Science Reviews 24, nos. 7–9: 951–998.
Kuffner, Ilsa B., Paul L. Jokiel, Ku’ulei S. Rodgers, Aandreas J. Andersson, and Fred T. Mackenzie. 2012. “An Apparent “Vital Effect” Of Calcification Rate On The Sr/Ca Temperature Proxy In The Reef Coral Montipora capitata.” Geochemistry, Geophysics, Geosystems 13, no. 8 (August): 1–10. doi:10.1029/2012GC004128.
Kunioka, Daisuke, Kotaro Shirai, Naoto Takahata, Yuji Sano, Takashi Toyofuku, and Yurika Ujiie. 2006. “Microdistribution Of Mg/Ca, Sr/Ca And Ba/Ca Ratios In Pulleniatina obliquiloculata Test By Using A NanoSIMS: Implications For The Vital Effect Mechanism.” Geochemistry, Geophysics, Geosystems 7, no. 12 (December): 1–11. doi: 10.1029/2006GC001280.
Kusakabe, Minoru, and Yukihiro Matsuhisa. 2008. “Oxygen Three-Isotope Ratios Of Silicate Reference Materials Determined By Direct Comparison With VSMOW-Oxygen.” Geochemical Journal 42, no. 4 (January): 309–317.
Laidler, K. J. 1996. “A Glossary Of Terms Used In Chemical Kinetics, Including Reaction Dynamics.” Pure and Applied Chemistry 68, no. 1: 149–192.
Langdon, Chris, Taro Takahashi, Colm Sweeney, Dave Chipman, John Goddard, Francesca Marubini, Heather Aceves, Heidi Barnett, and Marlin J. Atkinson. 2000. “Effect Of Calcium Carbonate Saturation State On The Calcification Rate Of An Experimental Coral Reef.” Global Biogeochemical Cycles 14, no. 2 (June): 639–654.
Lea, David W., Tracy A. Mashiotta, and Howard J. Spero. 1999. “Controls On Magnesium And Strontium Uptake In Planktonic Foraminifera Determined By Live Culturing.” Geochimica et Cosmochimica Acta 63, no. 16: 2369–2379.
Lea, D. W. 2014. “Elemental And Isotopic Proxies of Past Ocean Temperatures.” In Treatise on Geochemistry, 2nd ed., edited by H. D. Holland, and K. K. Turekian. Vol. 8: 373–397. Oxford, United Kingdom: Elsevier.
Lear, C. H., H. Elderfield, and P. A. Wilson. 2000. “Cenozoic Deep-Sea Temperatures And Global Ice Volumes From Mg/Ca In Benthic Foraminiferal Calcite.” Science 287 (14 January): 269–272.
Lear, Caroline H., Yair Rosenthal, and Niall Slowey. 2002. “Benthic Foraminiferal Mg/Ca-Paleothermometry: A Revised Core-Top Calibration.” Geochimica et Cosmochimica Acta 66, no. 19 (1 October): 3375–3387.
Lear, Caroline H., Elaine M. Mawbey, and Yair Rosenthal. 2010. “Cenozoic Benthic Foraminiferal Mg/Ca And Li/Ca Records: Toward Unlocking Temperatures And Saturation States.” Paleoceanography and Paleoclimatology 25, no. 4 (December): PA4215. doi: 10.1029/2009PA001880.
Levi, Camille, Laurent Labeyrie, Frank Bassinot, François Guichard, Elsa Cortijo, Claire Waelbroeck, Nicolas Caillon, Josette Duprat, Thibault de Garidel-Thoron, and Harry Elderfield. 2007. “Low-Latitude Hydrological Cycle And Rapid Climate Changes During The Last Deglaciation.” Geochemistry, Geophysics, Geosystems 8, no. 5 (May): Q05N12. doi: 10.1029/2006GC001514.
Li, Q., J. M. McArthur, and T. C. Atkinson. 2012. “Lower Jurassic Belemnites As Indicators Of Palaeo-Temperature.” Palaeogeography, Palaeoclimatology, Palaeoecology 315– 316 (15 January): 38–45.
Lisiecki, Lorraine E., and Maureen E. Raymo. 2005. “A Pliocene-Pleistocene Stack Of 57 Globally Distributed Benthic δ18O Records.” Paleoceanography and Paleoclimatology 20, no. 1 (March): PA1003. doi: 10.1029/2004PA001071.
Liu, Zonghui, Mark Pagani, David Zinniker, Robert DeConto, Matthew Huber, Henk Brinkhuis, Sunita R. Shah, R. Mark Leckie, and Ann Pearson. 2009a. “Global Cooling During The Eocene-Oligocene Climate Transition.” Science 323, no. 5918 (27 February): 1187–1190.
Liu, Z., B. L. Otto-Bliesner, F. He, E. C. Brady, R. Tomas, P. U. Clark, A. E. Carlson, et al. 2009b. “Transient Simulation Of Last Deglaciation With A New Mechanism For Bølling-Allerød Warming.” Science 325, no. 5938 (17 July): 310–314.
Lloyd, Max K., Uri Ryb, and John M. Eiler. 2018. “Experimental Calibration Of Clumped Isotope Reordering In Dolomite.” Geochimica et Cosmochimica Acta 242 (1 December): 1–20.
Marchitto, T. M., S. P. Bryan, W. B. Curry, and D. C. McCorkle. 2007. “Mg/Ca Temperature Calibration For The Benthic Foraminifer Cibicidoides pachyderma.” Paleoceanography and Paleoclimatology 22, no. 1 (March): PA1203. doi: 10.1029/2006PA001287.
Marchitto, T. M., S. P. Bryan, W. Doss, M. T. McCulloch, and P. Montagna. 2018. “A Simple Biomineralization Model To Explain Li, Mg, And Sr Incorporation Into Aragonitic Foraminifera And Corals.” Earth and Planetary Science Letters 481 (1 January): 20–29.
MARGO, 2004. MARGO Multiproxy Approach for the Reconstruction of the Glacial Ocean surface (archived project web pages and templates), Bremerhaven, PANGAEA. Retrieved from http://epic.awi.de/30068/ 10th February 2017.
Marlowe, I. T., S. C. Brassell, G. Eglinton, and J. C. Green. 1990. “Long-Chain Alkenones And Alkyl Alkenoates And The Fossil Coccolith Record Of Marine Sediments.” Chemical Geology 88, nos. 3–4 (November): 349–375.
Marr, Julene P., Joel A. Baker, Lionel Carter, Aidan S. R. Allan, Gavin B. Dunbar, and Helen C. Bostock. 2011. “Ecological And Temperature Controls On Mg/Ca Ratios Of Globigerina bulloides From The Southwest Pacific Ocean.” Paleoceanography and Paleoclimatology 26, no. 2 (June): PA2209. doi: 10.1029/2010PA002059.
Marriott, Caedmon S., Gideon M. Henderson, Nick S. Belshaw, and Alexander W. Tudhope. 2004. “Temperature Dependence Of δ7Li, δ44Ca And Li/Ca During Growing Of Calcium Carbonate.” Earth and Planetary Science Letters 222, no. 2 (30 May): 615–624.
Mathien-Blard, Elise, and Franck Bassinot. 2009. “Salinity Bias On The Foraminifera Mg/Ca Thermometry: Correction Procedure And Implications For Past Ocean Hydrographic Reconstructions.” Geochemistry, Geophysics, Geosystems 10, no. 12 (December): Q12011. doi: 10.1029/2008GC002353.
MathPages. 2019. “Bayesian Marbles.” https://www.mathpages.com/home/kmath143/kmath143.htm.
Maupin, Christopher R., Terrence M. Quinn, and Robert B. Halley. 2008. “Extracting A Climate Signal From The Skeletal Geochemistry Of The Caribbean Coral Siderastrea siderea.” Geochemistry, Geophysics, Geosystems 9, no. 12 (December): 1–13. doi: 10.1029/2008GC002106.
Medina-Elizalde, Martín, David W. Lea, and Matthew S. Fantle. 2008. “Implications Of Seawater Mg/Ca Variability For Plio-Pleistocene Tropical Climate Reconstruction.” Earth and Planetary Science Letters 269, nos. 3–4 (30 May): 585–595.
Meland, Marius Y., Eystein Jansen, Henry Elderfield. Trond M. Dokken, Are Olsen, and Richard G. J. Bellerby. 2006. “Mg/Ca Ratios In The Planktonic Foraminifer Neogloboquadrina pachyderma (Sinistral) In The Northern North Atlantic/Nordic Seas.” Geochemistry, Geophysics, Geosystems 7, no. 6 (June): Q06P14. doi: 10.1029/2005GC001078.
Mewes, Antje, Gerald Langer, Lennart J. de Nooijer, Jelle Bijma, and Gert-Jan Reichart. 2014. “Effect Of Different Seawater Mg2+ Concentrations On Calcification In Two Benthic Foraminifers.” Marine Micropaleontology 113 (December): 56–64.
Miller, Kenneth G., Richard G. Fairbanks, and Gregory S. Mountain. 1987. “Tertiary Oxygen Isotope Synthesis, Sea Level History, And Continental Margin Erosion.” Paleoceanography and Paleoclimatology 2, no. 1 (February): 1–19. doi: 10.1029/PA002i001p00001.
Min, G. Rong, R. Lawrence Edwards, Frederick W. Taylor, Jacques Récy, Christina D. Gallup, and J. Warren Beck. 1995. “Annual Cycles of U/Ca In Coral Skeletons And U/Ca Thermometry.” Geochimica et Cosmochimica Acta 59, no. 10 (May): 2025–2042.
Mitsuguchi, Takehiro, Eiji Matsumoto, Osamu Abe, Tetsuo Uchida, and Peter J. Isdale. 1996. “Mg/Ca Thermometry In Coral Skeletons.” Science 274, no. 5289 (8 November): 961–963.
Mix, Alan C., Edouard Bard, and Ralph Schneider. 2001. “Environmental Processes Of The Ice Age: Land, Oceans, Glaciers (EPILOG).” Quaternary Science Reviews 20, no. 4 (February): 627–657.
Montagna, Paolo, Malcolm McCulloch, Eric Douville, Matthias López Correa, Julie Trotter, Riccardo Rodolfo-Metalpa, Delphine Dissard, et al. 2014. “Li/Mg Systematics In Scleractinian Corals: Calibration Of The Thermometer.” Geochimica et Cosmochimica Acta 132 (1 May): 288–310.
Müller, Peter J., Gerog Kirst, Götz Ruhland, Isabel von Storch, and Antoni Rosell-Melé. 1998. “Calibration Of The Alkenone Paleotemperature Index UK’37 Based On Core-Tops From The Eastern South Atlantic And The Global Ocean (60°N–60°S).” Geochimica et Cosmochimica Acta 62, no. 10 (May): 1757–1772.
Müller, R. Dietmar, Maria Sdrolias, Carmen Gaina, and Walter R. Roest. 2008. “Age, Spreading Rates, And Spreading Asymmetry Of The World’s Ocean Crust.” Geochemistry, Geophysics, Geosystems 9, no. 4 (April): 1–19: Q04006. doi: 10.1029/2007GC001743.
Naafs, B. D. A., J. Hefter, and R. Stein. 2012. “Application Of The Long Chain Diol Index (LDI) Paleothermometer To The Early Pleistocene (MIS 96).” Organic Geochemistry 49 (August): 83–85.
Nehrke, G., N. Keul, G. Langer, L. J. de Nooijer, J. Bijma, and A. Meibom. 2013. “A New Model For Biomineralization And Trace-Element Signatures Of Foraminifera Tests.” Biogeosciences 10 (29 October): 6759–6767. doi: 10.5194/bg-10-6759-2013.
Nigam, Rajiv, Rajeev Saraswat, and Abhijit Mazumder. 2003. “Life Spans Of Planktonic Foraminifers: New Insight Through Sediment Traps.” Journal of the Palaeontological Society of India 48: 129–133.
Nürnberg, Dirk, Jelle Bijma, and Christoph Hemleben. 1996. “Assessing The Reliability Of Magnesium In Foraminiferal Calcite As A Proxy For Water Mass Temperatures.” Geochimica et Cosmochimica Acta 60, no. 5 (March): 803–814.
Nürnberg, D., A. Müller, and R. R. Schneider. 2000. “Paleo-Sea Surface Temperature Calculations In The Equatorial East Atlantic From Mg/Ca Ratios In Planktic Foraminifera: A Comparison To Sea Surface Temperature Estimates From UK’37, Oxygen Isotopes, And Foraminiferal Transfer Function.” Paleoceanography and Paleoclimatology 15, no. 1 (February): 124–134. http://doi.org/10.1029/1999PA000370.
O’Neil, James R., Robert N. Clayton, and Toshiko K. Mayeda. 1969. “Oxygen Isotope Fractionation in Divalent Metal Carbonates.” Journal of Chemical Physics 51: 5547–5558.
Oomori, Tamotsu, Hiroshi Kaneshima, Yoko Maezato, and Yasushi Kitano. 1987. “Distribution Coefficient Of Mg2+ Ions Between Calcite And Solution At 10–50°C.” Marine Chemistry 20, no. 4 (March): 327–336.
Pak, Dorothy K., David W. Lea, and James P. Kennett. 2004. “Seasonal And Interannual Variation In Santa Barbara Basin Water Temperatures Observed In Sediment Trap Foraminiferal Mg/Ca.” Geochemistry, Geophysics, Geosystems 5, no. 12 (December): Q12008. doi: 10.1029/2004GC000760.
Park, Yu-Hyeon, Masanobu Yamamoto, Seung-Il Nam, Tomohiro Irino, Leonid Polyak, Naomi Harada, Kana Nagashima, Boo-Keun Khim, Kazuhisa Chikita, and Sei-Icho Saitoh. 2014. “Distribution, Source And Transportation Of Glycerol Dialkyl Glycerol Tetraethers In Surface Sediments From The Western Arctic Ocean And The Northern Bering Sea.” Marine Chemistry 165 (20 October): 10–24.
Passey, Benjamin H., and Gregory A. Henkes. 2012. “Carbonate Clumped Isotope Bond Reordering And Geospeedometry.” Earth and Planetary Science Letters 351–352 (15 October): 223–236.
Pawlowicz, Rich. 2013. “Key Physical Variables In The Ocean: Temperature, Salinity, And Density.” Nature Education Knowledge 4, no. 4: 13. https://www.nature.com/scitable/knowledge/library/key-physical-variables-in-the-ocean-temperature-102805293/.
Pérez-Huerta, Alberto, Ismael Coronado, and Thomas A. Hegna. 2018. “Understanding Biomineralization In The Fossil Record.” Earth-Science Reviews 179 (April): 95–122.
Peterson, Ray G., and Lothar Stramma. 1991. “Upper-Level Circulation In The South Atlantic Ocean.” Progress in Oceanography 26 (no. 1): 1–73.
Pogge von Strandmann, Philip A. E. 2008. “Precise Magnesium Isotope Measurements In Core Top Planktic And Benthic Foraminifera.” Geochemistry, Geophysics, Geosystems 9, no. 12 (December): Q12015. doi: 10.1029/2008GC002209.
Prahl, F. G., and S. G. Wakeham. 1987. “Calibration Of Unsaturation Patterns In Long-Chain Ketone Compositions For Palaeotemperature Assessment.” Nature 330 (26 November): 367–369.
Railsback, L. Bruce, Philip L. Gibbard, Martin J. Head, Ny Riavo G. Voarintsoa, and Samuel Toucanne. 2015. “An Optimized Scheme Of Lettered Marine Isotope Substages For The Last 1.0 Million Years, And The Climostratigraphic Nature Of Isotope Stages And Substages.” Quaternary Science Reviews 111 (1 March): 94–106.
Raitzsch, M., A. Dueñas-Bohórquez, G.-J. Reichart, L. J. de Nooijer, and T. Bickert. 2010. “Incorporation Of Mg And Sr In Calcite Of Cultured Benthic Foraminifera: Impact Of Calcium Concentration And Calcite Saturation State.” Biogeosciences 7, no. 3 (4 March): 869–881. doi: 10.5194/bg-7-869-2010.
Raja, Ramalingam, Pratul Kumar Saraswati, Karyne Rogers, and Kenji Iwao. 2005. “Magnesium And Strontium Compositions Of Recent Symbiont-Bearing Benthic Foraminifera.” Marine Micropaleontology 58 (December): 31–44.
Rampen, Sebastiaan W., Verónica Willmott, Jung-Hyun Kim, Eleonora Uliana, Gesine Mollenhauer, Enno Schefuss, Jaap S. Sinninghe Damsté, and Stefan Schouten. 2012. “Long Chain 1,13- And 1,15-Diols As A Potential Proxy For Palaeotemperature Reconstruction.” Geochimica et Cosmochimica Acta 84 (1 May): 204–216.
Ravelo, Ana Christina, and Claude Hillaire-Marcel. 2007. “The Use Of Oxygen And Carbon Isotopes Of Foraminifera In Paleoceanography.” In Developments in Marine Geology, edited by Claude Hillaire-Marcel and Anne De Vernal. Vol. 1: 735–764. Amsterdam, Netherlands: Elsevier.
Ray, Paul H., David C. White, and Thomas D. Brock. 1971. “Effect Of Temperature On The Fatty Acid Composition Of Thermus aquaticus.” Journal of Bacteriology 106, no. 1 (May): 25–30.
Regenberg, Marcus, Dirk Nürnberg, Silke Steph, Jeroen Groeneveld, Dieter Garbe-Schönberg, Ralf Tiedemann, and and Wolf-Christian Dullo. 2006. “Assessing The Effect Of Dissolution On Planktonic Foraminiferal Mg/Ca Ratios: Evidence From Caribbean Core Tops.” Geochemistry, Geophysics, Geosystems 7, no. 7 (July): Q07P15. doi: 10.1029/2005GC001019.
Regenberg, Marc, Silke Steph, Dirk Nürnberg, Ralf Tiedemann,and Dieter Garbe-Schönberg. 2009. “Calibrating Mg/Ca Ratios Of Multiple Planktonic Foraminiferal Species With δ18O-Calcification Temperatures: Paleothermometry For The Upper Water Column.” Earth and Planetary Science Letters 278, no. 3–4 (25 February): 324–336.
Richey, Julie N., and Jessica E. Tierney. 2016. “GDGT And Alkenone Flux In The Northern Gulf Of Mexico: Implications For The TEX86 and UK’37 Paleothermometers.” Paleoceanography and Paleoclimatology 31, no. 12 (December): 1547–1561. doi:10.1002/2016PA003032.
Richter, Frank M., and Donald J. DePaulo. 1987. “Numerical Models For Diagenesis And The Neogene Sr Isotopic Evolution Of Seawater From DSDP Site 590B.” Earth and Planetary Science Letters 83 (nos. 1–4): 27–38.
Robinson, Stuart A., Micha Ruhl, David Lee Astley, B. David A. Naafs, Alexander J. Farnsworth, Paul R. Bown, Hugh C. Jenkyns, Daniel J. Lunt, Charlotte O’Brien, Richard D. Pancost, and Paul J. Markwick. 2017. “Early Jurassic North Atlantic Sea-Surface Temperatures From TEX86 Palaeothermometry.” Sedimentology 64, no. 1 (January) Special Issue: Mesozoic Climates and Oceans: 215–230.
Rodrigo-Gámiz, M., F. Martínez-Ruiz, S. W. Rampen, S. Schouten, and J. S. Sinninghe Damsté. 2014. “Sea Surface Temperature Variations In The Western Mediterranean Sea Over The Last 20 Kyr: A Dual-Organic Proxy (UK’37 and LDI) Approach.” Paleoceanography and Paleoclimatology 29, no. 2 (February): 87–98. doi:10.1002/2013PA002466.
Rodrigo-Gámiz, M., S. W. Rampen, H. de Haas, M. Baas, S. Schouten, and J. S. Sinninghe Damsté. 2015. “Constraints On The Applicability Of The Organic Temperature Proxies UK’37, TEX86 And LDI In The Subpolar Region Around Iceland.” Biogeosciences 12: 6573–6590. doi: 10.5194/bg-12-6573-2015.
Rohling, E. J., G. Marino, and K. M. Grant. 2015. “Mediterranean Climate And Oceanography, And The Periodic Development Of Anoxic Events (Sapropels).” Earth-Science Reviews 143 (April): 62–97.
Rosenthal, Yair, Edward A. Boyle, and Niall Slowey. 1997. “Temperature Control On The Incorporation Of Magnesium, Strontium, Fluorine, And Cadmium Into Benthic Foraminiferal Shells From Little Bahama Bank: Prospects For Thermohaline Paleoceanography.” Geochimica et Cosmochimica Acta 61, no. 17 (September): 3633–3642.
Rosenthal, Y., G. P. Lohmann, K. C. Lohmann, and R. M. Sherrell. 2000. “Incorporation And Preservation Of Mg in Globigerinoides sacculifer: Implications For Reconstructing The Temperature And 18O/16O Of Seawater.” Paleoceanography and Paleoclimatology 15, no. 1(February): 135–145. https://doi.org/10.1029/1999PA000415.
Rosenthal, Yair, and George P. Lohmann. 2002. “Accurate Estimation Of Sea Surface Temperatures Using Dissolution Corrected Calibrations For Mg/Ca Paleothermometry.” Paleoceanography and Paleoclimatology 17, no. 3 (September): 1044–1049. doi:10.1029/2001PA000749, 2002.
Rosenthal, Yair. and Braddock Linsley. 2006. “Mg/Ca And Sr/Ca Paleothermometry From Calcareous Marine Fossils.” In The Encyclopedia of Quaternary Sciences, edited by Scott Elias and Cary Mock, 871–883. Amsterdam, Netherlands: Elsevier.
Russell, Ann D., Bärbel Hönisch, Howard J. Spero, and David W. Lea. 2004. “Effects Of Seawater Carbonate Ion Concentration And Temperature On shell U, Mg, And Sr In Cultured Planktonic Foraminifera.” Geochimica et Cosmochimica Acta 68, no. 21 (1 November): 4347–4361.
Sabbatini, Anna, Franck Boussinot, Soumaya Boussetta, Alessandra Negri, Hélène Rebaubier, Fabien Dewilde, Julius Nouet, Nicolas Caillon, and Caterina Morigi. 2011. “Further Constraints On The Diagenetic Influences And Salinity Effect On Globigerinoides ruber (White) Mg/Ca Thermometry: Implications In The Mediterranean Sea.” Geochemistry Geophysics Geosystems 12, no. 10: Q10005. doi: 10.1029/2011GC003675.
Sachs, Julian P., Ralph R. Schneider, Timothy I. Eglinton, Katherine H. Freeman, Gerald Ganssen, Jerry F. McManus, and Delia W. Oppo. 2000. “Alkenones As Paleoceanographic Proxies.” Geochemistry Geophysics Geosystems 1 (November 21): 2000GC000059. doi.org/10.1029/2000GC00059.
Sadekov, Aleksey Yu, Stephen M. Eggins, and Patrick De Deckker. 2005. “Characterization Of Mg/Ca Distributions In Planktonic Foraminifera Species By Electron Microprobe Mapping.” Geochemistry, Geophysics, Geosystems 6, no. 12: Q12P06. doi: 10.1029/2005GC000973.
Sadekov, Aleksey, Stephen M. Eggins, Patrick De Deckker, and Dick Kroon. 2008. “Uncertainties In Seawater Thermometry Deriving From Intratest And Intertest Mg/Ca Variability In Globigerinoides ruber.” Paleoceanography and Paleoclimatology 23, no. 1: PA1215. doi: 10.1029/2007PA001452.
Sadekov, Aleksey, Stephen M. Eggins, Patrick De Deckker, Ulysses Ninnemann, Wolfgang Kuhnt, and Franck Bassinot. 2009. “Surface And Subsurface Seawater Temperature Reconstruction Using Mg/Ca Microanalysis Of Planktonic Foraminifera Globigerinoides ruber, Globigerinoides sacculifer, And Pulleniatina obliquiloculata.” Paleoceanography and Paleoclimatology 24, no. 3: PA3201. doi: 10.1029/2008PA001664.
Sadekov, Aleksey Yu, Flora Bush, Joanna Kerr, Raja Ganeshram, and Henry Elderfield. 2014. “Mg/Ca Composition Of Benthic Foraminifera Miliolacea As A New Tool Of Paleoceanography.” Paleoceanography and Paleoclimatology 29, no. 10: 990–1001. doi: 10.1002/2014PA002654.
Saenger, Casey, Anne L. Cohen, Delia W. Oppo, and Dennis Hubbard. 2008. “Interpreting Sea Surface Temperature From Strontium/Calcium Ratios In Montastrea Corals: Link With Growth Rate And Implications For Proxy Reconstructions.” Paleoceanography and Paleoclimatology 23, no. 3: PA3102. doi: 10.1029/2007PA001572.
Savin, Samuel M., and Robert G. Douglas. 1973. “Stable Isotope And Magnesium Geochemistry Of Recent Planktonic Foraminifera From The South Pacific.” GSA Bulletin 84, no. 7 (July 1): 2327–2342.
Schauer, Andrew J., Julia Kelson, Casey Saenger, and Katharine W. Huntington. 2016. “Choice Of 17O Correction Affects Clumped Isotope (Δ47) Values Of CO2 Measured With Mass Spectrometry.” Rapid Communications in Mass Spectrometry 30, no. 24 (30 December): 2607–2616.
Schouten, Stefan, Ellen C. Hopmans, Enno Schefuß, and Jaap S. Sinninghe Damsté. 2002. “Distributional Variations In Marine Crenarchaeotal Membrane Lipids: A New Tool For Reconstructing Ancient Sea Water Temperatures?” Earth and Planetary Science Letters 204, nos. 1–2 (30 November): 265–274.
Schrag, Daniel P., Donald J. DePaolo, and Frank M. Richter. 1992. “Oxygen Isotope Exchange In A Two-Layer Model Of Oceanic Crust.” Earth and Planetary Science Letters 111, nos. 2–4 (July): 305–317.
Segev, Einat., and Jonathan Erez. 2006. “Effect Of Mg/Ca Ratio In Seawater On Shell Composition In Shallow Benthic Foraminifera.” Geochemistry, Geophysics, Geosystems 7, no. 2 (February): Q02P09. doi: 10.1029/2005GC000969.
Servais, Thomas, David A. T. Harper, Jun Li, Axel Munnecke, Alan W. Owen, and Peter M. Sheehan. 2009. “Understanding The Great Ordovician Diversification Event (GOBE): Influences Of Paleogeography, Paleoclimate, Or Paleoecology?” GSA Today 19, nos. 4–5 (April/May): 4–10.
Shackleton, N. J. 1974. “Attainment Of Isotopic Equilibrium Between Ocean Water And The Benthonic Foraminifera Genus Uvigerina: Isotopic Changes In The Ocean During The Last Glacial.” Centre National de la Recherche Scientifique Colloques Internationaux 219: 203–209.
Skinner, L. C., and H. Elderfield. 2005. “Constraining Ecological And Biological Bias In Planktonic Foraminiferal Mg/Ca And δ18Occ: A Multispecies Approach To Proxy Calibration Testing.” Paleoceanography and Paleoclimatology 20, no. 1 (March), PA1015. doi: 10.1029/2004PA001058.
Skirbekk, Kari, Morten Hald, Thomas M. Marchitto, Juho Junttila, Dorthe Klitgaard Kristensen, and Steffen Aagaard Sørensen. 2016. “Benthic Foraminiferal Growth Seasons Implied From Mg/Ca-Temperature Correlations For Three Arctic Species.” Geochemistry, Geophysics, Geosystems 17, no. 11: 4684–4704. doi.org/10.1002/2016GC006505.
Snelling, Andrew A., Martin Ernst, Esther Scheven, Joachim Scheven, Steven A. Austin, Kurt P. Wise, Paul Garner, Michael Garton, and David Tyler, D. 1996. “The Geological Record.” Creation Ex Nihilo Technical Journal 10, no. 3 (December): 333–334.
Sosdian, S. M., R. Greenop, M. P. Hain, G. L. Foster, P. N. Pearson, and C. H. Lear. 2018. “Constraining The Evolution Of Neogene Ocean Carbonate Chemistry Using The Boron Isotope pH Proxy.” Earth and Planetary Science Letters 498 (15 September): 362–376.
Spero, Howard J., Stephen M. Eggins, Ann D. Russell, Lael Vetter, Matt R. Kilburn, and Bärbel Hönisch. 2015. “Timing And Mechanism For Intratest Mg-Variability In A Living Planktic Foraminifer.” Earth and Planetary Science Letters 409 (1 January): 32–42.
Spezzaferri, Silvia, Michal Kucera, Paul Nicholas Pearson, Bridget Susan Wade, Sacha Rappo, Christopher Robert Poole, Raphaël Morard, and Claudio Stalder. 2015. “Fossil And Genetic Evidence For The Polyphyletic Nature Of The Planktonic Foraminifera “Globigerinoides”, And Description Of The New Genus Trilobatus.” PLoS ONE 10, no. 5 (May 28): e0128108. doi: 10.1371/journal.pone.0128108.
Steph, Silke, Ralf Tiedemann, Matthias Prange, Jeroen Groeneveld, Dirk Nürnberg, Lars Reuning, Michal Schulz, and Gerald H. Haug. 2006. “Changes In Caribbean Surface Hydrography During The Pliocene Shoaling Of The Central American Seaway.” Paleoceanography and Paleoclimatology 21, no. 4 (December): PA4221. doi: 10.1029/2004PA001092.
Stolper, Daniel A., John M. Eiler, and John A. Higgins. 2018. “Modeling The Effects Of Diagenesis On Carbonate Clumped-Isotope Values In Deep-And Shallow-Water Settings.” Geochimica et Cosmochimica Acta 227 (15 April): 264–291.
Stott, Lowell, Kevin Cannariato, Robert Thunell, Gerald H. Haug, Athanasios Koutavas, and Steve Lund. 2004. “Decline Of Surface Temperature And Salinity In The Western Tropical Pacific Ocean In The Holocene Epoch.” Nature 431 (2 September): 56–59.
Stott, Lowell, Axel Timmermann, and Robert Thunell. 2007. “Southern Hemisphere And Deep-Sea Warming Led Deglacial Atmospheric CO2 Rise And Tropical Warming.” Science 318, no. 5849 (19 October): 435–438.
Swart, P. K, H. Elderfield, and M. J. Greaves. 2002. “A High-Resolution Calibration Of Sr/Ca Thermometry Using The Caribbean Coral Montastraea annularis.” Geochemistry, Geophysics, Geosystems 3, no. 11 (November): 1–11. doi: 10.1029/2002GC000306.
Tachikawa, Kazuyo, Sophie Sépulcre, Takashi Toyofuku, and Edouard Bard. 2008. “Assessing Influence Of Diagenetic Carbonate Dissolution On Planktonic Foraminiferal Mg/Ca In The Southeastern Arabian Sea Over The Past 450 Ka: Comparison Between Globigerinoides ruber And Globigerinoides sacculifer.” Geochemistry, Geophysics, Geosystems 9, no. 4 (April): Q04037. doi: 10.1029/2007GC001904.
Tierney, Jessica E. 2012. “GDGT Thermometry: Lipid Tools For Reconstructing Paleotemperatures.” In Reconstructing Earth’s Deep-Time Climate, edited by Linda C. Ivany, and Brian T. Huber. The Paleontological Society Papers,Vol. 18: 115–131. Paleontological Society Short Course, November 3, 2012. Charlotte, North Carolina: The Paleontological Society.
Tierney, J. E. 2014. “Biomarker-Based Inferences Of Past Climate: The TEX86 Paleotemperature Proxy.” In Treatise on Geochemistry, 2nd ed. vol. 2, chapter 12, 379–393, edited by H. D. Holland, and K. K. Turekian. Amsterdam, Netherlands: Elsevier.
Tierney, Jessica E., and Martinn P. Tingley. 2015. “A TEX86 Surface Sediment Database And Extended Bayesian Calibration.” Scientific Data 2 (23 June): 150029. doi: 10.1038/sdata.2015.29.
Tierney, Jessica E., Nerilie J. Abram, Kevin J. Anchukaitis, Michael N. Evans, Cyril Giry, K. Halimeda Kilbourne, Casey P. Saenger, Henry C. Wu, and Jens Zinke. 2015. “Tropical Sea Surface Temperatures For The Past Four Centuries Reconstructed From Coral Archives.” Paleoceanography and Paleoclimatology 30, no. 3 (March): 226–252. doi:10.1002/2014PA002717.
Tierney, Jessica E., and Martin P. Tingley. 2018. “BAYSPLINE: A New Calibration For The Alkenone Paleothermometer.” Paleoceanography and Paleoclimatology 33, no. 3 (March): 281–301. doi: 10.1002/2017PA003201.
Toyofuku, Takashi, Hiroshi Kitazato, Hodaka Kawahata, Masashi Tsuchiya, and Masato Nohara. 2000. “Evaluation Of Mg/Ca Thermometry In Foraminifera: Comparison Of Experimental Results And Measurements In Nature.” Paleoceanography and Paleoclimatology 15, no. 4 (August): 456–464. doi:10.1029/1000PA000460.
Tribble, Jane S., and Fred T. MacKenzie. 1998. “Recrystallization Of Magnesian Calcite Overgrowths On Calcite Seeds Suspended In Seawater.” Aquatic Geochemistry 4, nos. 3–4 (September): 337–360.
Vander Putten, Erika, Frank Dehairs, Eddy Keppens, and Willy Baeyens. 2000. “High Resolution Distribution Of Trace Elements In The Calcite Shell Layer Of Modern Mytilus edulis: Environmental And Biological Controls.” Geochimica et Cosmochimica Acta 64, no. 6 (March): 997–1011.
Van Raden, Ulrike Jannette, Jeroen Groeneveld, Markus Raitzsch, and Michal Kucera. 2011. “Mg/Ca In The Planktonic Foraminifera Globorotalia inflata And Globigerinoides bulloides From Western Mediterranean Plankton Tow And Core Top Samples.” Marine Micropaleontology 78, nos. 3–4 (March): 101–112.
Veizer, Jan, and Andreas Prokoph. 2015. “Temperatures And Oxygen Isotopic Composition Of Phanerozoic Oceans.” Earth-Science Reviews 146 (July): 92–104.
Visser, Katherine, Robert Thunell, and Lowell Stott. 2003. “Magnitude And Timing Of Temperature Change In The Indo-Pacific Warm Pool During Deglaciation.” Nature 421 (9 January): 152–155.
Von Langen, Peter J., Dorothy K. Pak, Howard J. Spero, and David W. Lea. 2005. “Effects Of Temperature On Mg/Ca In Neogloboquadrinid Shells Determined By Live Culturing.” Geochemistry, Geophysics, Geosystems 6, no. 10 (October): Q10P03. doi: 10.1029/2005GC000989.
Watkins, James M., Laura C. Nielsen, Frederick J. Ryerson, and Donald J. DePaolo. 2013. “The Influence Of Kinetics On The Oxygen Isotope Composition Of Calcium Carbonate.” Earth and Planetary Science Letters 375 (1 August): 349–360.
Watkins, James M., Jonathan D. Hunt, Frderick J. Ryerson, and Donald J. DePaolo. 2014. “The Influence of Temperature, pH, And Growth Rate On The δ18O Composition Of Inorganically Precipitated Calcite.” Earth and Planetary Science Letters 404 (15 October): 332–343.
Watkins, J. M., and J. D. Hunt. 2015. “A Process-Based Model For Non-Equilibrium Clumped Isotope Effects In Carbonates.” Earth and Planetary Science Letters 432 (15 December): 152–165.
Weinkauf, Manuel F. G., José G. Kunze, Joanna J. Waniek, and Michal Kučera. 2016. “Seasonal Variation In Shell Calcification Of Planktonic Foraminifera In The NE Atlantic Reveals Species-Specific Response To Temperature, Productivity And Optimum Growth Conditions.” PLoS ONE 11, no. 2 (February 9): e0148363. doi: 10.1371/journal.pone.0148363.
Weldeab, Syee, Adam Arce, and Sabine Kasten. 2016. “Mg/Ca-ΔCO32-pore water–Temperature Calibration For Globobulimina spp.: A Sensitive Paleothermometer For Deep-Sea Temperature Reconstruction.” Earth and Planetary Science Letters 438 (15 March): 95–102.
Worraker, William. 2018. “Heat Problems Associated With Genesis Flood Models—Part 1: Introduction And Thermal Boundary Conditions.” Answers Research Journal 11:171– 191. https://answersingenesis.org/geology/geologic-time-scale/heat-problems-associated-with-genesis-flood-models-part-1/.
Wu, Henry C., Braddock K. Linsley, Emilie P. Dassié, Benedetto Schiraldi Jr., and Peter B. deMenocal. 2013. “Oceanographic Variability In The South Pacific Convergence Zone Region Over The Last 210 Years from Multi-Site Coral Sr/Ca Records.” Geochemistry, Geophysics, Geosystems 14 (May): 1435–1453. doi:10.1029/2012GC004293.
Zaarur, Shikma, Hagit P. Affek, and Mark T. Brandon. 2013. “A Revised Calibration Of The Clumped Isotope Thermometer.” Earth and Planetary Science Letters 382 (15 November): 47–57.
Zhang, Peng, Rina Zuraida, Yair Rosenthal, Ann Holbourn, Wolfgang Kuhnt, and Jian Xu. 2019. “Geochemical Characteristics From Tests Of Four Modern Planktonic Foraminiferal Species In The Indonesian Throughflow Region And Their Implications.” Geoscience Frontiers 10, no. 2 (March): 505–516.
Zhang, Yi Ge, Mark Pagani, and Zhengrong Wang. 2016. “Ring Index: A New Strategy To Evaluate The Integrity Of TEX86 Paleothermometry.” Paleoceanography and Paleoclimatology 31, no. 2 (February): 220–232. doi: 10.1002/2015PA002848.
Zinke, Jens, Juan P. D’Olivo, Chrisoph J. Gey, Malcolm T. McCulloch, J. Henrich Bruggemann, Janice M. Lough, and Mireille M. M. Guillaume. 2019. “Multi-Trace-Element Sea Surface Temperature Coral Reconstruction For The Southern Mozambique Channel Reveals Teleconnections With The Tropical Atlantic.” Biogeosciences 16 no. 3 (4 February): 695–712.
Nomenclature
Bayesian: Bayesian statistics is based on the Bayesian interpretation of probability, in which probability expresses a measure of belief or expectation of an event, which can change in response to new information, in contrast to the traditional frequentist interpretation which views probability as the limit of the relative frequency of an event after a large number of trials. It uses Bayes’ theorem to compute and update probabilities after obtaining new data. Bayes’ theorem describes the conditional probability of an event based on data plus prior information or beliefs about the event or conditions related to the event (e.g. MathPages 2019). For example, in Bayesian inference, Bayes’ theorem can be used to estimate the parameters of a probability distribution or statistical model.
Benthic: Benthic organisms (collectively benthos) live at the bottom of an ocean or lake. Species living on the bottom are described as epifaunal, while those living within the surface layer or just below are termed infaunal.
Bølling/Allerød: A warm climate interval starting abruptly following the Heinrich 1 event at the end of the Pleistocene and ending with the start of the Younger Dryas, i.e. occupying the interval ~14.5–12.9 ka BP in the conventional chronology (Carlson et al. 2007; Liu et al. 2009b).
Coccoliths: Plates of calcite formed by single-celled algae known as coccolithophores; coccoliths are the main constituent of chalk deposits.
Diol: These are alcohols, hydrocarbon molecules characterized by two hydroxyl groups attached to a central chain of saturated carbon atoms. In the IUPAC naming convention they are formally denoted alkane-n,n-diol, where alkane corresponds to the number of carbon atoms in the chain, and n,n give the numbers of the carbons in the sequence where the hydroxyls are attached. The sequence is numbered so as to give one of the hydroxyl groups the lowest possible number. Thus, for example, propane-1,2-diol has 3 carbon atoms in its chain, with hydroxyl groups attached to atoms 1 and 2 in the sequence. The diols of interest in temperature reconstructions are based on considerably longer alkanes than this.
Eustigmatophyte: Single-celled eukaryotic algae found in marine and freshwater environments and soils. They are photosynthetic autotrophs, i.e. their main energy source is sunlight.
Gametogenesis: The production of gametes, the cells involved in sexual reproduction to produce a new individual organism. At this point in the life cycle of a calcifying foraminifer it may deposit a significant amount of calcite in its test (Köhler-Rink and Kühl 2005).
Heinrich 1 (or H1): The last of a series of events (H1-H6) generally viewed as the production of large numbers of icebergs in the Hudson Strait region of Canada. H1 is dated in the conventional chronology at about 18 ka BP. The defining diagnostic feature of Heinrich events in sediment cores is the presence of detrital carbonate (limestone and dolomite) from lower Palaeozoic basins of Northern Canada. Heinrich events are also marked by high lithic to foraminifer ratio and increased relative abundance of the cold-water planktonic foraminifer Neogloboquadrina pachyderma (Hodell et al. 2008).
Isomer: Molecules with the same chemical formula but different chemical structures. Isomers do not necessarily share similar chemical properties unless they also have the same functional groups.
Mixed layer: When referring to the oceans, this is the near-surface region which has been homogenized in temperature, density and salinity by turbulence generated by (1) wind and wave action, often generating or interacting with ocean currents, and (2) Rayleigh-Taylor instability, a vertical instability due to cooling at the surface or to injection of brine produced by sea ice formation. Typically the mixed layer extends downwards by 20–90 m, being deeper in winter than in summer. However it may extend down much further (as in the Labrador Sea) if a Rayleigh-Taylor instability occurs. Beneath the mixed layer are the barrier layer and the thermocline, where temperature falls rapidly with increasing depth.
Oil window: The geological environment in which petroleum products are thought to form from kerogen (solid organic material) as a result of thermal degradation and cracking during burial. The typical range of depths is 760–4,880 m and the range of temperatures 65–150°C.
Partition coefficient: The ratio of equilibrium concentrations of a particular compound (atomic or molecular) between two immiscible solvents, at least one of which is aqueous: the second phase, although usually liquid, may be gas, liquid or solid. In the case of magnesium in the context of Mg/Ca thermometry, it is denoted DMg and refers to the ratio of magnesium (Mg2+) concentrations in the shell calcite and seawater respectively.
Pelagic: The pelagic zone of the oceans refers to the water column of the open ocean. Thus the benthic zone and the demersal zone immediately above it are excluded, as are coastal and continental shelf regions.
Planktonic (or planktic): Planktonic/planktic organisms live in open water, generally in the near-surface region, and are unable to swim against a current. Hence in the oceans they are carried along by the prevailing near-surface currents. Most are microscopic in size, though larger organisms (e.g. jellyfish) are included in the definition.
Porcelaneous: Benthic foraminifera can be agglutinated or calcareous. Calcareous species are divided into those whose shells have a clear or translucent appearance (hyaline) with tiny perforations (pores) and those whose shells are white and opaque and have no perforations (porcelaneous).
Primary Organic Membrane (POM): Most extant planktonic foraminifera produce their tests by the sequential addition of chambers. The walls of each chamber are initially formed of two calcite layers (a bilamellar wall) secreted on either side of an organic template or sheet known as the Primary Organic Membrane or POM. Additional layers are deposited on the outer surface of existing chambers as each successive chamber is added to the test during ontogenetic growth. Just prior to gametogenesis a final, often thick, layer is deposited on the outside of each chamber exposed in the last whorl of the test (Erez 2003; Sadekov, Eggins and De Dekker 2005). Erez (2003) suggests that a better term would be Primary Organic Sheet (acronym POS) as POM can also be used to mean “particulate organic matter”, but POM will be used in this article.
Rayleigh fractionation: This describes the chemical evolution of a system with multiple phases in which one phase is continuously removed from the system. Consider, for example, the case of coral skeleton aragonite formation in which the Sr/Ca ratio of the precipitate is the key variable of interest. Gaetani et al. (2011) explain the Rayleigh fractionation process in terms of the average concentrations of Sr and Ca in aragonite precipitated from a single “batch” of fluid, respectively CSr and CCa; the concentrations of Sr and Ca in the calcifying fluid as precipitation begins, respectively CSr0 and CCa0; the remaining mass fraction of the calcifying fluid FL; and the temperature-dependent Nernst partition coefficients between aragonite and seawater, DSrAragonite-Seawater and DCaAragonite-Seawater; these partition coefficients express the equilibrium ratios CiAragonite/CiSeawater for phase i. Given previously determined values of the Nernst partition coefficients for Ca, Mg, Sr and Ba between aragonite and seawater as functions of temperature, Gaetani et al. (2011) measured Mg/Ca, Sr/Ca and Ba/Ca ratios on the coral skeletons of interest and were then then able to estimate formation temperatures analytically from their Rayleigh fractionation equations.
Regioisomer: A type of constitutional isomer. In constitutional isomers molecules with the same molecular formula are bonded together in different orders. In a regioisomer, a functional group or other molecular component changes position on a parent structure. In long organic molecules the parent structure is a basic “carbon skeleton” to which various molecular groups can be attached.
Salinity: Ocean water salinity is the mass fraction of dissolved salts (mostly but not exclusively sodium chloride), typically about 3.5% or 35‰ in modern-day oceans. It is relatively low in the Baltic Sea and can be very high in enclosed inland seas subject to high evaporation (e.g. the Dead Sea). Although conceptually simple salinity is not straightforward to measure: the water has to be filtered down to a pore size of 0.2 μm and pressure, temperature and electrical conductivity all need to be measured. Practical salinity units, or psu, give approximately the same numbers as salinity defined in terms of mass fraction, but are conceptually different; their use is formally incorrect and officially discouraged (Pawlowicz 2013; see also fig. 1), but is still widespread in the literature.
Sapropel: A dark, organically rich sediment formed when oxygen levels in bottom waters are very low, e.g. as in ocean anoxic events. For a review see Rohling, Marino, and Grant (2015).
Scleractinian corals: These are also known as stony or hard corals, in which the individual animals or polyps (phylum Cnidaria) build calcium carbonate skeletons by active precipitation of calcium and carbonate ions from the water. Individual skeletons are called corallites. Many scleractinian corals live colonially and build reefs; these are the hermatypic corals, which are of greatest interest to climate scientists. All modern scleractinian coral skeletons consist of aragonite, which is harder but chemically less stable than calcite. Scleractinian corals first appear in the fossil record in the Middle Triassic.
Test: The exterior shell produced by a spherical or nearly spherical marine organism. The organisms which produce tests include sea urchins and many microorganisms including foraminifera and radiolarians.
Younger Dryas: A cold interval in the Northern Hemisphere during deglaciation after the LGM (Last Glacial Maximum); in conventional terms the Younger Dryas is dated as taking place between ~12.9 and 11.5 ka BP (Carlson et al. 2007), while the LGM is dated at ~21 ka BP (Mix, Bard, and Schneider 2001). The Younger Dryas is thought to have resulted from a change in the North Atlantic Ocean circulation (Carlson et al. 2007).
Appendix: Definition and Calibration of Temperature Proxies
Alkenone unsaturation index
Brassell et al. (1986) introduce the alkenone unsaturation index UK37, as
where C37:2 is the relative abundance of the molecule (C37H72O) with two carbon–carbon double bonds, C37:3 the abundance of the molecule (C37H70O) with three double bonds, and so on (Eglinton and Eglinton 2008). In many cases there are very few C37 molecules with four double bonds, so that to a good approximation C37:4=0 and equation (A1) reduces to
where UK'37 is the index most often used in subsequent literature. The SST calibration given by Prahl and Wakeham (1987) for the index defined in equation (A2) is
over the temperature range 11–28°C. This correlation refers specifically to the surface mixed layer. It is based on measurements on samples from a range of geographical locations. The correlation coefficient is close to unity, r=0.997. Brassell et al (1986) refer to sediments reaching down to 550kaBP in the uniformitarian timescale, while Prahl and Wakeham’s (1987) analysis is only cited for sediments down to 18kaBP. In a later calibration study covering the range 0–29°C, Müller et al. (1998) found a closely similar correlation, viz. UK'37=0.033*T+0.044 (r2=0.958) for samples from a total of 370 sites between 60°S and 60°N in both Atlantic and Pacific oceans.
More sophisticated calibrations for present-day conditions are given by Conte et al. (2006). Using ocean surface C37 alkenone unsaturation measurements from a wide range of locations (n=629 original data points reduced to 567, temperature range –1 to 30°C), they derive a single polynomial "global" surface water calibration of UK'37 which predicts alkenone production temperatures over the diversity of modern-day ocean environments and alkenone synthesizing populations:
Here the coefficient of determination r2=0.97, and the “mean standard error” of the estimate is 1.2°C. Two exceptions were found in sediments from the western Argentine Basin, which appeared to have been affected by lateral advection (transport), and, in contrast with previous measurements, from the upwelling region near Cape Verde. In both cases measured temperatures fell several degrees below values predicted by (A4). These exceptions highlight advection as a source of uncertainty. Thus ocean currents may have transported and deposited the measured alkenones an unknown, possibly long distance from the region where they were synthesized such that inferred temperatures are not representative of where they are found.
Conte et al. (2006) also derive a calibration for UK'37 in surface (core-top) sediments against annual mean SST (denoted AnnO) in terms of a linear model (temperature in °C):
Here r2=0.97, n=592 and the mean standard error of the estimate is quoted as 1.1°C. Conte et al. (2006) note that UK'37 in surface sediments is consistently higher than that predicted from AnnO and the surface water temperature calibration. The magnitude of this offset increases as the surface water AnnO decreases; Conte et al. (2006) suggest that it may be due to (1) seasonality in production and/or thermocline production, (2) differential degradation of 37:3 and 37:2 alkenones, or some combination of these effects.
TEX86
This index, originally defined by Schouten et al. (2002), is written more conveniently in the form
following Tierney (2014). The terms [GDGT–1], [GDGT–2], and [GDGT–3] refer to the proportions of core isoGDGT structures containing 1, 2, and 3 cyclopentane rings respectively, and [cren′] to the proportion of Crenarchaeol regioisomer, which contains 4 cyclopentane rings and 1 cyclohexane ring. The linear SST correlation deduced by Schouten et al. (2002) for temperatures in the range 1–30°C is
with r2=0.92; T is the annual mean SST in °C. On the basis of extended data sets Liu et al. (2009a) and Kim et al. (2010) have developed SST calibrations in terms of variants of TEX86. Liu et al. (2009a) represent the SST calibration for five high-latitude sites in reciprocal form, viz.
(range –3 to 30°C, r2=0.82, n=287) while Kim et al. (2010) define two distinct alternatives, viz. TEXL86 for annual mean SST in the range –3 to 30°C in subpolar oceans,
and TEXH86 for warmer oceans where SST>15°C
where TEX86 is as defined in equation (A6). The calibrations given by Kim et al. (2010) are:
(range –3 to 30°C, r2=0.86, n=396) and
(range 5 to 30°C, r2=0.87, n=255). Since TEX86 and its variants are treated as independent variables in these calibrations, it is possible to estimate the root mean square (RMS) predictive uncertainty associated with each, which Tierney (2014) gives as ±3.7°C in equation (A8), ±4°C in (A11) and ±2.5°C in (A12). There are other versions of TEX86 SST calibrations in the literature, some of them relating to lacustrine rather than marine environments.
The Ring Index (RI) introduced by Zhang, Pagani, and Wang (2016) is defined by
Zhang, Pagani, and Wang (2016) point out that although GDGT–0 has a weight of 0 here, it does contribute to a sample’s Ring Index (RI) value via its impact on the relative abundance of the cyclized GDGTs since by definition the sum of all GDGTs, including GDGT–0, is unity. The global RI-TEX86 correlation cited by Zhang, Pagani, and Wang (2016) is
with r2=0.87, n=531 and a 2σ uncertainty margin of ±0.30. This correlation includes data from Red Sea samples even though the TEX86-SST relationship in these samples deviates significantly from the acknowledged global SST calibration.
LDI
The LDI (long diol index) as defined by Rampen et al. (2012) may be written in the form
(Rodrigo-Gámiz et al. 2014) where the expressions in brackets denote the fractional abundances of the temperature-sensitive diols in the sediment samples (see Nomenclature for definition of the diol naming convention). The SST calibration derived by Rampen et al. (2012) is
for which r2=0.969 and the number of points n=162. They report a residual error of <5.4°C and a mean standard error of 2.0°C with respect to WOA (World Ocean Database) temperatures. Rodrigo-Gámiz et al. (2014) report a mean standard deviation of 0.02 in LDI for their samples, equivalent to a 1σ uncertainty in T of ±0.5°C.












